Mathematics
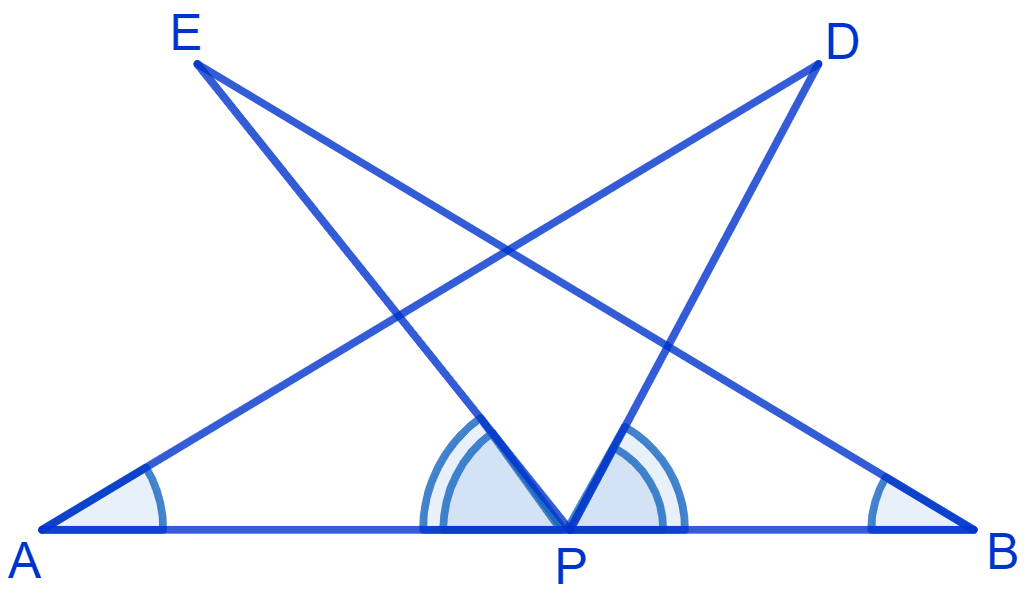
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB. Show that
(i) Δ DAP ≅ Δ EBP
(ii) AD = BE
Answer
Given :
P is the mid-point of AB.
∴ AP = BP ……(1)
Given,
⇒ ∠BAD = ∠ABE …..(2)
From figure,
⇒ ∠BAD = ∠PAD and ∠ABE = ∠PBE
Substituting values in equation (2), we get :
⇒ ∠PAD = ∠PBE ……..(3)
(i) Given,
⇒ ∠EPA = ∠DPB ………(4)
Adding ∠DPE to both sides of the above equation,
⇒ ∠EPA + ∠DPE = ∠DPB + ∠DPE
∴ ∠DPA = ∠EPB ……(5)
In Δ DAP and Δ EBP,
⇒ ∠PAD = ∠PBE [From (3)]
⇒ AP = BP [From (1)]
⇒ ∠DPA = ∠EPB [From (5)]
∴ Δ DAP ≅ Δ EBP (By A.S.A. congruence rule)
Hence, proved that Δ DAP ≅ Δ EBP.
(ii) As,
Δ DAP ≅ Δ EBP
We know that,
Corresponding parts of congruent triangles are equal.
∴ AD = BE (By C.P.C.T.)
Hence, proved that AD = BE.
Related Questions
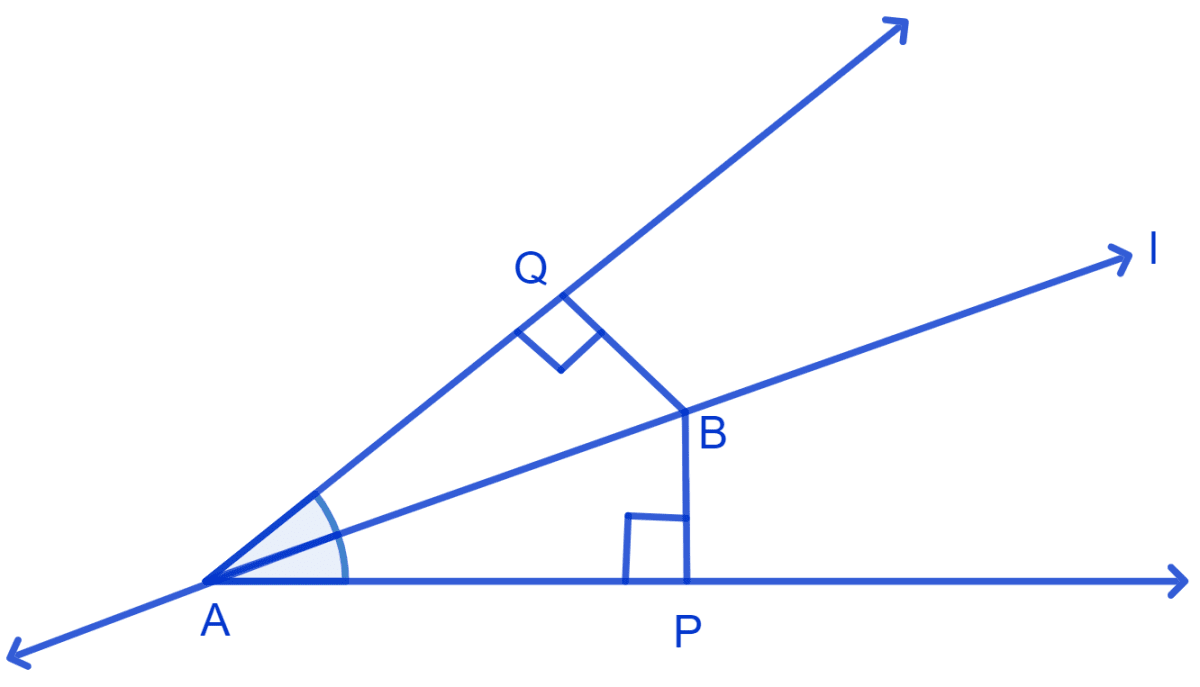
Line l is the bisector of an angle ∠A and B is any point on l. BP and BQ are perpendiculars from B to the arms of ∠A. Show that:
(i) Δ APB ≅ Δ AQB
(ii) BP = BQ or B is equidistant from the arms of ∠A.
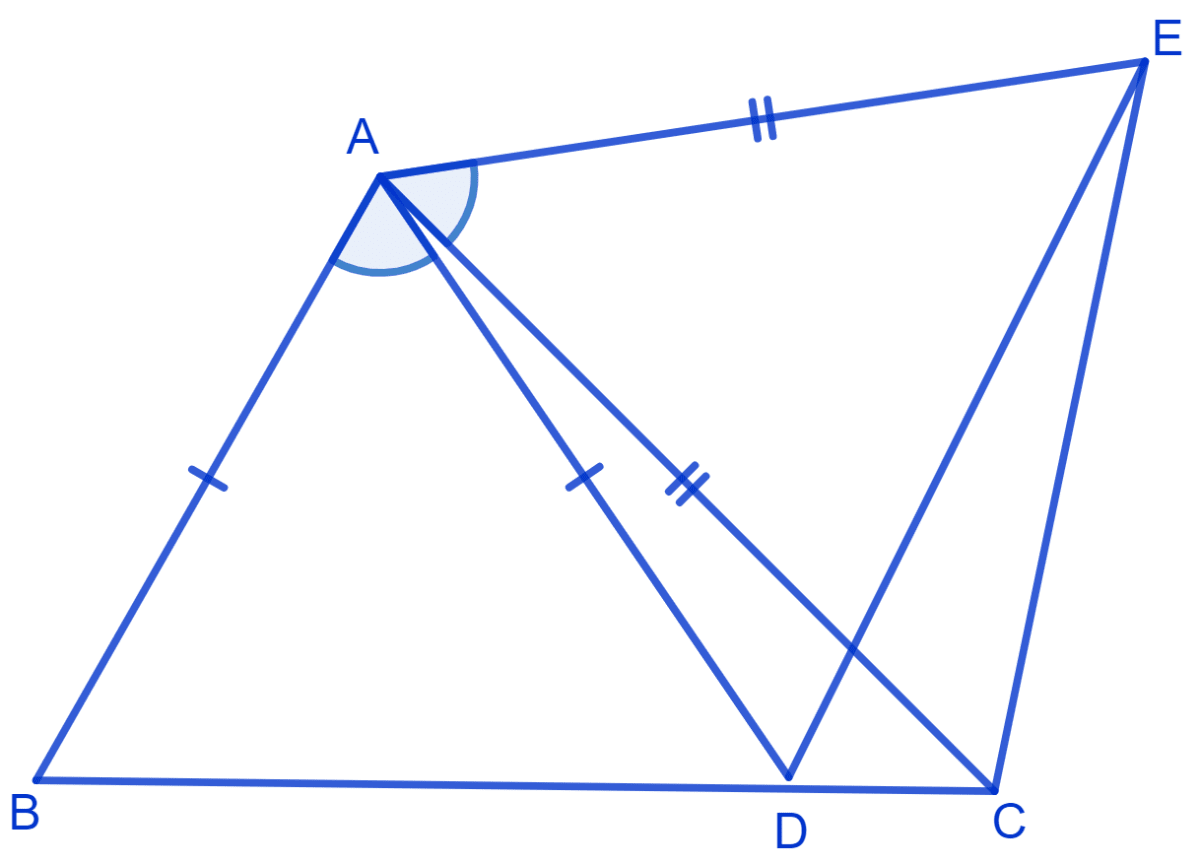
In figure, AC = AE, AB = AD and ∠BAD = ∠EAC. Show that BC = DE.
In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Show that :
(i) OB = OC
(ii) AO bisects ∠A