Mathematics
In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Show that :
(i) OB = OC
(ii) AO bisects ∠A
Triangles
2 Likes
Answer
Given :
AB = AC
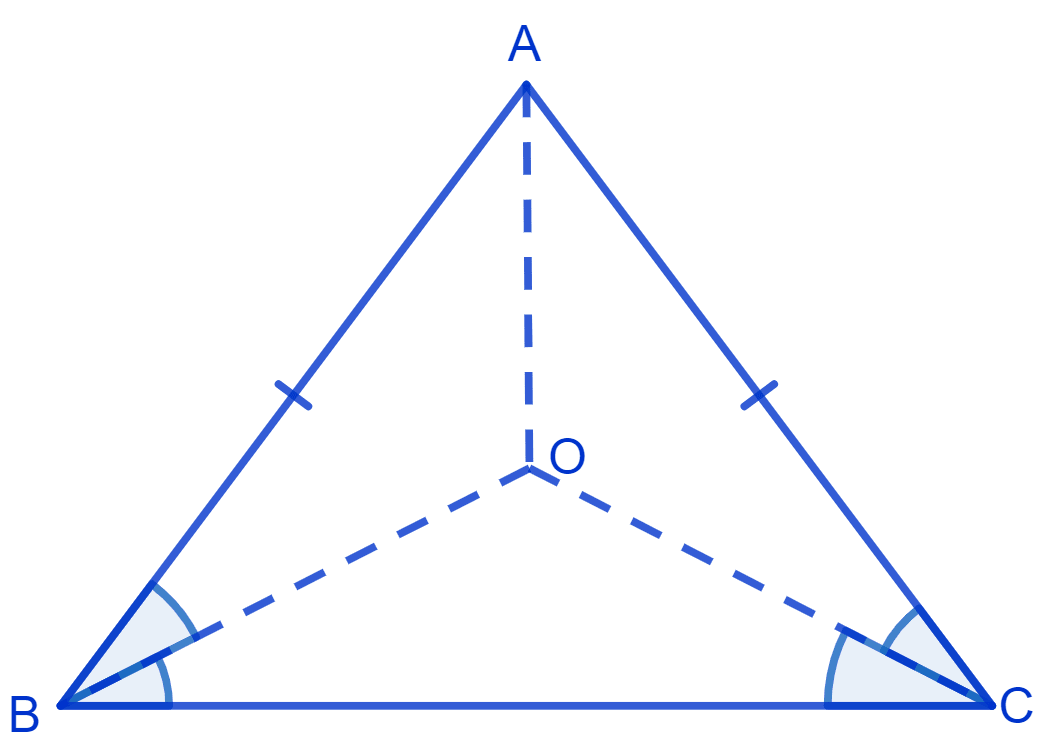
OB is the bisectors of ∠B
⇒ ∠ABO = ∠OBC = .
OC is the bisectors of ∠C
⇒ ∠ACO = ∠OCB = .
(i) It is given that in triangle ABC, AB = AC
⇒ ∠ACB = ∠ABC
Dividing both sides of equation by 2, we get :
⇒ ∠OCB = ∠OBC
We know that,
Sides opposite to equal angles of a triangle are also equal.
⇒ OB = OC.
Hence, proved that OB = OC.
(ii) In Δ OAB and Δ OAC,
⇒ AO = AO (Common)
⇒ OB = OC (Proved above)
⇒ AB = AC (Proved above)
∴ Δ OAB ≅ Δ OAC (By S.S.S. congruence rule)
We know that,
Corresponding parts of congruent triangles are equal.
⇒ ∠BAO = ∠CAO (By C.P.C.T.)
∴ AO bisects ∠A
Hence, proved that AO bisects ∠A.
Answered By
1 Like
Related Questions
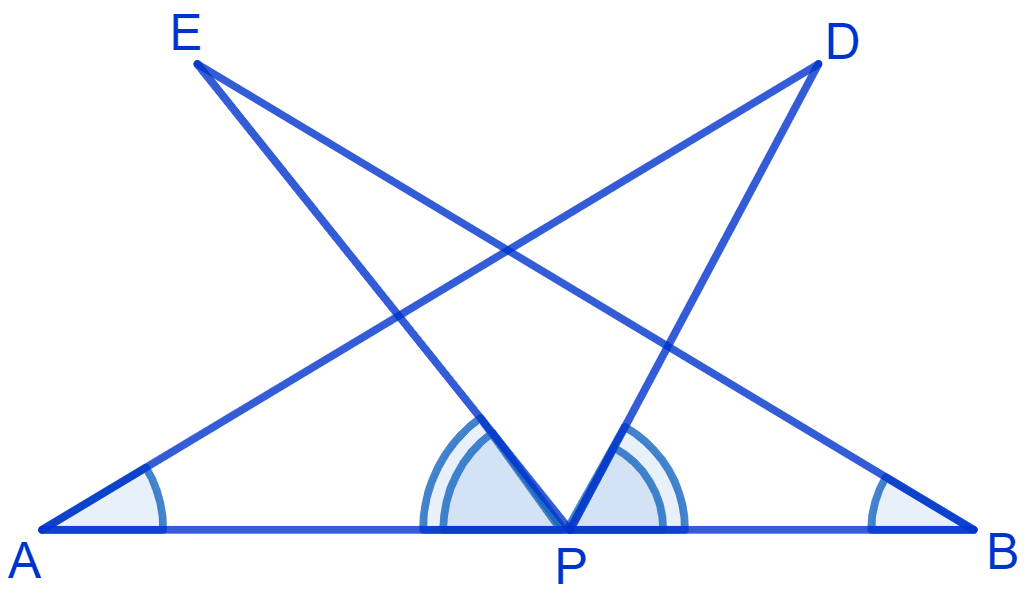
AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB. Show that
(i) Δ DAP ≅ Δ EBP
(ii) AD = BE
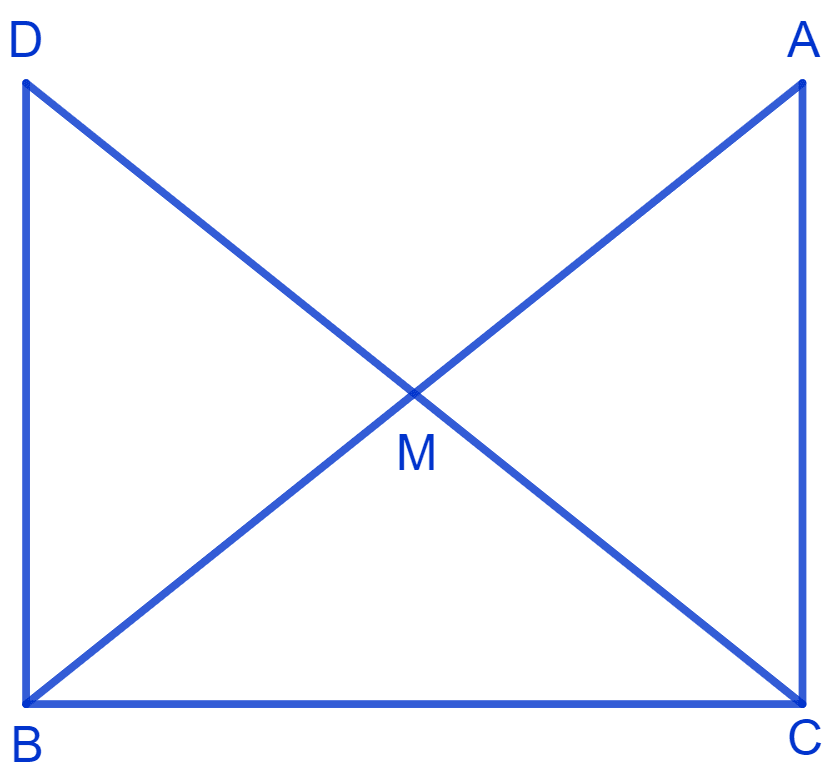
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM. Point D is joined to point B. Show that :
(i) Δ AMC ≅ Δ BMD
(ii) ∠DBC is a right angle
(iii) Δ DBC ≅ Δ ACB
(iv) CM =
In Δ ABC, AD is the perpendicular bisector of BC. Show that Δ ABC is an isosceles triangle in which AB = AC.
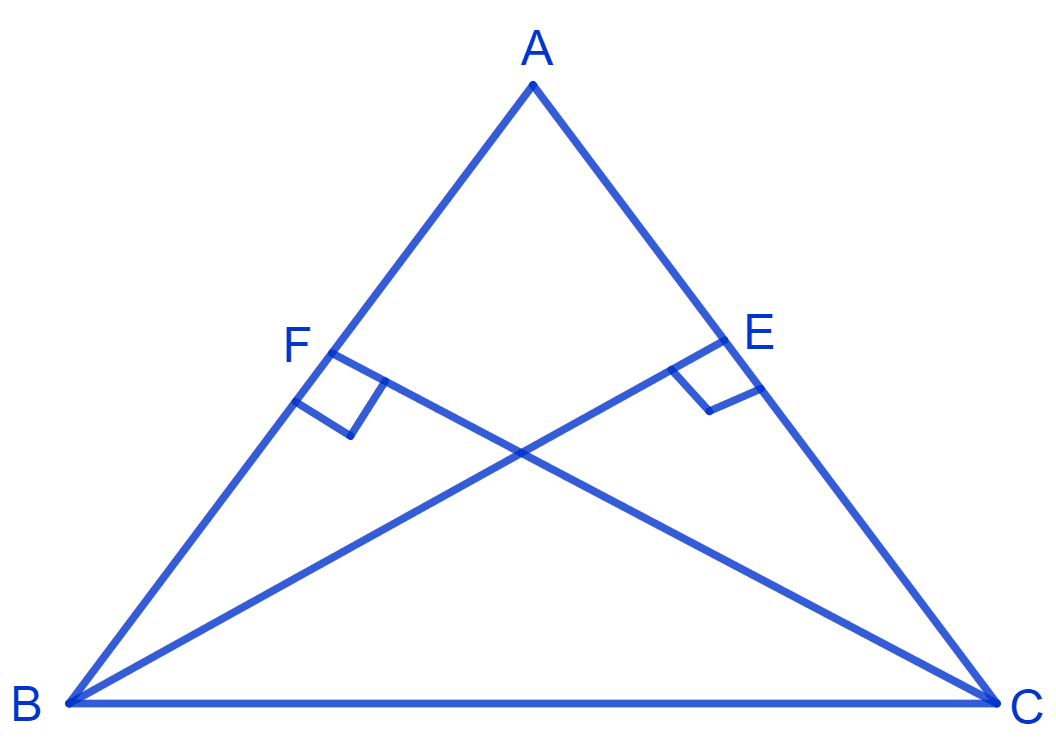
ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively see Fig. Show that these altitudes are equal.