Mathematics
A vertical tower is 20 m high. A man standing at some distance from the tower knows that the cosine of the angle of elevation of the top of the tower is 0.53. How far is he standing from the foot of the tower ?
Heights & Distances
9 Likes
Answer
Let angle of elevation be θ.
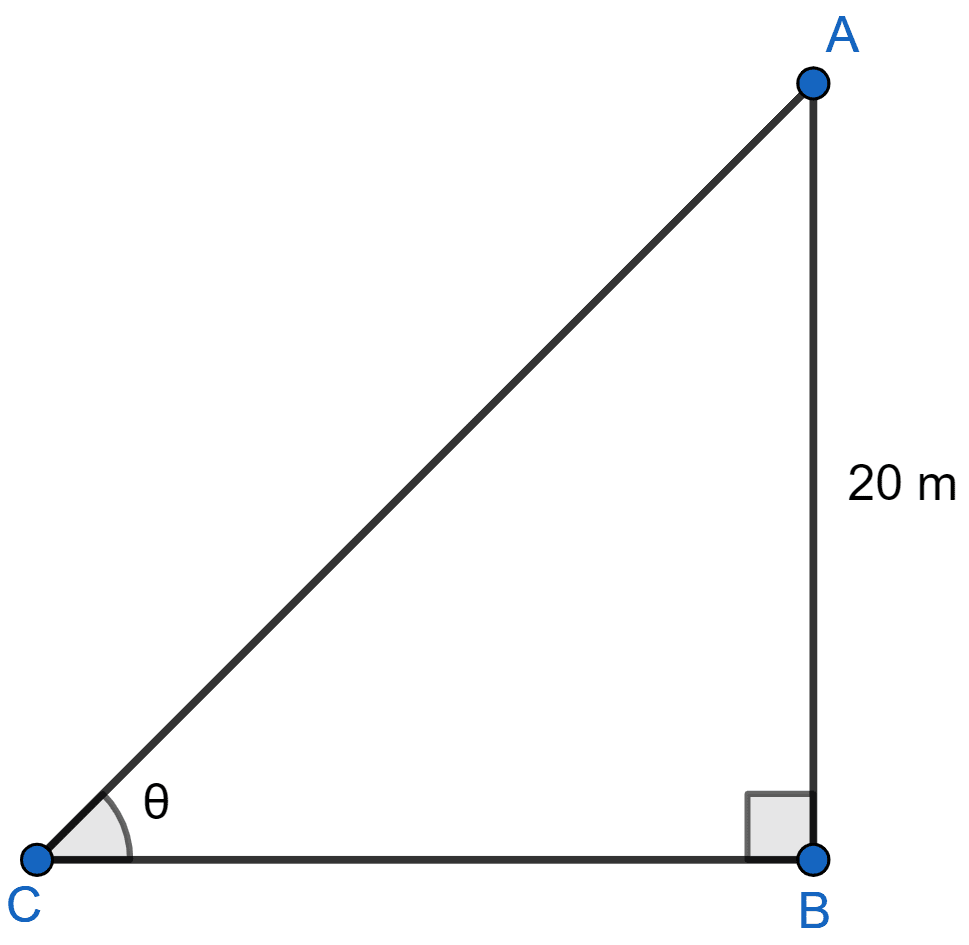
According to question,
⇒ cos θ = 0.53
⇒ cos θ = cos 58°
⇒ θ = 58°.
⇒ cos2 θ = 0.2809
⇒ 1 - sin2 θ = 0.2809
⇒ sin2 θ = 1 - 0.2809
⇒ sin2 θ = 0.7191
⇒ sin θ =
⇒ sin θ = 0.848
⇒ tan θ = = 1.6
The man is standing at a distance of 12.5 meters.
Answered By
3 Likes
Related Questions
With reference to the given figure, a man stands on the ground at point A, which is on the same horizontal plane as B, the foot of the vertical pole BC. The height of the pole is 10 m. The man's eye is 2 m above the ground. He observes the angle of elevation of C, the top of the pole, as x°, where tan x° = . Calculate:
(i) the distance AB in metres;
(ii) angle of elevation of the top of the pole when he is standing 15 metres from the pole. Give your answer to the nearest degree.

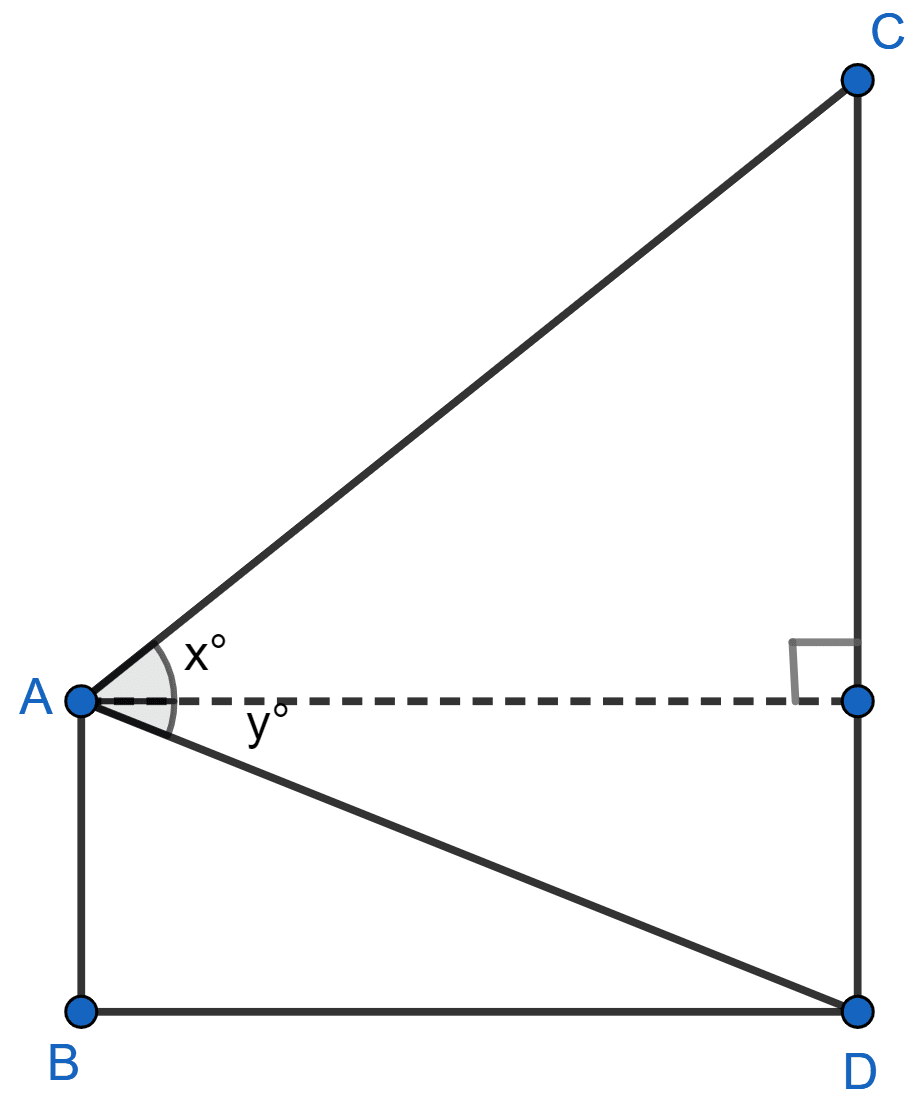
From a window A, 10 m above the ground the angle of elevation of the top C of a tower is x°, where tan x° = and the angle of depression of the foot D of the tower is y°, where tan y° = . Calculate the height CD of the tower in metres.
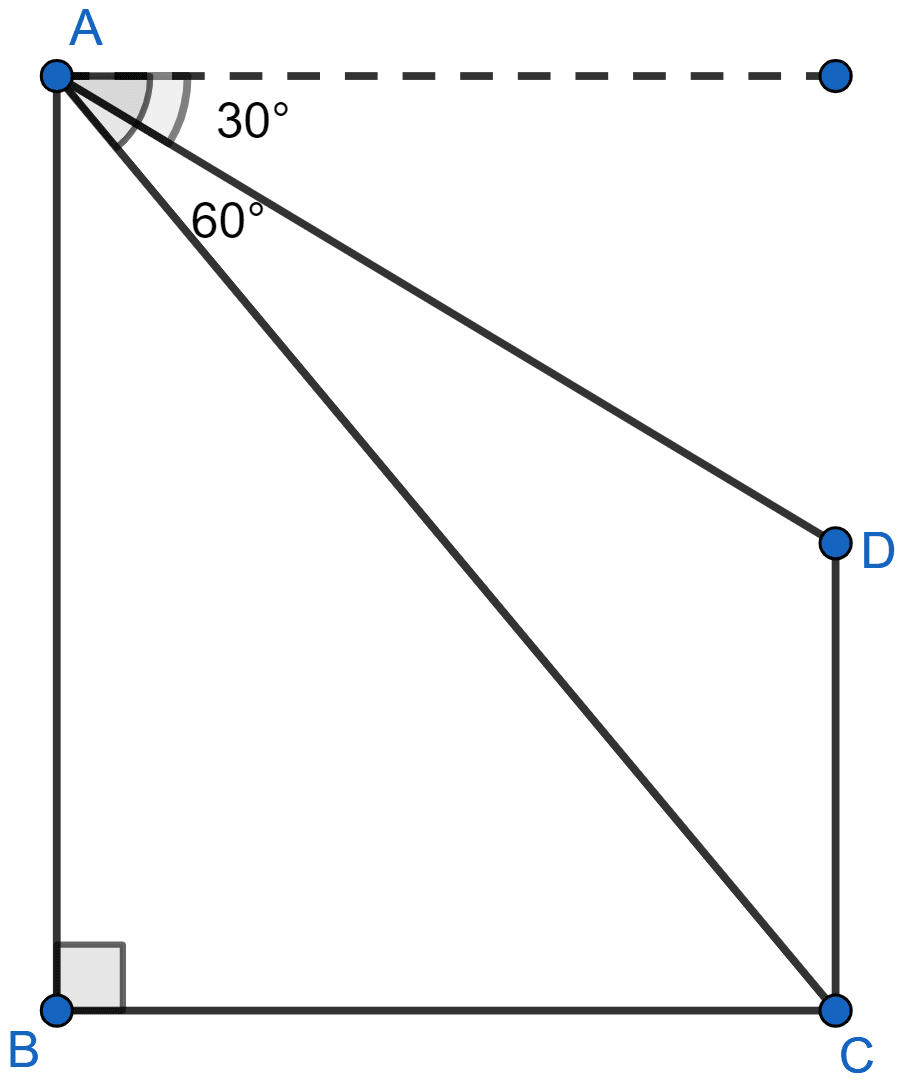
A vertical pole and a vertical tower are on the same level ground in such a way that from the top of the pole the angle of elevation of the top of the tower is 60° and the angle of depression of the bottom of the tower is 30°. Find :
(i) the height of the tower, if the height of the pole is 20 m;
(ii) the height of the pole, if the height of the tower is 75 m
In the given figure, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find :
(i) the horizontal distance between AB and CD.
(ii) the height of the lamp post.