KnowledgeBoat Logo

Mathematics

In the given figure, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find :

(i) the horizontal distance between AB and CD.

(ii) the height of the lamp post.

In the given figure, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find : (i) the horizontal distance between AB and CD. (ii) the height of the lamp post. Heights and Distances, Concise Mathematics Solutions ICSE Class 10.

Heights & Distances

2 Likes

Answer

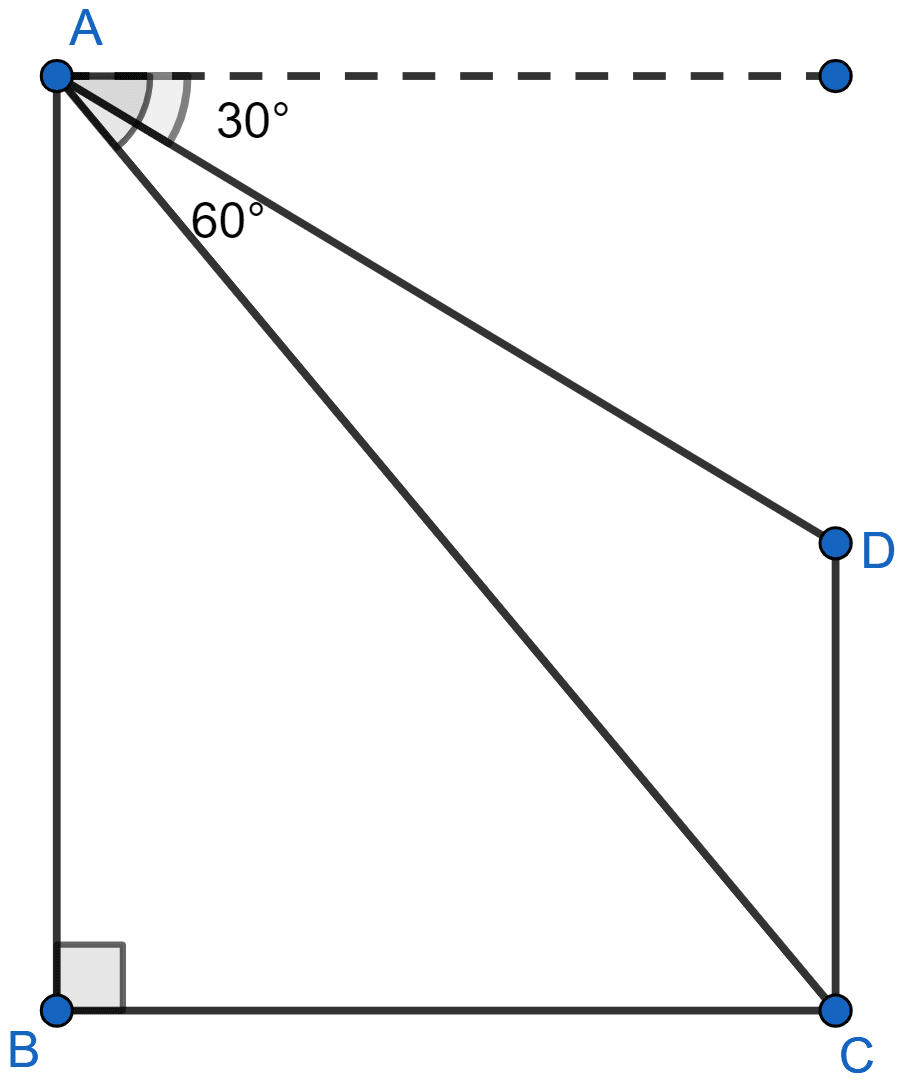
(i) We know that,

Alternate angles are equal.

∴ ∠ACB = ∠EAC = 60°

In the given figure, from the top of a building AB = 60 m high, the angles of depression of the top and bottom of a vertical lamp post CD are observed to be 30° and 60° respectively. Find : (i) the horizontal distance between AB and CD. (ii) the height of the lamp post. Heights and Distances, Concise Mathematics Solutions ICSE Class 10.

In △ABC,

tan 60°=PerpendicularBase⇒3=ABBC⇒BC=AB3=601.732=34.64 m.\text{tan 60°} = \dfrac{\text{Perpendicular}}{\text{Base}} \\[1em] \Rightarrow \sqrt{3} = \dfrac{AB}{BC} \\[1em] \Rightarrow BC = \dfrac{AB}{\sqrt{3}} = \dfrac{60}{1.732} = 34.64 \text{ m}.

Hence, horizontal distance between AB and CD = 34.64 meters.

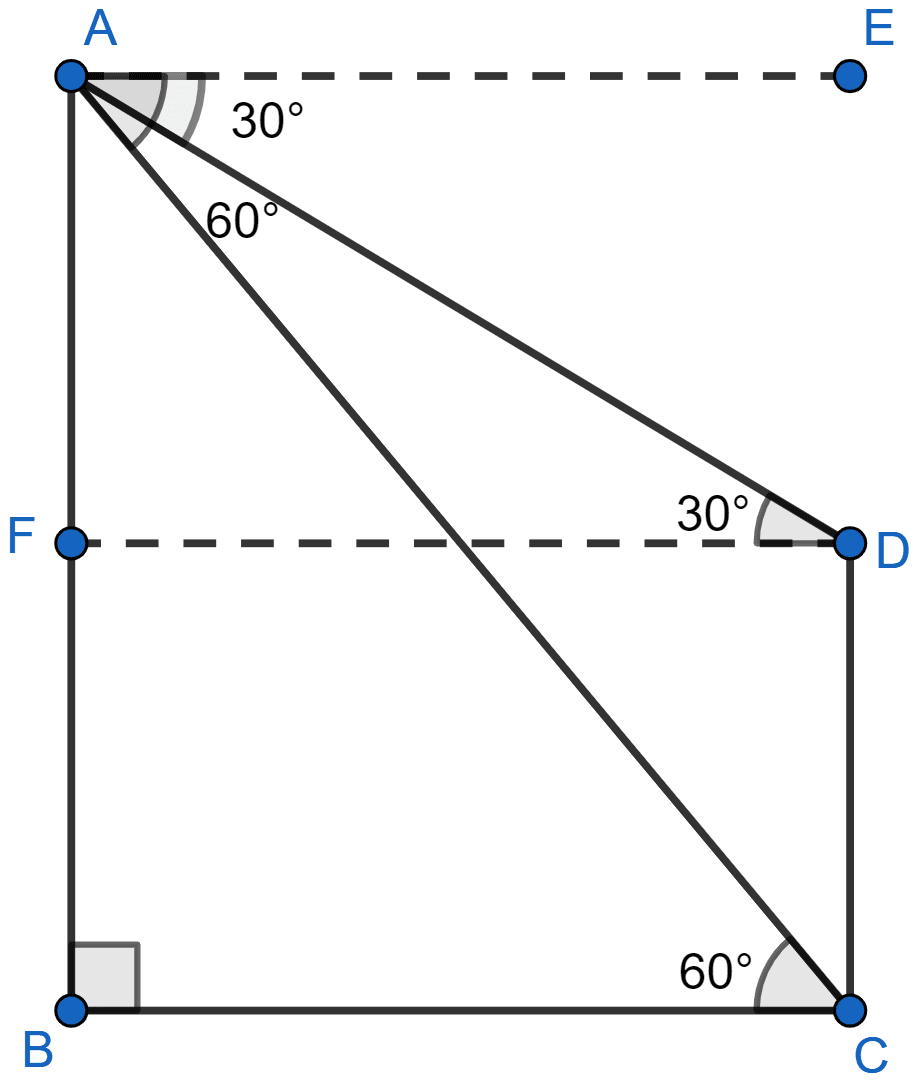
(ii) We know that,

From figure,

FD = BC = 34.64 m

As, alternate angles are equal.

∴ ∠ADF = ∠EAD = 30°

In △AFD,

tan 30°=PerpendicularBase⇒13=AFFD⇒AF=FD3⇒AF=34.641.732=20 m.\text{tan 30°} = \dfrac{\text{Perpendicular}}{\text{Base}} \\[1em] \Rightarrow \dfrac{1}{\sqrt{3}} = \dfrac{AF}{FD} \\[1em] \Rightarrow AF = \dfrac{FD}{\sqrt{3}} \\[1em] \Rightarrow AF = \dfrac{34.64}{1.732} = 20 \text{ m}.

From figure,

BF = AB - AF = 60 - 20 = 40 m.

∴ CD = 40 m.

Hence, height of lamp post = 40 m.

Answered By

1 Like

Related Questions