Mathematics
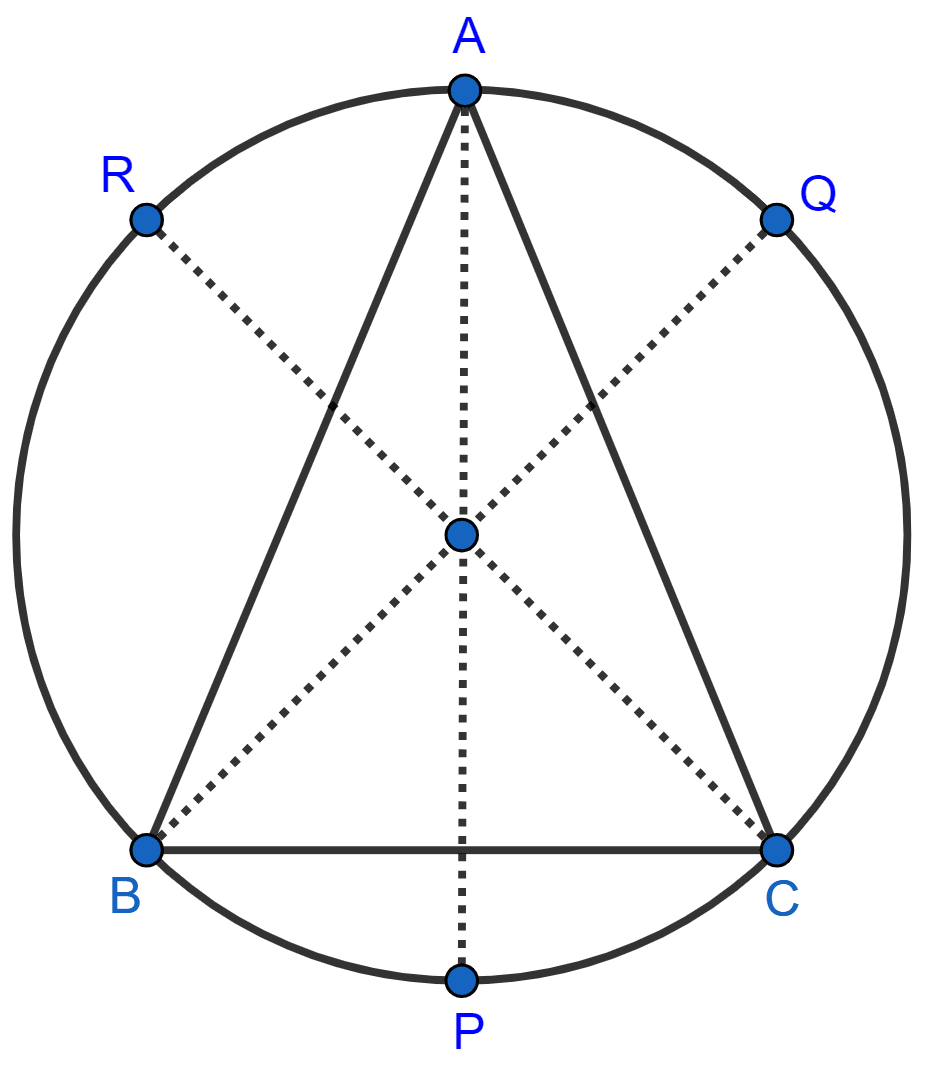
A triangle ABC is inscribed in a circle. The bisectors of angle BAC, ABC and ACB meet the circumcircle of the triangle at points P, Q and R respectively. Prove that :
(i) ∠ABC = 2∠APQ
(ii) ∠ACB = 2∠APR
(iii) ∠QPR = 90° - ∠BAC.
Circles
3 Likes
Answer
Join PQ and PR.
(i) BQ is the bisector of ∠ABC.
∴ ∠ABQ = ∠ABC ………(1)
We know that,
Angles in same segment are equal.
∴ ∠APQ = ∠ABQ …………(2)
From (1) and (2) we get,
⇒ ∠APQ = ∠ABC ………..(3)
⇒ ∠ABC = 2∠APQ.
Hence, proved that ∠ABC = 2∠APQ.
(ii) CR is the bisector of ∠ACB.
∴ ∠ACR = ∠ACB ………(4)
We know that,
Angles in same segment are equal.
∴ ∠ACR = ∠APR …………(5)
From (3) and (4) we get,
⇒ ∠APR = ∠ACB ………..(6)
⇒ ∠ACB = 2∠APR.
Hence, proved that ∠ACB = 2∠APR.
(iii) Adding equations (3) and (6), we get :
⇒ ∠APQ + ∠APR = ∠ABC + ∠ACB
⇒ ∠QPR = (∠ABC + ∠ACB)
⇒ ∠QPR = (180° - ∠BAC)
⇒ ∠QPR = 90° - ∠BAC.
Hence, proved that ∠QPR = 90° - ∠BAC.
Answered By
3 Likes
Related Questions
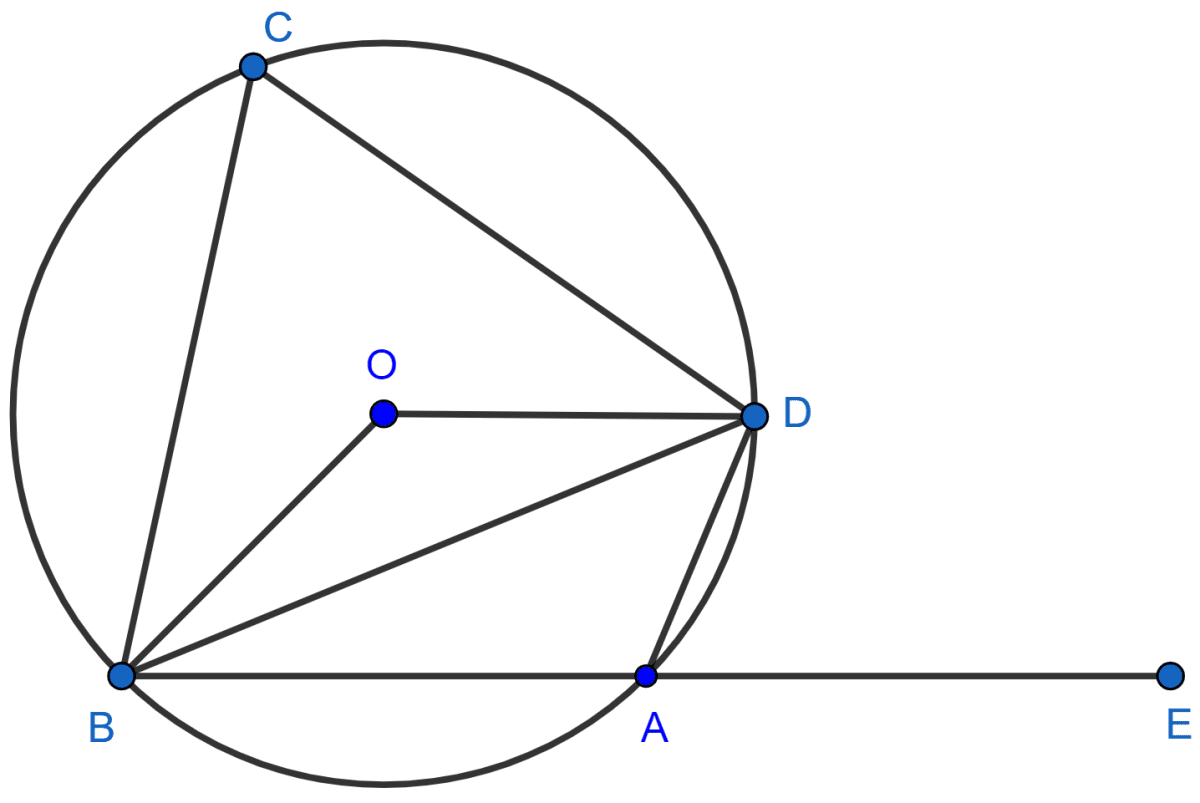
In the figure, given below, CP bisects angle ACB. Show that DP bisects angle ADB.
In the figure, given below, AD = BC, ∠BAC = 30° and ∠CBD = 70°. Find :
(i) ∠BCD
(ii) ∠BCA
(iii) ∠ABC
(iv) ∠ADB

In the given figure, AD is a diameter. O is the centre of the circle. AD is parallel to BC and ∠CBD = 32°. Find :
(i) ∠OBD
(ii) ∠AOB
(iii) ∠BED

In the figure given, O is the centre of the circle. ∠DAE = 70°. Find giving suitable reasons, the measure of
(i) ∠BCD
(ii) ∠BOD
(iii) ∠OBD