KnowledgeBoat Logo

Mathematics

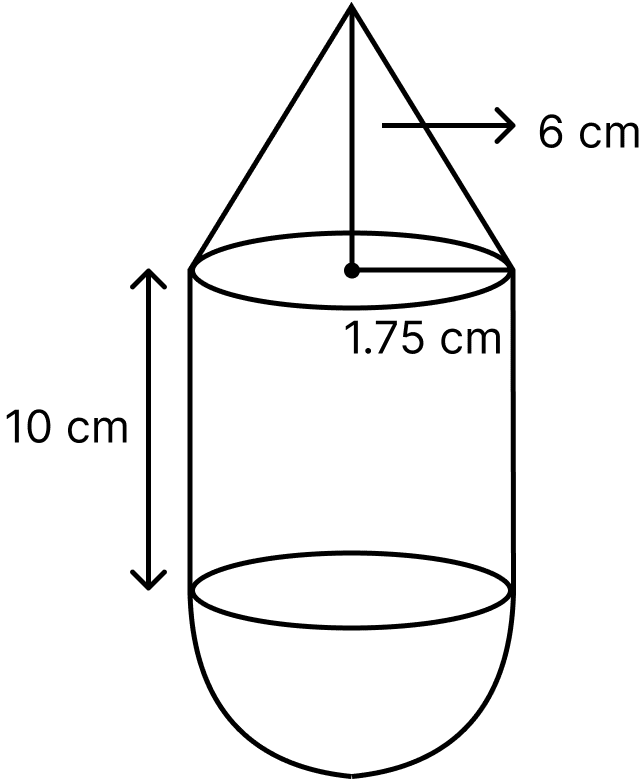
A solid is in the form of a right circular cylinder with a hemisphere at one end and a cone at the other end. Their common diameter is 3.5 cm and the height of the cylindrical and conical portions are 10 cm and 6 cm respectively. Find the volume of the solid. (Take π = 3.14)

Mensuration

26 Likes

Answer

The solid in the form of a right circular cylinder with a hemisphere at one end and a cone at the other end is shown in the figure below:

A solid is in the form of a right circular cylinder with a hemisphere at one end and a cone at the other end. Their common diameter is 3.5 cm and the height of the cylindrical and conical portions are 10 cm and 6 cm respectively. Find the volume of the solid. Mensuration, ML Aggarwal Understanding Mathematics Solutions ICSE Class 10.

Given,

Common Diameter = 3.5 cm,

Common Radius = Diameter2=3.52\dfrac{\text{Diameter}}{2} = \dfrac{3.5}{2} = 1.75 cm.

Height of cylindrical part (h1) = 10 cm.

Height of conical part (h2) = 6 cm.

Volume of solid (V) = Volume of cone + Volume of cylinder + Volume of hemisphere

V=13πr2h2+πr2h1+23πr3=πr2(h23+h1+2r3)=3.14×(1.75)2×(63+10+2×1.753)=3.14×3.0625×(2+10+1.167)=3.14×3.0625×(13.167)=126.617 cm3.V = \dfrac{1}{3}πr^2h2 + πr^2h1 + \dfrac{2}{3}πr^3 \\[1em] = πr^2\Big(\dfrac{h2}{3} + h1 + \dfrac{2r}{3}\Big) \\[1em] = 3.14 \times (1.75)^2 \times \Big(\dfrac{6}{3} + 10 + \dfrac{2 \times 1.75}{3}\Big) \\[1em] = 3.14 \times 3.0625 \times (2 + 10 + 1.167) \\[1em] = 3.14 \times 3.0625 \times (13.167) \\[1em] = 126.617 \text{ cm}^3.

Hence, the volume of the solid is 126.62 cm3.

Answered By

15 Likes

Related Questions