Mathematics
A man 1.8 m high stands at a distance of 3.6 m from a lamp post and casts a shadow of 5.4 m on the ground. Find the height of the lamp post.
Heights & Distances
Answer
Let AB be the lamp post and CD the height of man.
BD is the distance of man from the foot of the lamp and FD is the shadow of man.
CE || DB.
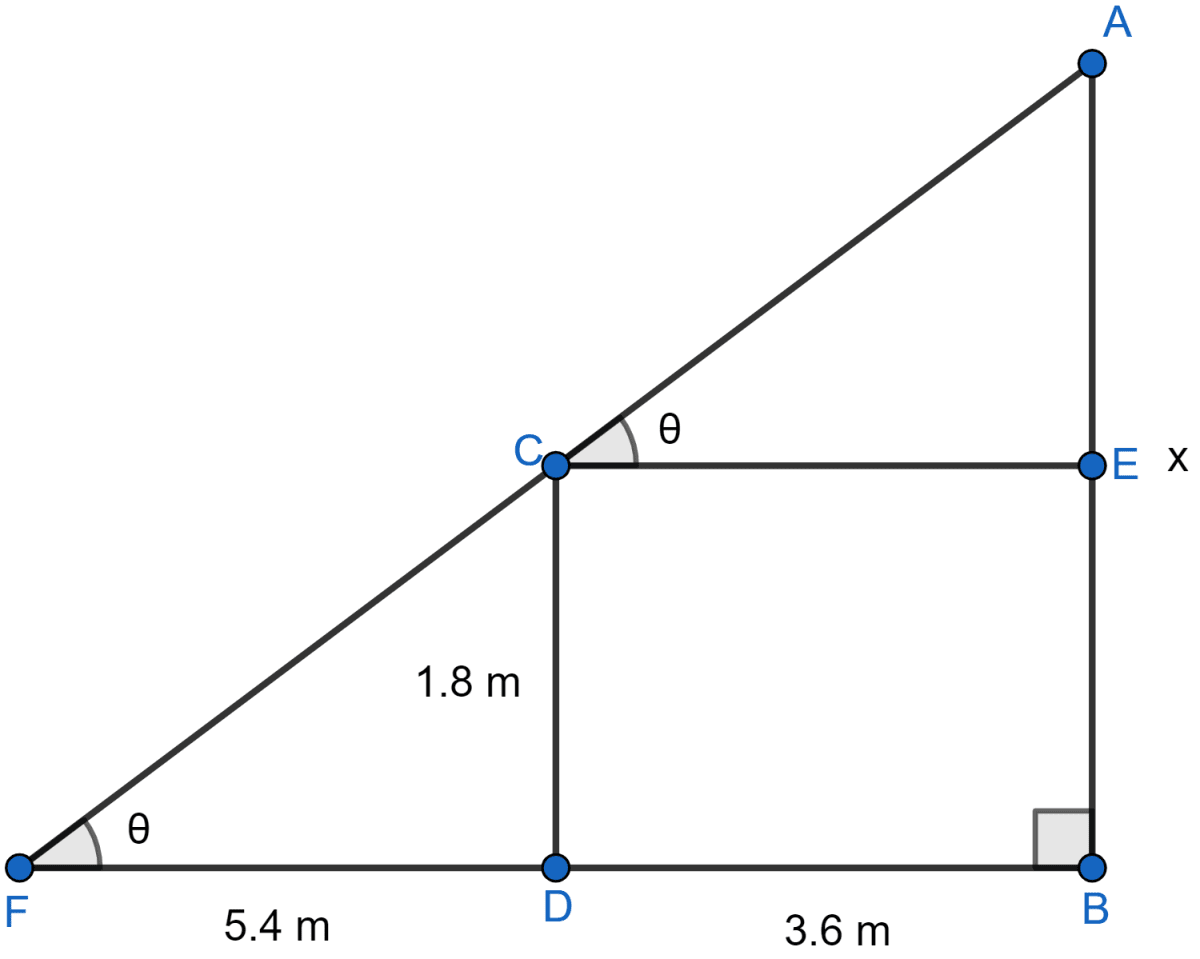
Take AB = x and CD = 1.8 m
EB = CD = 1.8 m
CE = DB = 3.6 m
AE = (x - 1.8) m
Shadow (FD) = 5.4 m
Considering right angled △ACE, we get
Considering right angled △CFD, we get
Comparing Eq 1 and Eq 2 we get,
Hence, the height of the lamp post is 3 meters.
Answered By
Related Questions
An aeroplane at an altitude of 250 m observes the angle of depression of two boats on the opposite banks of a river to be 45° and 60° respectively. Find the width of the river. Write the answer correct to the nearest whole number.
From a tower 126 m high, the angles of depression of two rocks which are in a horizontal line through the base of the tower are 16° and 12° 20'. Find the distance between the rocks if they are on
(i) the same side of the tower
(ii) the opposite sides of the tower.
The angles of depression of the top and the bottom of a 8 m tall building from the top of a multi-storeyed building are 30° and 45° respectively. Find the height of the multi storeyed building and the distance between the two buildings, correct to two decimal places.
A pole of height 5 m is fixed on the top of a tower. The angle of elevation of the top of pole as observed from a point A on the ground is 60° and the angle of depression of the point A from the top of the tower is 45°. Find the height of the tower. (Take )