Mathematics
A largest possible sphere is carved out from a solid wooden cube of side 7 cm. Find :
(i) the volume of sphere
(ii) the percentage of wood wasted in the process.
(Take π = )
Mensuration
4 Likes
Answer
(i) Largest sphere can have diameter = side of cube = 7 cm.
Radius of sphere (r) = = 3.5 cm
By formula,
Hence, volume of sphere = cm3.
(ii) By formula,
Volume of cube = (side)3
= 73
= 343 cm3.
Volume of wood left = Volume of cube - Volume of sphere
Percentage of wood wasted =
Solving,
Hence, percentage of wood wasted = %.
Answered By
2 Likes
Related Questions
If the 3rd and the 9th terms of an arithmetic progression are 4 and -8 respectively. Which term of it is zero ?
A and B are two events such that P(A) = 0.56 and P(B) = 0.64, find :
(i) P(not A)
(ii) P(not B)
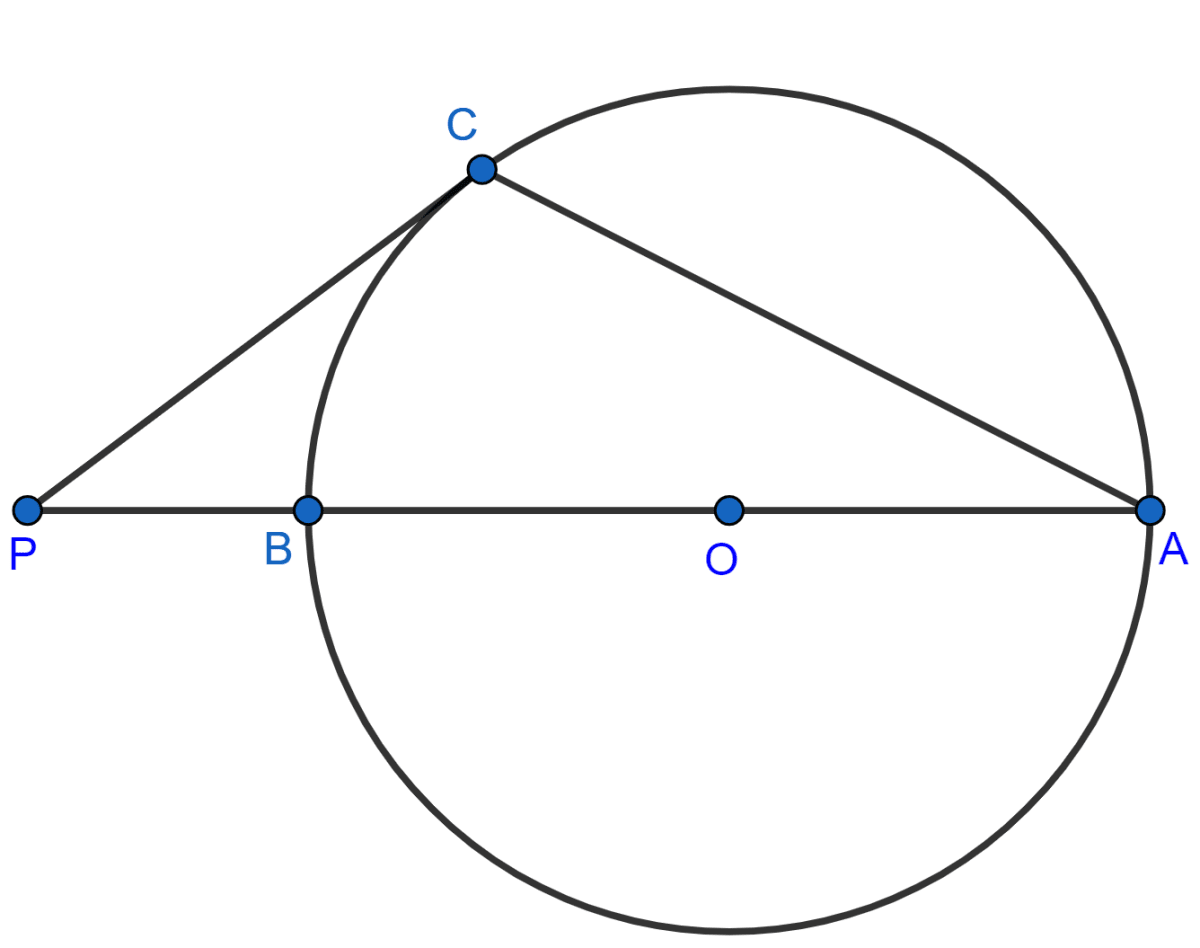
As shown in the figure, the tangent at point C of the circle and diameter AB when produced intersect at point P. If angle PCA = 110°, find the angle CBA.
Calculate the mean, by step-deviation method, for the frequency distribution given below :
Class-interval Frequency 0-5 6 5-10 14 10-15 30 15-20 48 20-25 32 25-30 16 30-35 10 35-40 4