A ladder rests against a wall at an angle α to the horizontal. Its foot is pulled away from the wall through a distance a, so that it slides a distance b down the wall making an angle β with the horizontal. Show that :

$\dfrac{a}{b} = \dfrac{\text{cos α - cos β}}{\text{sin β - sin α}}$

In the figure AB and CD represent the same ladder. So, their length must be equal.

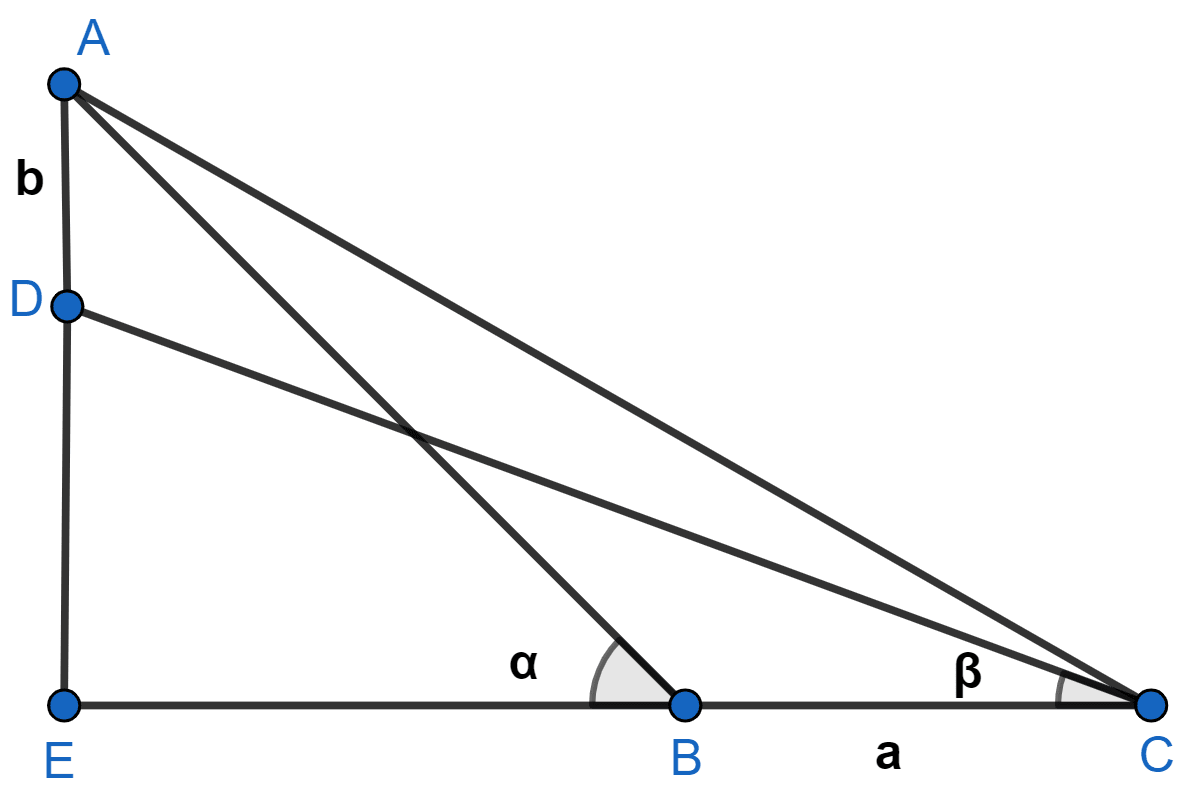
Let length of the ladder be h.

∴ AB = CD = h

In △AEB :

sin α = $\dfrac{AE}{AB}$

AE = AB sin α = h sin α.

In △AEB :

cos α = $\dfrac{BE}{AB}$

BE = AB cos α = h cos α.

In △DEC :

sin β = $\dfrac{DE}{CD}$

DE = CD sin β = h sin β

cos β = $\dfrac{CE}{CD}$

CE = CD cos β = h cos β

From figure,

$\Rightarrow \dfrac{a}{b} = \dfrac{BC}{AD} \\[1em] \Rightarrow \dfrac{a}{b} = \dfrac{CE - BE}{AE - DE} \\[1em] \Rightarrow \dfrac{a}{b} = \dfrac{\text{h cos β} - \text{h cos α}}{\text{h sin α} - \text{h sin β}} \\[1em] \Rightarrow \dfrac{a}{b} = \dfrac{\text{cos β} - \text{cos α}}{\text{sin α} - \text{sin β}}$

Hence, proved that $\dfrac{a}{b} = \dfrac{\text{cos β} - \text{cos α}}{\text{sin α} - \text{sin β}}$.