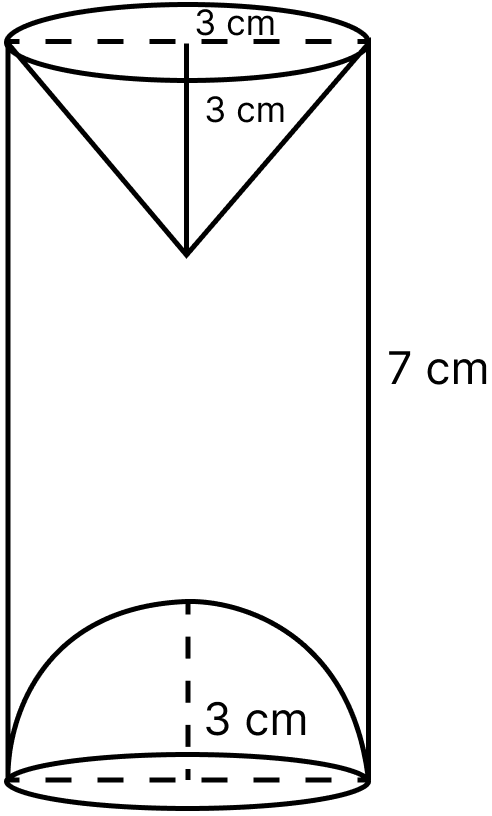
A hemispherical and conical hole are scooped out of a solid wooden cylinder. Find the volume of the remaining solid where the measurements are as follows :

The height of the cylinder is 7 cm, radius of each hemisphere, cone and cylinder is 3 cm. Height of cone is 3 cm. Give your answer correct to nearest whole number. Take π = $\dfrac{22}{7}.$

Given,

Height of cone (h₁) = 3 cm.

Height of cylinder = 7 cm.

From figure,

Volume of remaining solid = Volume of cylinder - Volume of cone - Volume of hemisphere.

∴ Volume of remaining solid = $πr^2h - \dfrac{1}{3}πr^2h_1 - \dfrac{2}{3}πr^3$

$= πr^2\Big(h - \dfrac{h_1}{3} - \dfrac{2r}{3}\Big) \\[1em] = \dfrac{22}{7} \times 3 \times 3 \Big(7 - \dfrac{3}{3} - \dfrac{2 \times 3}{3}\Big) \\[1em] = \dfrac{198}{7} \times \Big(7 - 1 - 2\Big) \\[1em] = \dfrac{198}{7} \times 4 \\[1em] = \dfrac{792}{7} \\[1em] = 113.14 \text{ cm}^3 \approx 113 \text{ cm}^3.$

Hence, the volume of the remaining solid correct to nearest whole number is 113 cm³.