Use ruler and compasses only for this question. Draw a circle of radius 4 cm and mark two chords AB and AC of the circle of length 6 cm and 5 cm respectively.

(i) Construct the locus of points, inside the circle, that are equidistant from A and C. Prove your construction.

(ii) Construct the locus of points, inside the circle, that are equidistant from AB and AC.

Steps of construction :

1. Construct a circle with center as O and radius 4 cm.

2. Take a point A on the circle. From A make arcs of radius 6 cm and 5 cm and where they intersect the circle mark those points as B and C respectively.

(i) We know that locus of points that are equidistant from two points is the perpendicular bisector of line segment joining those points.

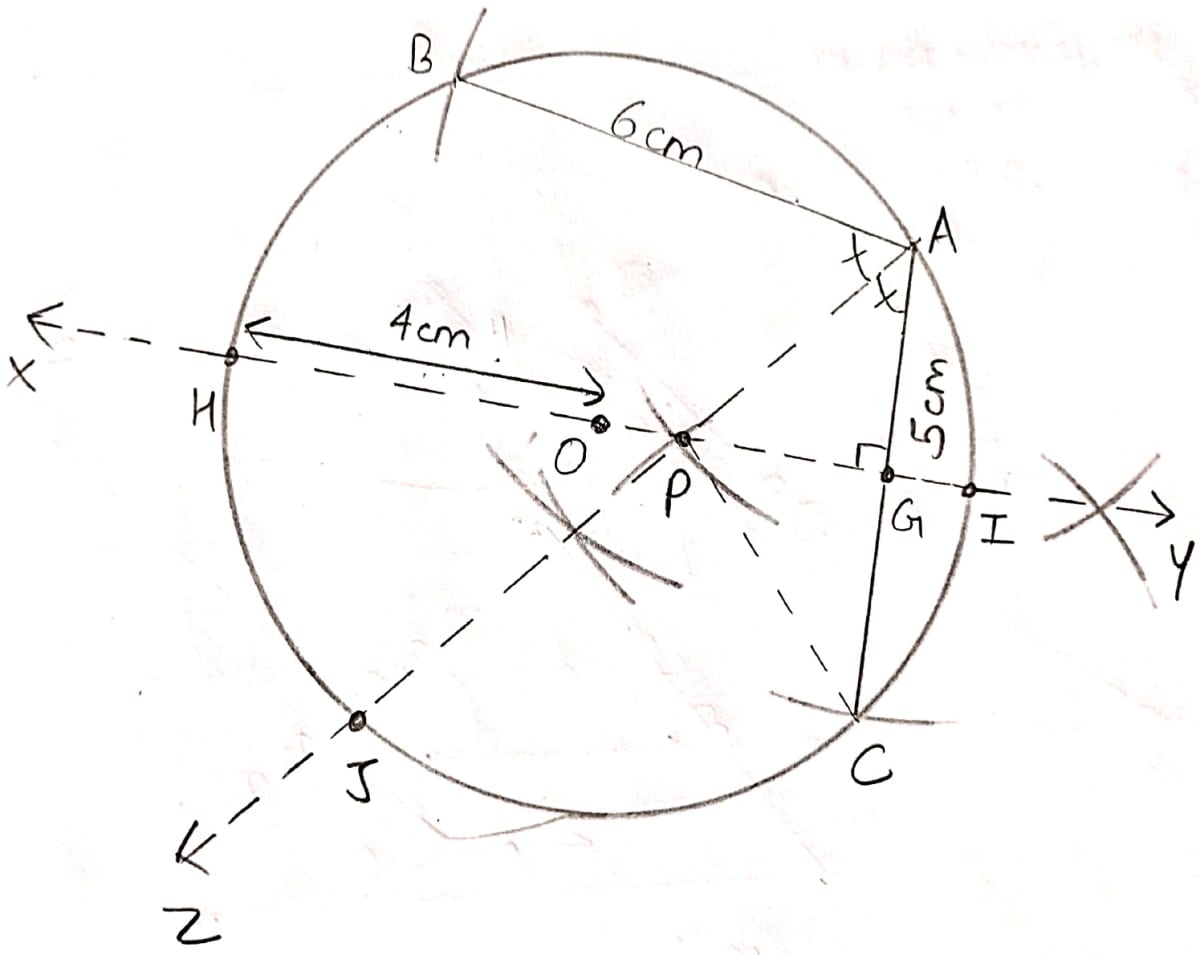
So from figure,

IH is the locus of points inside the circle, that are equidistant from A and C.

Hence, the locus is the diameter of the circle which is perpendicular to the chord AC.

Proof:

Consider △GPA and △GPC.

∠PGC = ∠PGA (Both are equal to 90°)

PG = PG (Common side)

CG = AG (They are equal as GH bisects AC).

Hence, by SAS axiom △GPA ~ △GPC.

Since triangles are similar, hence the ratio of their corresponding sides are equal.

$\therefore \dfrac{AP}{PG} = \dfrac{PC}{PG} \\[1em] \Rightarrow AP = \dfrac{PC}{PG} \times PG \\[1em] \Rightarrow AP = PC.$

Hence, proved that AP = PC.

(ii) We know that locus of points that are equidistant from two lines is the angular bisector of the lines.

So, from figure,

AZ is the angular bisector of angle between AB and AC.

Hence, locus is the chord of the circle bisecting ∠BAC.