Ruler and compasses only may be used in this question. All construction lines and arcs must be clearly shown, and be of sufficient length and clarity to permit the assessment.
(i) Construct a triangle ABC, in which BC = 6 cm, AB = 9 cm, and ∠ABC = 60°.
(ii) Construct the locus of all points, inside △ABC, which are equidistant from B and C.
(iii) Construct the locus of the vertices of the triangles with BC as base, which are equal in area to △ABC.
(iv) Mark the point Q, in your construction, which would make △QBC equal in area to △ABC, and isosceles.
(v) Measure and record the length of CQ.

Question

Ruler and compasses only may be used in this question. All construction lines and arcs must be clearly shown, and be of sufficient length and clarity to permit the assessment.
(i) Construct a triangle ABC, in which BC = 6 cm, AB = 9 cm, and ∠ABC = 60°.
(ii) Construct the locus of all points, inside △ABC, which are equidistant from B and C.
(iii) Construct the locus of the vertices of the triangles with BC as base, which are equal in area to △ABC.
(iv) Mark the point Q, in your construction, which would make △QBC equal in area to △ABC, and isosceles.
(v) Measure and record the length of CQ.

KnowledgeBoat · Accepted Answer

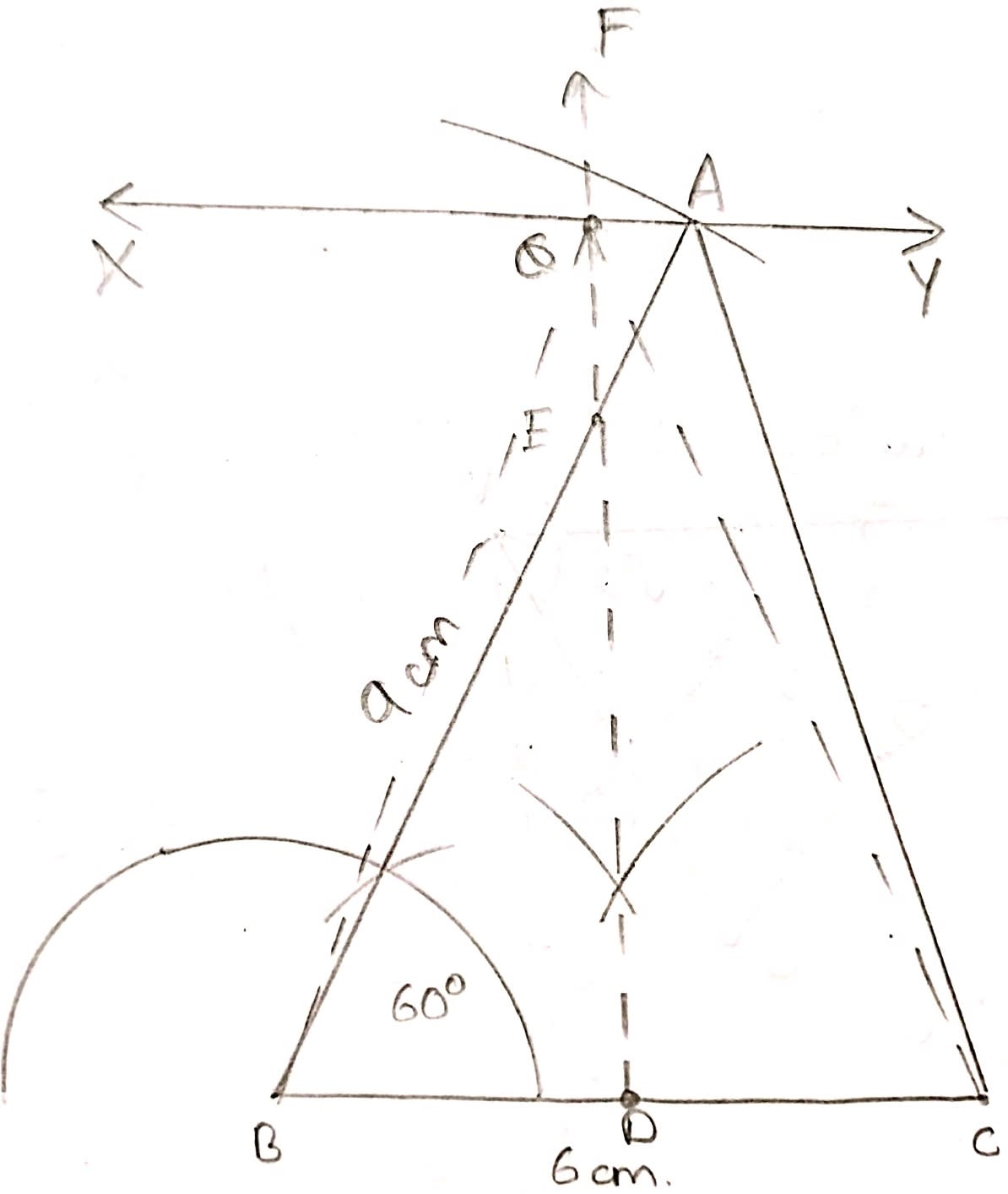
(i) Steps of construction : 1. Draw BC = 6 cm as base. 2. Construct ∠ABC = 60° and make an arc from B such as AB = 9 cm. 3. Join points A, B and C such that ABC forms a triangle. (ii) We know that locus of points equidistant from two points is the perpendicular bisector of the line segment joining two points. From figure, Right bisector of BC (i.e. DE in figure) inside △ABC. (iii) The locus of the vertices of the triangles with BC as base, which are equal in area to △ABC is a straight line through A and parallel to BC, the area will be same as triangles will be on same base and between same parallel lines. (iv) Q is the point of intersection of right bisector of BC and the straight line through A parallel to BC. (v) On measuring, CQ = 8.2 cm approximately.

Mathematics

Locus

Answer

Related Questions

Construct an isosceles triangle ABC such that AB = 6 cm, BC = AC = 4 cm. Bisect ∠C internally and mark a point P on this bisector such that CP = 5 cm. Find the points Q and R which are 5 cm from P and also 5 cm from the line AB.

Using ruler and compass only, construct a semicircle with diameter BC = 7 cm. Locate a point P on the circumference of the semicircle such that A is equidistant from B and C. Completely the cyclic quadrilateral ABCD such that D is equidistant from AB and BC. Measure ∠ADC and write it down.

Using ruler and compasses only, construct a quadrilateral ABCD in which AB = 6 cm, BC = 5 cm, ∠B = 60°, AD = 5 cm and D is equidistant from AB and BC. Measure CD.