Mathematics
Use graph paper for this question.
(i) The point P(2, -4) is reflected about the line x = 0 to get the image Q. Find the coordinates of Q.
(ii) Point Q is reflected about the line y = 0 to get the image R. Find the coordinates of R.
(iii) Name the figure PQR.
(iv) Find the area of the figure PQR.
Reflection
39 Likes
Answer
The graph is given below:
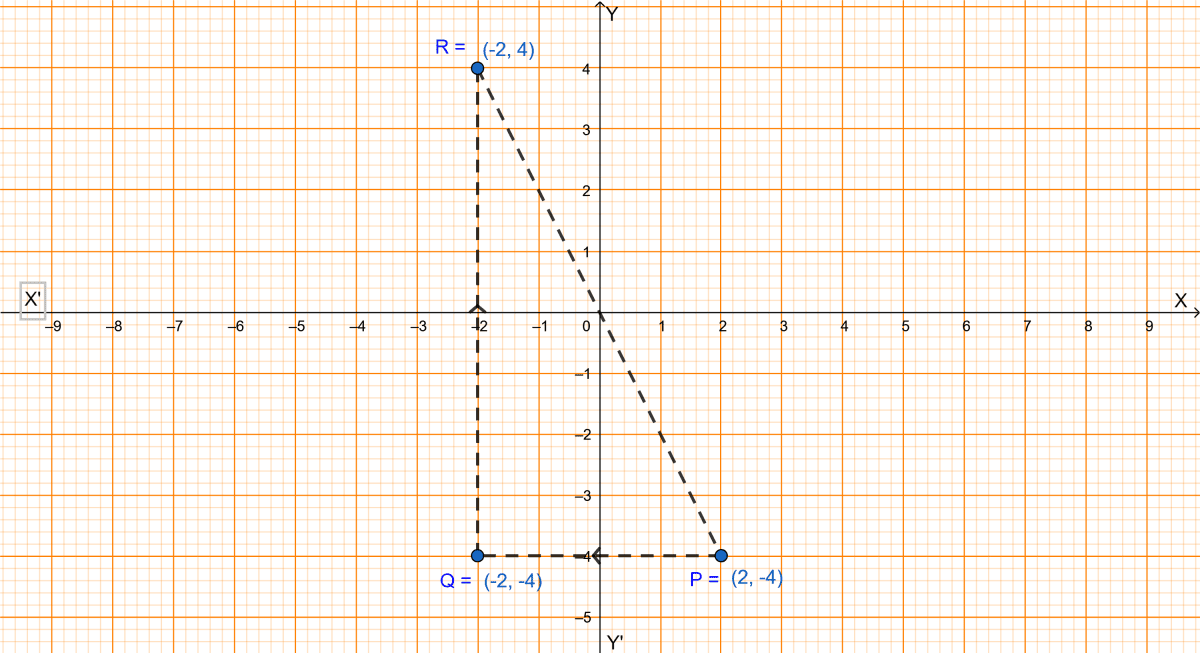
(i) From graph we get,
The coordinate of Q is (-2, -4).
(ii) From graph we get,
The coordinate of R is (-2, 4).
(iii) From graph we get,
The image formed is right angled triangle.
(iv) We know that in the graph,
One block represents 1 unit.
Area of right angle triangle = base × height.
Hence, the area of figure PQR is 16 sq. units.
Answered By
9 Likes
Related Questions
Use graph paper for this question (take 2cm = 1 unit along both x and y axis). ABCD is a quadrilateral whose vertices are A(2, 2), B(2, -2), C(0, -1) and D(0, 1).
(i) Reflect quadrilateral ABCD on the y-axis and name it as A'B'CD.
(ii) Write down the coordinates of A' and B'.
(iii) Name two points which are invariant under the above reflection.
(iv) Name the polygon A'B'CD.
Use a graph sheet for this question. Take 1cm = 1 unit along both x and y-axes.
(i) Plot the points : A(0, 5), B(3, 0), C(1, 0) and D(1, -5).
(ii) Reflect the points B, C and D on the y-axis and name them as B', C' and D' respectively.
(iii) Write down the coordinates of B', C' and D'.
(iv) Join the points A, B, C, D, D', C', B', A in order and give a name to the closed figure ABCDD'C'B'.
Using a graph paper, plot the points A(6, 4) and B(0, 4).
(i) Reflect A and B in the origin to get images A' and B'.
(ii) Write the coordinates of A' and B'.
(iii) State the geometrical name for the figure ABA'B'.
(iv) Find its perimeter.
Use graph paper to solve this question.
(i) Plot the points A(4, 6) and B(1, 2).
(ii) If A' is the image of A when reflected in the x-axis, write the coordinates of A'.
(iii) If B' is the image of B when reflected in the line AA', write the coordinates of B'.
(iv) Give the geometrical name for the figure ABA'B'.