Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord.

Let the common chord be AB, P and Q be the centers of the two circles.

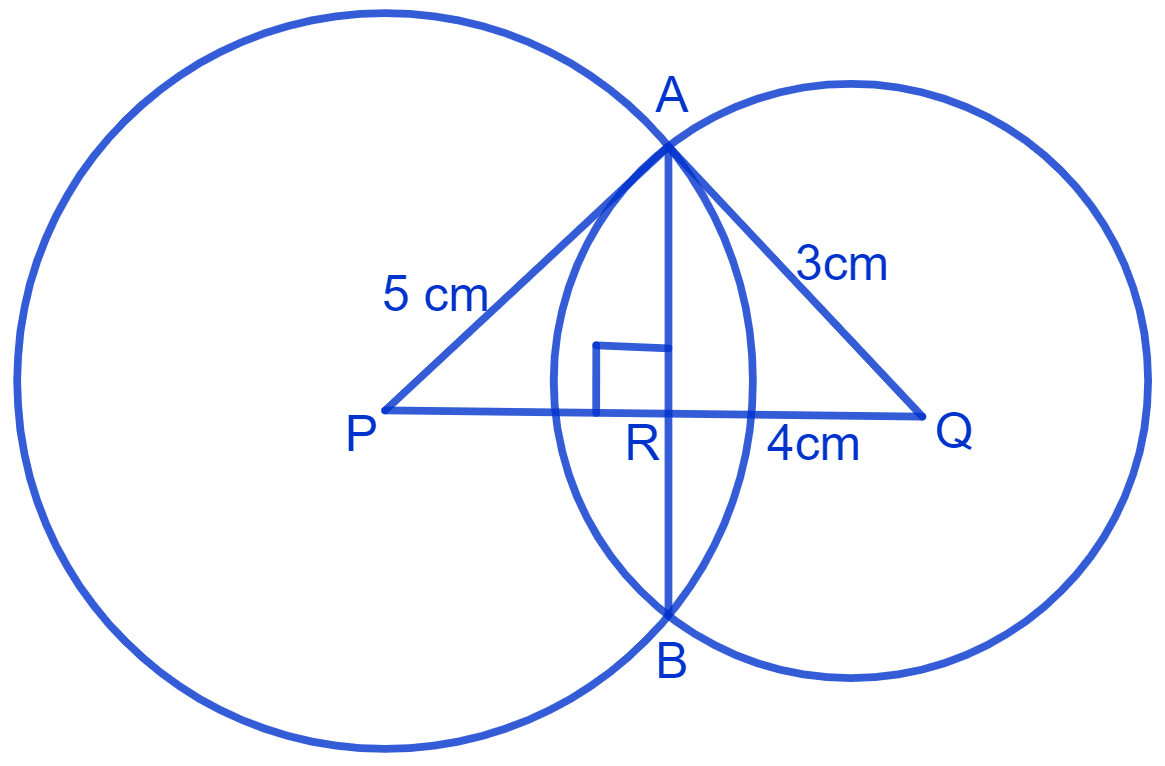
From figure,

⇒ AP = 5 cm and AQ = 3 cm

⇒ PQ = 4 cm (Given)

Join PQ ⊥ AB.

We know that,

Perpendicular from center to the chord, bisects the chord.

⇒ AR = RB = $\dfrac{1}{2}AB$

Let PR = x cm, RQ = (4 - x) cm.

In ∆ ARP,

By pythagoras theorem,

⇒ AP² = AR² + PR²

⇒ AR² = AP² - PR²

⇒ AR² = (5)² - (x)² …….(1)

In ∆ ARQ,

By pythagoras theorem,

⇒ AQ² = AR² + QR²

⇒ AR² = AQ² - QR²

⇒ AR² = 3² - (4 - x)² ……..(2)

From equation (1) and (2), we get :

⇒ (5)² - (x)² = (3)² - (4 - x)²

⇒ 25 - x² = 9 - (16 - 8x + x²)

⇒ 25 - x² = 9 - 16 + 8x - x²

⇒ 25 - x² = 8x - x² - 7

⇒ 25 + 7 - x² + x² = 8x

⇒ 32 = 8x

⇒ x = $\dfrac{32}{8}$

⇒ x = 4.

substituting the value of x in equation (1) we get :

⇒ AR² = 5² - 4²

⇒ AR² = 25 - 16

⇒ AR² = 9

⇒ AR = $\sqrt{9}$

⇒ AR = 3 cm.

As,

⇒ AR = $\dfrac{1}{2}AB$

⇒ AB = 2 x AR = 2 x 3 = 6 cm.

Hence, the length of common chord is 6 cm.