Mathematics
Triangle ABC is similar to triangle PQR. If AD and PM are corresponding medians of the two triangles, prove that: .
Similarity
2 Likes
Answer
Given, ∆ABC ~ ∆PQR
AD and PM are the medians, so BD = DC and QM = MR
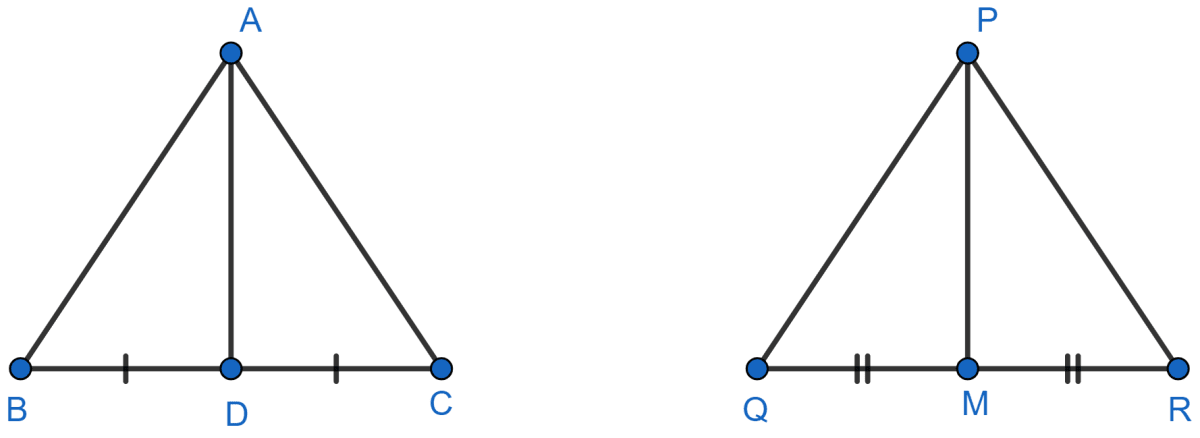
Since, corresponding sides of similar triangles are proportional.
We can write,
And, ∠ABC = ∠PQR i.e., ∠ABD = ∠PQM
∴ ∆ABD ~ ∆PQM [By SAS]
Since, corresponding sides of similar triangles are proportional.
.
Hence, proved that .
Answered By
1 Like
Related Questions
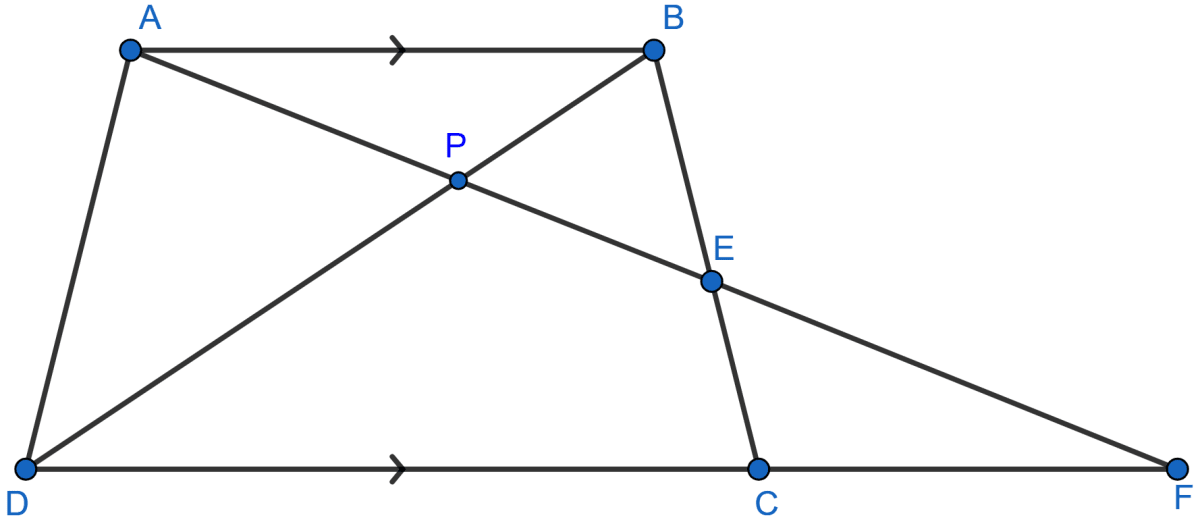
In the following figure, ABCD is a trapezium with AB || DC. If AB = 9 cm, DC = 18 cm, CF = 13.5 cm, AP = 6 cm and BE = 15 cm,
Calculate :
(i) EC (ii) AF (iii) PE
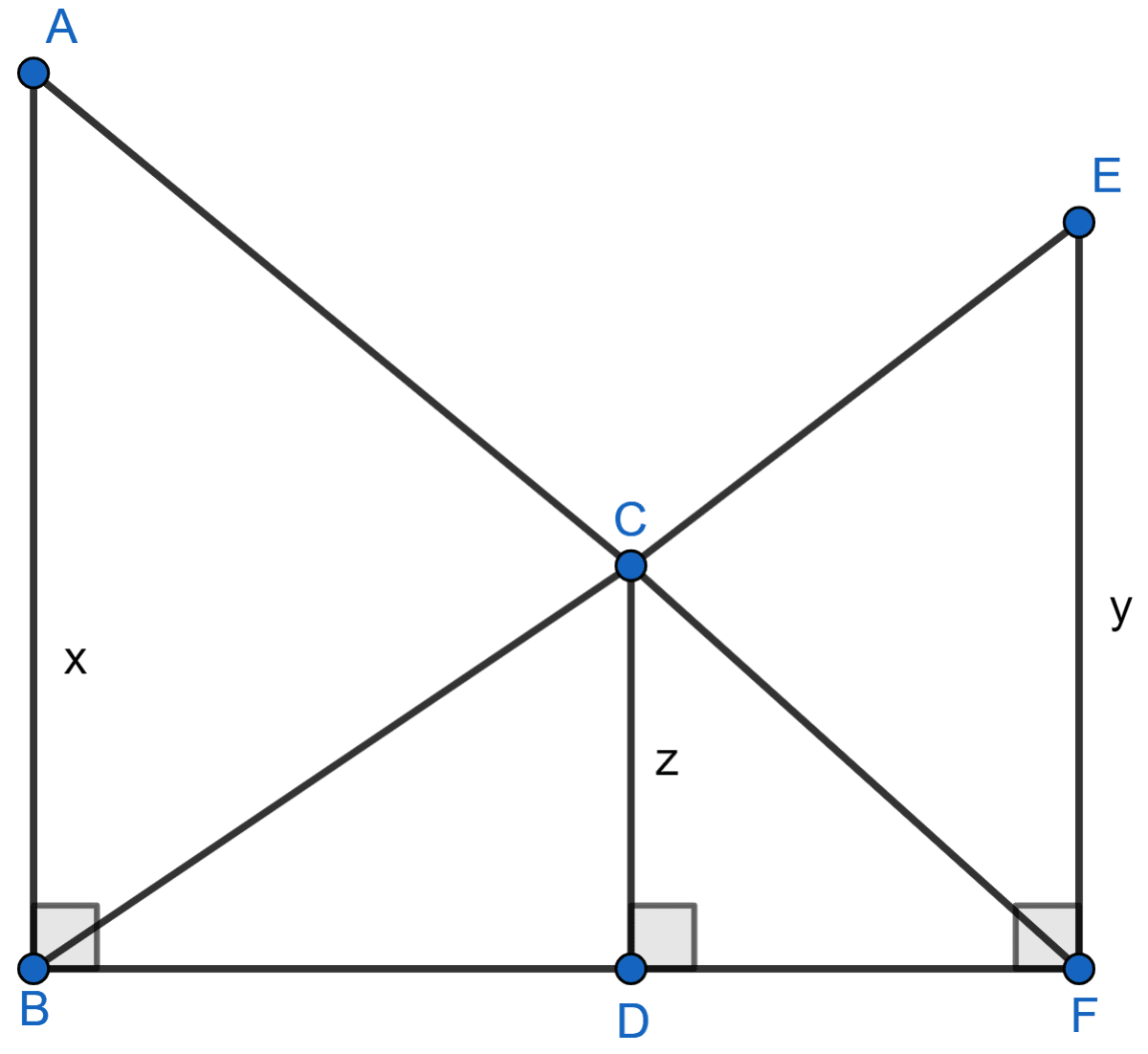
In the following figure, AB, CD and EF are perpendicular to the straight line BDF.
If AB = x and, CD = z unit and EF = y unit, prove that:
Triangle ABC is similar to triangle PQR. If AD and PM are altitudes of the two triangles, prove that: .
Triangle ABC is similar to triangle PQR. If bisector of angle BAC meets BC at point D and bisector of angle QPR meets QR at point M, prove that: .