The velocity-time graph of a moving body is given below in figure

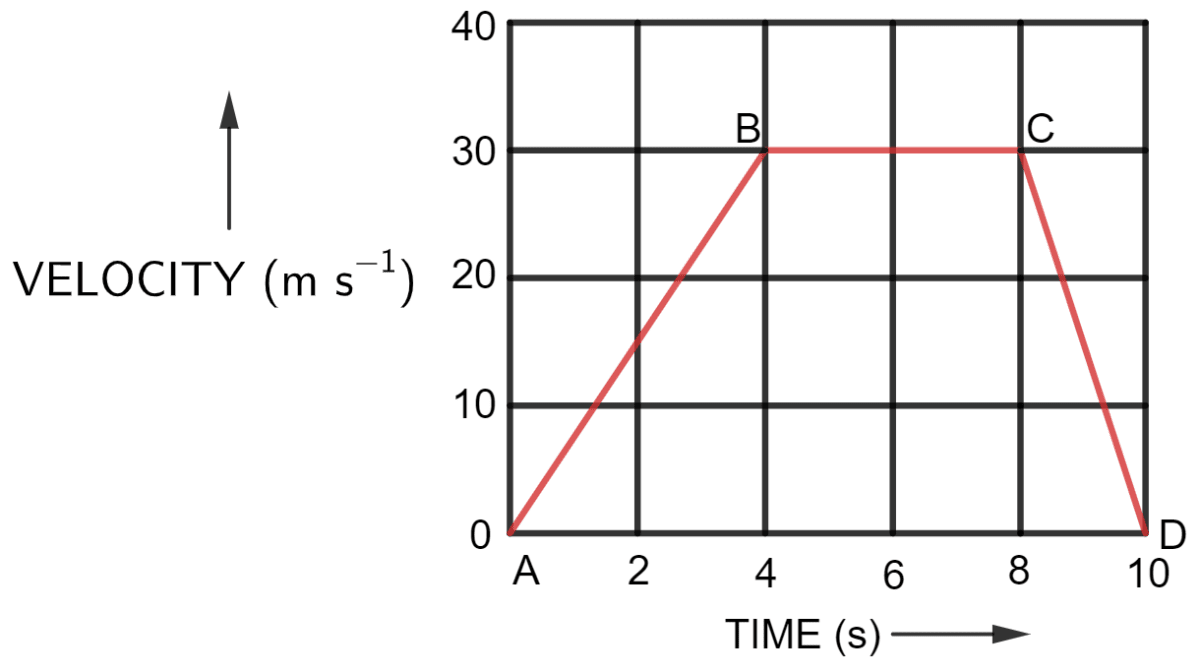
Find —

(i) The acceleration in parts AB, BC and CD.

(ii) Displacement in each part AB, BC, CD, and

(iii) Total displacement.

Let E be the point at t = 4 and F be the point at t = 8 as labelled in the graph below:

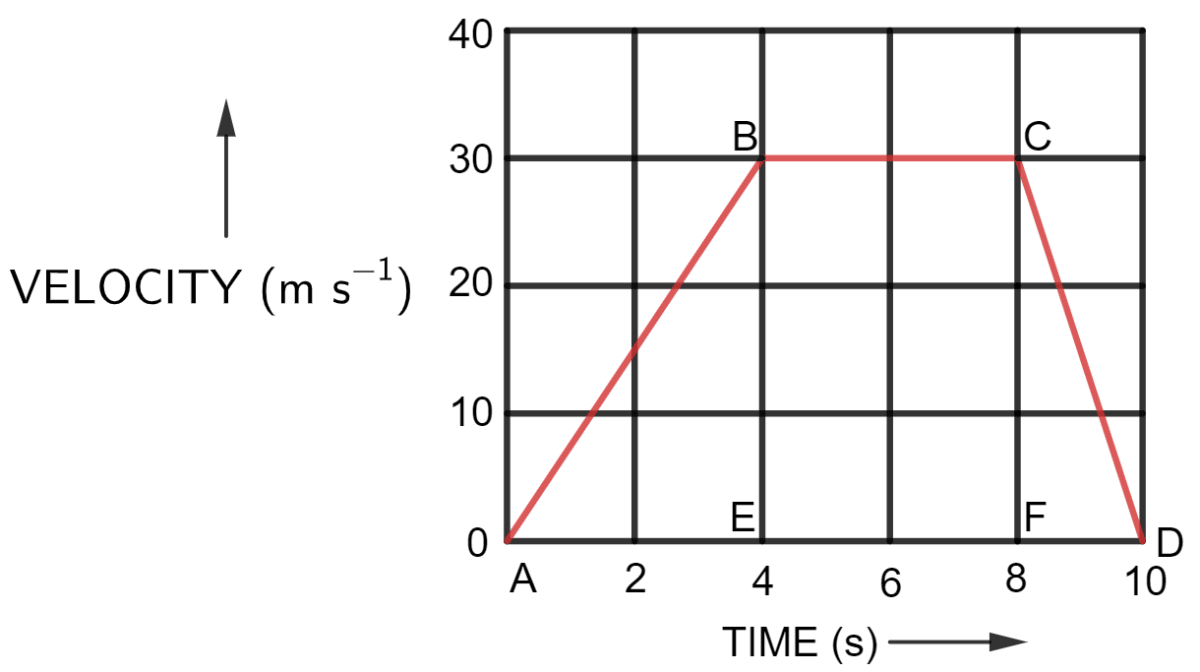
(i) Acceleration in part AB = slope of AB

$\text {slope of AB} = \dfrac{(30 - 0) \text {ms}^{-1}}{(4-0) \text {s}} \\[0.5em] \text {slope of AB} = \dfrac{30 \text {ms}^{-1}}{4 \text {s}} \\[0.5em] \text {slope of AB} = 7.5 \text {ms}^{-2} \\[0.5em]$

Hence, Acceleration in part AB = 7.5 m s^-2

Acceleration in part BC = slope of BC

We observe from the graph that there is no change in velocity in part BC. Hence, Acceleration in part BC = 0 m s^-1

Acceleration in part CD = slope of CD

$\text {slope of CD} = \dfrac{(0 - 30) \text {ms}^{-1}}{(10-8) \text {s}} \\[0.5em] \text {slope of CD} = \dfrac{-30 \text {ms}^{-1}}{2 \text {s}} \\[0.5em] \text {slope of CD} = -15 \text {ms}^{-2} \\[0.5em]$

Hence, Acceleration in part CD = -15 m s^-2

(ii) Displacement in each part is as follows —

(a) Displacement of part AB = Area of triangle ABE

$= \dfrac {1}{2} \times \text {base} \times \text {height}$

Substituting the values in the formula above, we get,

$= \dfrac {1}{2} \times {(4 -0) \text {s}} \times (30 - 0)\text {m s}^{-1} \\[0.5em] = \dfrac {1}{2} \times 4 \text {s} \times 30 \text {m s}^{-1} \\[0.5em] = 30 \text {m} \\[0.5em]$

Hence,
Displacement of part AB = 60 m

(b) Displacement of part BC = Area of Square EBCF

$= \text {length} \times \text {breadth}$

Substituting the values in the formula above, we get,

$= {(8 -4) \text {s}} \times (30 - 0)\text {m s}^{-1} \\[0.5em] = 4 \times 30 \text {m} \\[0.5em] = 120 \text {m} \\[0.5em]$

Hence,
Displacement of part BC = 120 m

(c) Displacement of part CD = Area of triangle CDF

$= \dfrac {1}{2} \times \text {base} \times \text {height}$

Substituting the values in the formula above, we get,

$= \dfrac {1}{2} \times {(10 -8) \text {s}} \times (30 - 0)\text {m s}^{-1} \\[0.5em] = \dfrac {1}{2} \times 2 \times 30 \text {m} \\[0.5em] = 30 \text {m} \\[0.5em]$

Hence,
Displacement of part CD = 30 m

(iii) Total displacement = Displacement of part AB + Displacement of part BC + Displacement of part CD
= 60 + 30 + 120 = 210

Hence,
total displacement = 210 m