Mathematics
The ordinate of a point lying on the line joining the points (6, 4) and (7, -5) is -23. Find the co-ordinates of that point.
Straight Line Eq
3 Likes
Answer
Let point A = (6, 4) and B = (7, -5).
Let the point P be (a, -23).
By formula,
Slope =
Slope of AB = = -9.
By point-slope form,
⇒ y - y1 = m(x - x1)
⇒ y - 4 = -9(x - 6)
⇒ y - 4 = -9x + 54
⇒ 9x + y = 54 + 4
⇒ 9x + y = 58.
Since, P lies on AB so it will satisfy the equation.
Substituting values of P in equation we get,
⇒ 9(a) + (-23) = 58
⇒ 9a - 23 = 58
⇒ 9a = 58 + 23
⇒ 9a = 81
⇒ a =
⇒ a = 9.
∴ P = (a, -23) = (9, -23).
Hence, co-ordinates of the required point are (9, -23).
Answered By
2 Likes
Related Questions
Write down the equation of the line whose gradient is and which passes through P, where P divides the line segment joining A(-2, 6) and B(3, -4) in the ratio 2 : 3.
Point A and B have co-ordinates (7, -3) and (1, 9) respectively. Find :
(i) the slope of AB.
(ii) the equation of perpendicular bisector of the line segment AB.
(iii) the value of 'p' if (-2, p) lies on it.
A and B are the two points on the x-axis and y-axis respectively. P(2, -3) is the mid-point of AB.

Find :
(i) the coordinates of A and B.
(ii) the slope of the line AB.
(iii) the equation of the line AB.
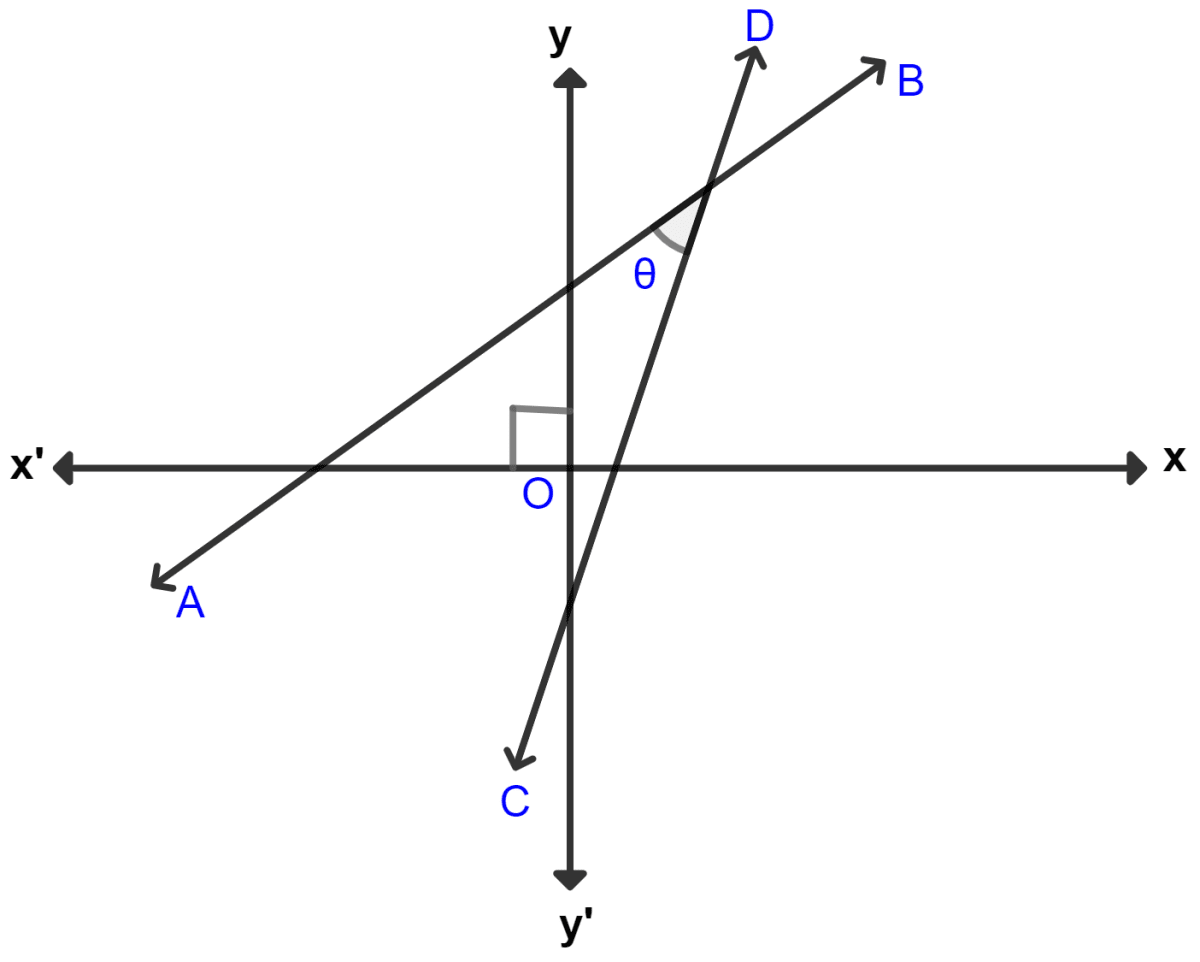
The given figure (not drawn to scale) shows two straight lines AB and CD. If equation of the line AB is : y = x + 1 and equation of line CD is : y = x - 1. Write down the inclination of lines AB and CD; also, find the angle θ between AB and CD.