Mathematics
The marks obtained by 120 students in a Mathematics test are given below :
| Marks | No. of students |
|---|---|
| 0 - 10 | 5 |
| 10 - 20 | 9 |
| 20 - 30 | 16 |
| 30 - 40 | 22 |
| 40 - 50 | 26 |
| 50 - 60 | 18 |
| 60 - 70 | 11 |
| 70 - 80 | 6 |
| 80 - 90 | 4 |
| 90 - 100 | 3 |
Draw an ogive for the given distribution on a graph sheet. Use a suitable scale for your ogive. Use your ogive to estimate :
(i) the median
(ii) the number of students who obtained more than 75% marks in a test ?
(iii) the number of students who did not pass in the test if the pass percentage was 40?
(iv) the lower quartile.
Measures of Central Tendency
18 Likes
Answer
Cumulative frequency distribution table :
| Marks | No. of students | Cumulative frequency |
|---|---|---|
| 0 - 10 | 5 | 5 |
| 10 - 20 | 9 | 14 (5 + 9) |
| 20 - 30 | 16 | 30 (14 + 16) |
| 30 - 40 | 22 | 52 (30 + 22) |
| 40 - 50 | 26 | 78 (52 + 26) |
| 50 - 60 | 18 | 96 (78 + 18) |
| 60 - 70 | 11 | 107 (96 + 11) |
| 70 - 80 | 6 | 113 (107 + 6) |
| 80 - 90 | 4 | 117 (113 + 4) |
| 90 - 100 | 3 | 120 (117 + 3) |
(i) Steps of construction of ogive :
Take 1 cm = 10 marks on x-axis.
Take 1 cm = 20 students on y-axis.
Plot the point (0, 0) as ogive starts from x-axis representing lower limit of first class.
Plot the points (10, 5), (20, 14), (30, 30), (40, 52), (50, 78), (60, 96), (70, 107), (80, 113), (90, 117) and (100, 120).
Join the points by a free hand curve.
Draw a line parallel to x-axis from point A (no. of students) = 60, touching the graph at point B. From point B draw a line parallel to y-axis touching x-axis at point C.
From graph, C = 43
Hence, median = 43.
(ii) Total marks = 100.
75% of 100 marks = = 75.
Draw a line parallel to y-axis from point D (marks) = 75, touching the graph at point E. From point E draw a line parallel to x-axis touching y-axis at point F.
From graph, F = 110.
It means that 110 students score either less or equal to 75% marks.
No. of students left = 120 - 110 = 10.
Hence, no. of students scoring more than 75% marks = 10.
(iii) Total marks = 100.
40% of 100 marks = = 40.
Draw a line parallel to y-axis from point G (marks) = 40, touching the graph at point H. From point H draw a line parallel to x-axis touching y-axis at point I.
From graph, I = 52.
Hence, no. of failed students = 52.
(iv) Here, n = 120, which is even.
By formula,
Lower quartile = = 30th term.
Draw a line parallel to x-axis from point J (no. of students) = 30, touching the graph at point K. From point K draw a line parallel to y-axis touching x-axis at point L.
From graph, L = 30
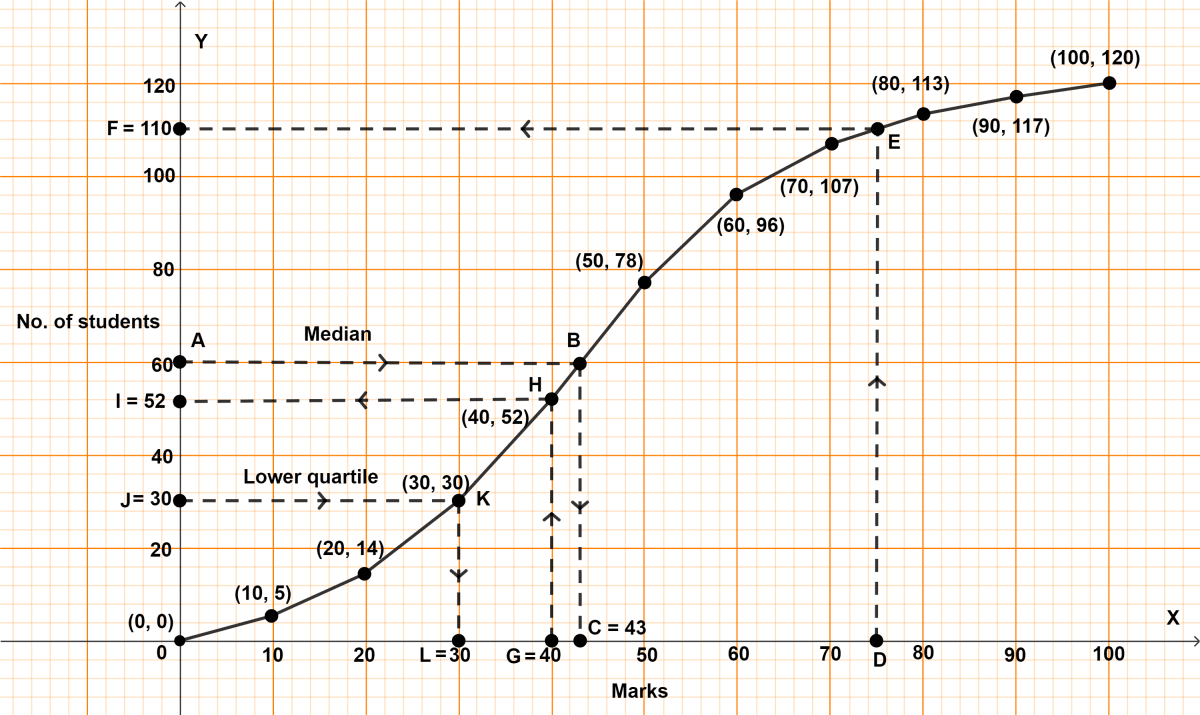
Hence, lower quartile = 30.
Answered By
13 Likes
Related Questions
The mean of 1, 7, 5, 3, 4 and 4 is m. The numbers 3, 2, 4, 2, 3, 3 and p have mean m - 1 and median q. Find p and q.
In a malaria epidemic, the number of cases diagnosed were as follows :
Date (July) Number 1 5 2 12 3 20 4 27 5 46 6 30 7 31 8 18 9 11 10 5 11 0 12 1 On what days do the mode, the upper and the lower quartiles occur ?
Using a graph paper, draw an ogive for the following distribution which shows a record of the weight in kilograms of 200 students.
Weight Frequency 40 - 45 5 45 - 50 17 50 - 55 22 55 - 60 45 60 - 65 51 65 - 70 31 70 - 75 20 75 - 80 9 Use your ogive to estimate the following :
(i) The percentage of students weighing 55 kg or more.
(ii) The weight above which the heaviest 30% of the students fall,
(iii) The number of students who are (a) under-weight and (b) over weight, if 55.70 kg is considered as standard weight ?
The distribution given below, shows the marks obtained by 25 students in an aptitude test. Find the mean, median and mode of the distribution.
Marks obtained No. of students 5 3 6 9 7 6 8 4 9 2 10 1