Mathematics
The locus of the centers of all circles, which are tangents to the arms AB and BC of angle ABC is :
perpendicular bisector of arm AB
perpendicular bisector of arm BC
bisector of angle ABC
none of these
Answer
From figure,
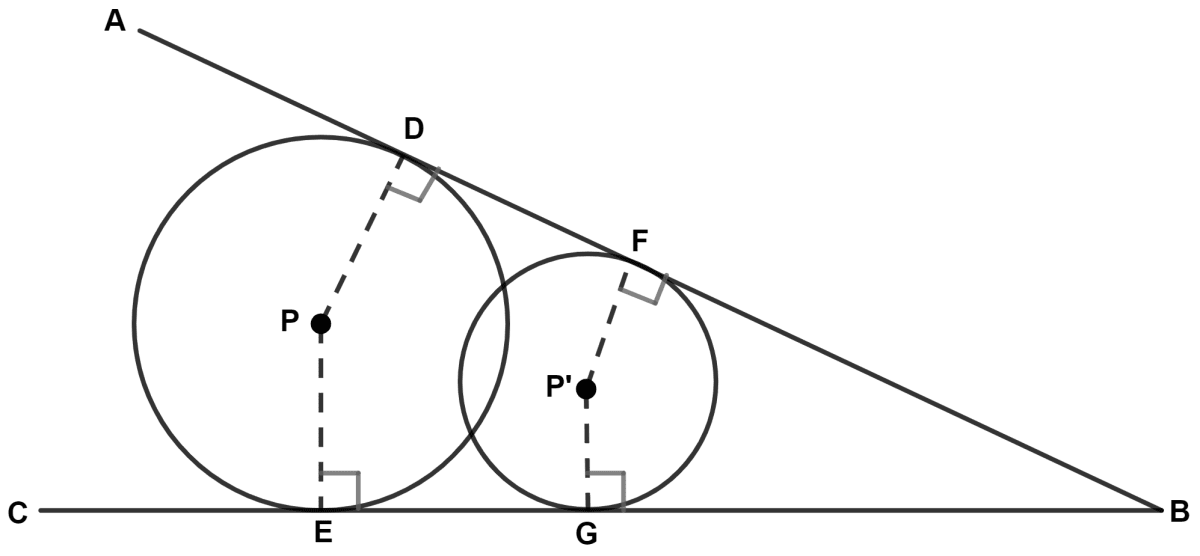
PD = PE (Radius of circle with center P)
P'F = P'G (Radius of circle with center P')
∴ The centers of circles are equidistant from the lines AB and BC.
We know that,
The locus of a point equidistant from two intersecting lines is the bisector of the angles between the lines.
∴ The locus of the centers of all circles, which are tangents to the arms AB and BC of angle ABC is the bisector of angle ABC.
Hence, Option 3 is the correct option.
Related Questions
In triangle LMN, bisectors of interior angles at L and N intersect each other at point A. Prove that:
(i) point A is equidistant from all the three sides of the triangle.
(ii) AM bisects angle LMN.
Use ruler and compasses only for this question.
(i) Construct △ABC, where AB = 3.5 cm, BC = 6 cm and ∠ABC = 60°.
(ii) Construct the locus of points inside the triangle which are equidistant from BA and BC.
(iii) Construct the locus of points inside the triangle which are equidistant from B and C.
(iv) Mark the point P which is equidistant from AB, BC and also equidistant from B and C. Measure and record the length of PB.
P is a moving point and AB is a chord of a circle. If P moves, within this circle, in such a way that it is at equal distances from points A and B. The locus of P is :
a chord perpendicular to chord AB
a chord that bisects the chord AB
a diameter of the circle
the diameter of the circle which is perpendicular to chord AB
AB is a line segment. A point P moves in such a way that the triangle APB is always an isosceles triangle with base AB. The locus of point P is the line which :
is parallel to AB
is perpendicular to AB
is perpendicular bisector of AB
passes through the mid-point of AB.