The line joining mid-points of two chords of a circle passes through its center. Prove that the chords are parallel.

In the figure, AB and CD are the two chords of a circle with center O. M and N are mid-points of AB and CD, respectively and MN is the line joining the mid-points of two chords and passing through center O.

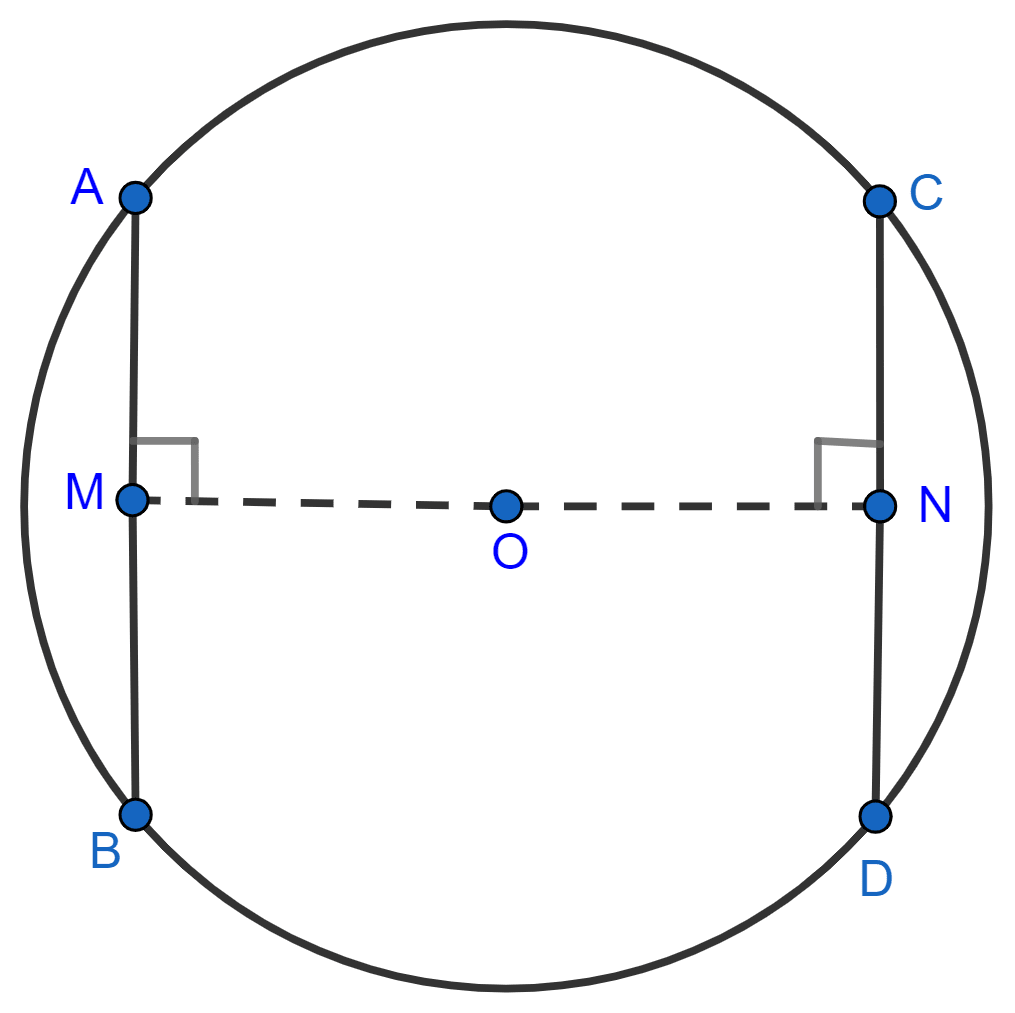
Since, the straight line drawn from the centre of a circle to bisect a chord is perpendicular to the chord,

∴ OM ⊥ AB and ON ⊥ CD.

So,

∠OMA = ∠OMB = 90° and ∠ONC = ∠OND = 90°

Since, ∠OMA = ∠OND = 90° (Alternate angles) and,

∠OMB = ∠ONC = 90° (Alternate angles)

Hence, proved that AB || CD.