Mathematics
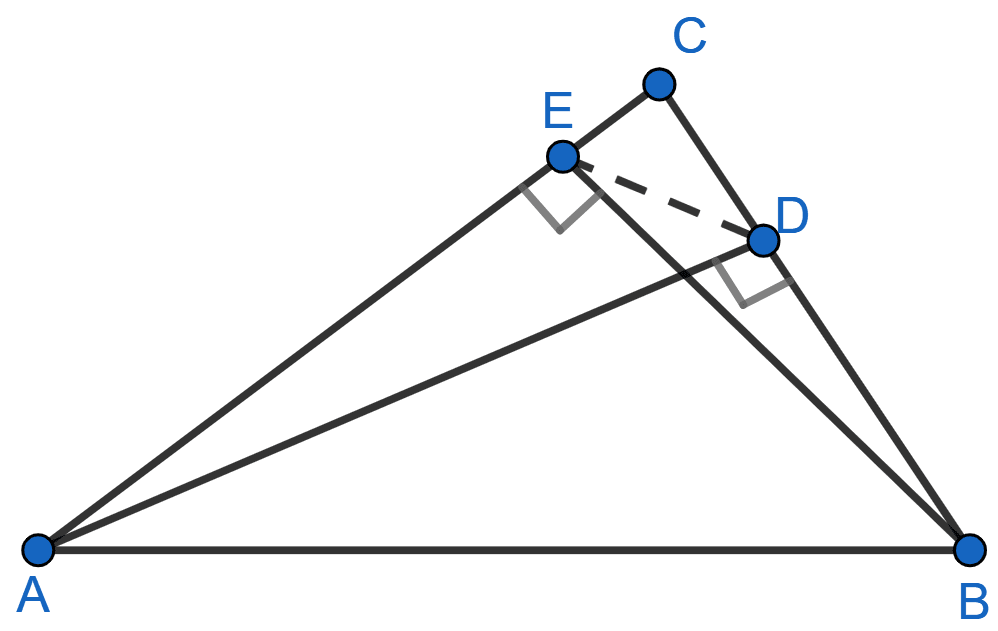
The following figure shows a triangle ABC in which AD and BE are perpendiculars to BC and AC respectively.
Show that :
(i) △ADC ~ △BEC
(ii) CA × CE = CB × CD
(iii) △ABC ~ △DEC
(iv) CD × AB = CA × DE
Similarity
2 Likes
Answer
(i) In △ADC and △BEC,
∠ADC = ∠BEC [Both = 90°]
∠ACD = ∠BCE [Common angle]
∴ △ADC ~ △BEC [By AA].
Hence, proved that △ADC ~ △BEC.
(ii) Since, △ADC ~ △BEC.
We know that,
Corresponding sides of similar triangles are equal.
⇒ CA × CE = CB × CD.
Hence, proved that CA × CE = CB × CD.
(iii) From part (ii) we get,
∠DCE = ∠BCA [Common angle]
∴ △ABC ~ △DEC [By SAS]
Hence, proved that △ABC ~ △DEC.
(iv) Since, △ABC ~ △DEC
We know that,
Corresponding sides of similar triangles are proportional to each other.
⇒
⇒ CD × AB = CA × DE.
Hence, proved that CD × AB = CA × DE.
Answered By
2 Likes
Related Questions
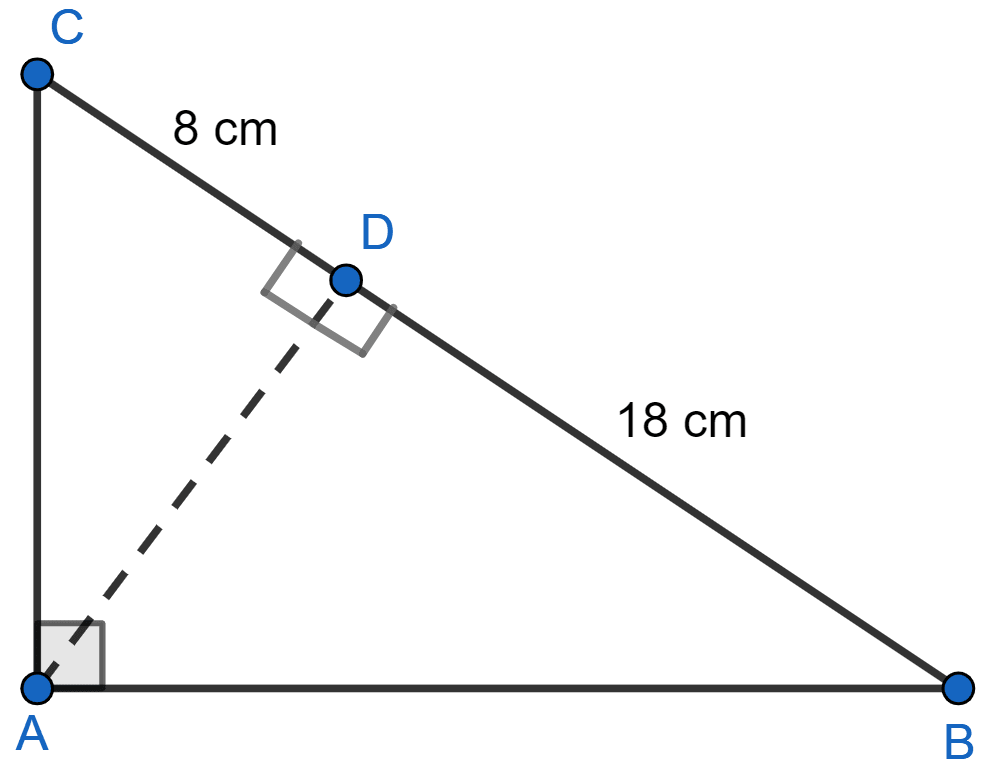
In the given figure, ABC is a right angled triangle with ∠BAC = 90°.
(i) Prove that : △ADB ~ △CDA.
(ii) If BD = 18 cm and CD = 8 cm, find AD.
(iii) Find the ratio of the area of △ADB is to area of △CDA.
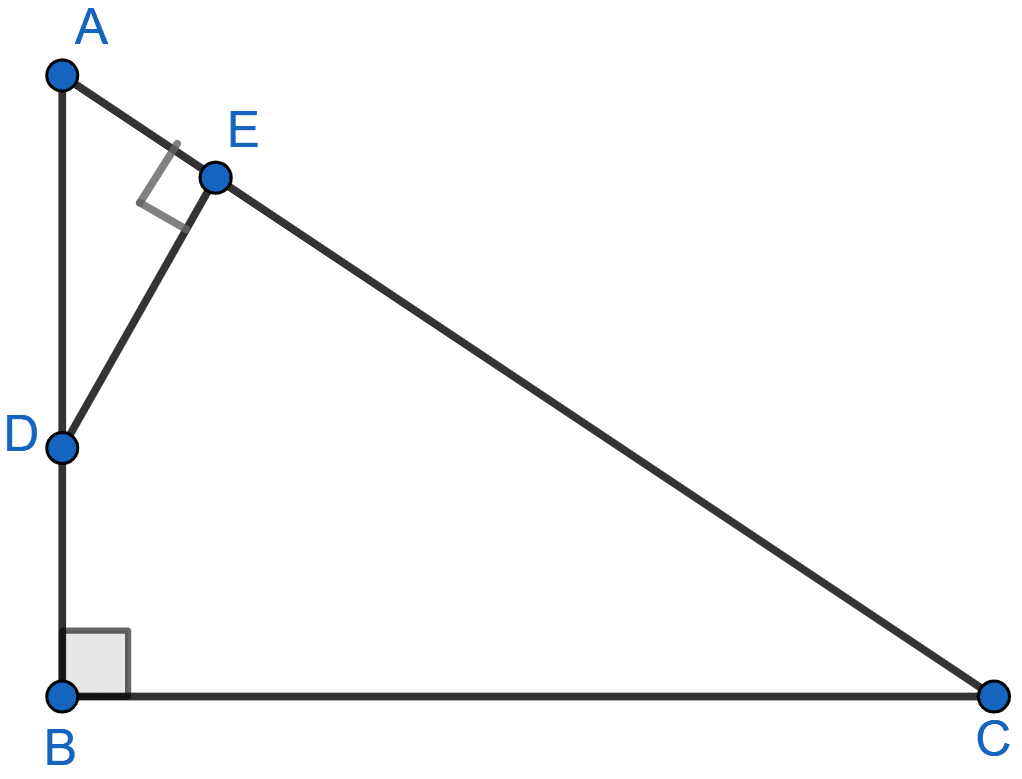
ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :
(i) △ADE ~ △ACB
(ii) If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
(iii) Find, area of △ADE : area of quadrilateral BCED.
Given : AB || DE and BC || EF. Prove that :
(i)
(ii) △DFG ~ △ACG.

PQR is a triangle. S is a point on the side QR of △PQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
(i) Prove △PQR ~ △SPR.
(ii) Find the lengths of QR and PS.
(iii)
