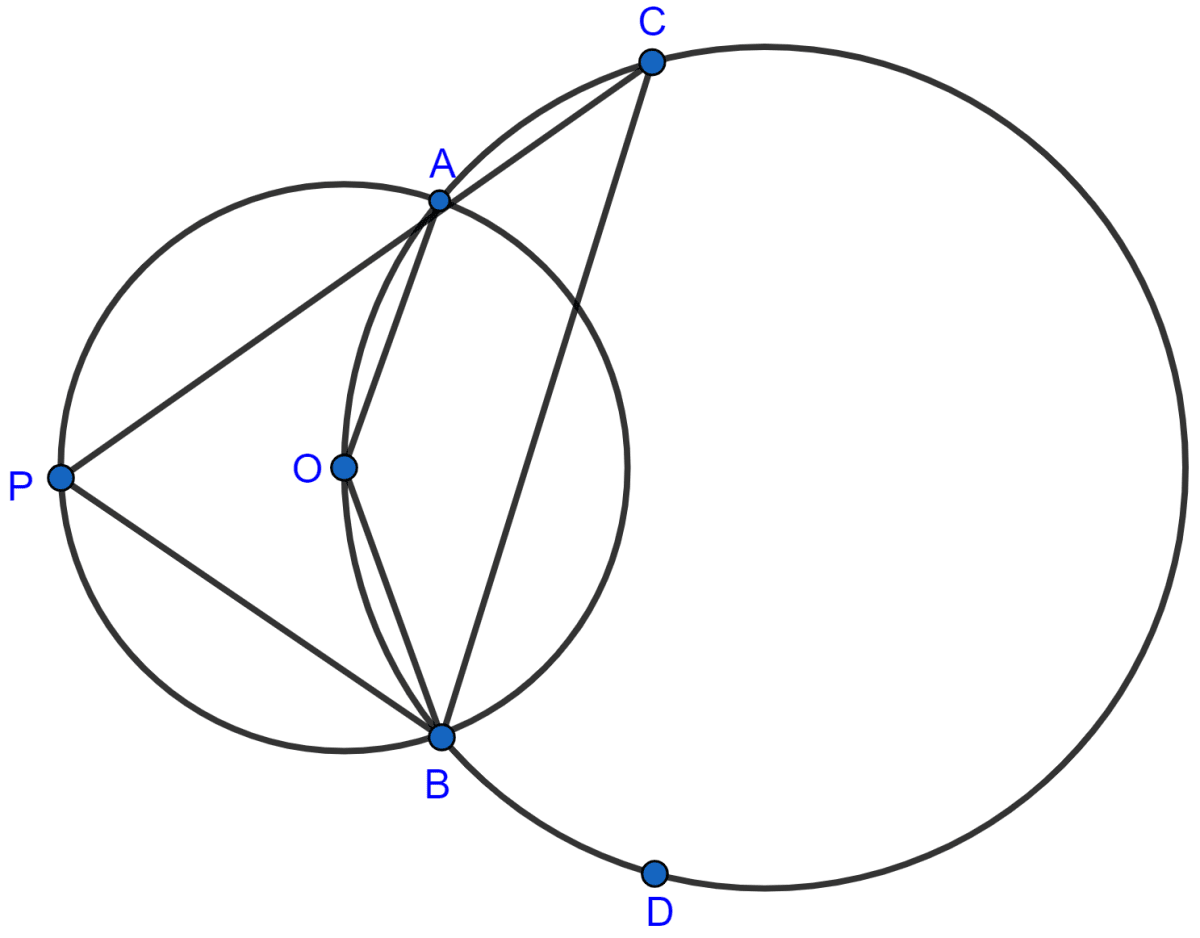
The figure shows two circles which intersect at A and B. The center of the smaller circle is O and lies on the circumference of the larger circle. Given ∠APB = a°.

Calculate, in terms of a°, the value of :

(i) obtuse ∠AOB,

(ii) ∠ACB,

(iii) ∠ADB.

Give reasons for your answers clearly.

(i) We know that,

Angle at the center is double the angle at the circumference subtended by the same chord.

obtuse ∠AOB = 2∠APB = 2a°.

Hence, obtuse ∠AOB = 2a°.

(ii) OACB is a cyclic quadrilateral.

⇒ ∠AOB + ∠ACB = 180° [Sum of opposite angles in a cyclic quadrilateral = 180°.]

⇒ ∠ACB + 2a° = 180°

⇒ ∠ACB = 180° - 2a°.

Hence, ∠ACB = 180° - 2a°.

(iii) Join AD and BD.

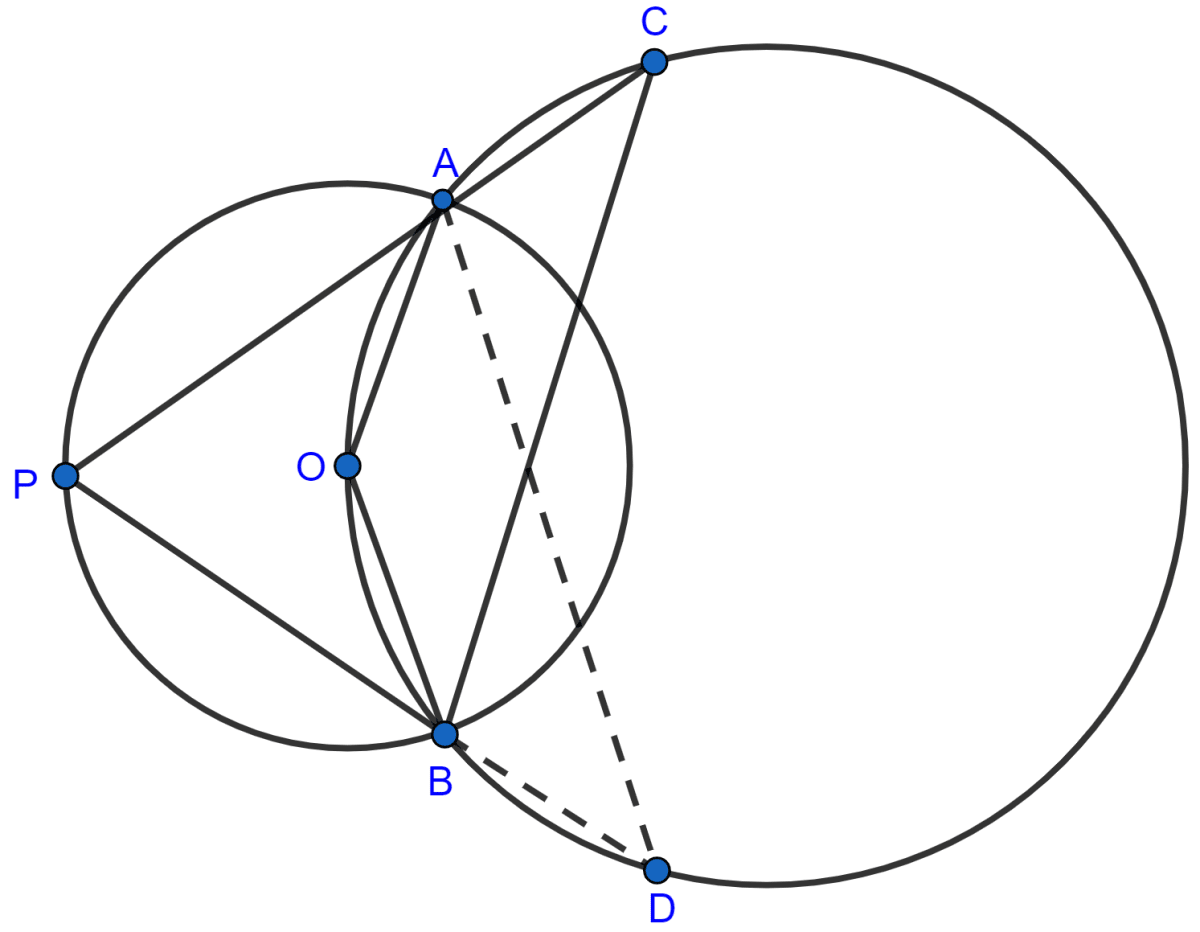
As, angles in same segment are equal.

∴ ∠ADB = ∠ACB = 180° - 2a°.

Hence, ∠ADB = 180° - 2a°.