Mathematics
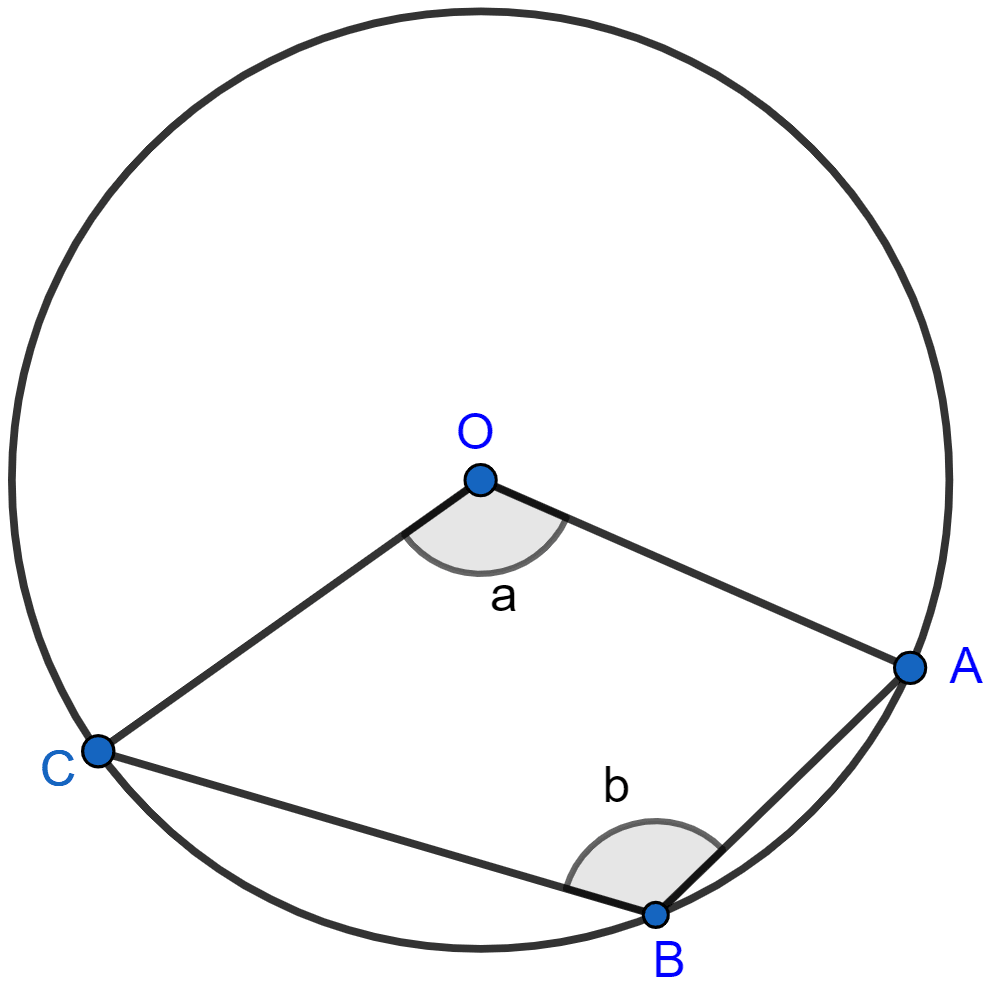
The figure given below, shows a circle with centre O.
Given : ∠AOC = a and ∠ABC = b.
(i) Find the relationship between a and b.
(ii) Find the measure of angle OAB, if OABC is a parallelogram.
Circles
17 Likes
Answer
(i) We know that,
Angle at the centre is double the angle at the circumference subtended by the same chord
∴ ∠ABC = Reflex ∠COA
⇒ b = (360° - a)
⇒ 2b = 360° - a
⇒ a + 2b = 360° …..(1)
Hence, relationship between a and b is given by the equation : a + 2b = 360°.
(ii) From equation 1, we get :
⇒ a + 2b = 360°
⇒ a = 360° - 2b
As OABC is a parallelogram, the opposite angles are equal.
So, a = b
⇒ 360° - 2b = b
⇒ 3b = 360°
⇒ b = = 120°
Let ∠OAB = x and ∠OCB = x.
⇒ ∠OAB + ∠OCB + ∠AOC + ∠ABC = 360°
⇒ x + x + a + b = 360°
⇒ 2x + 120° + 120° = 360°
⇒ 2x + 240° = 360°
⇒ 2x = 120°
⇒ x = = 60°.
Hence, ∠OAB = 60°.
Answered By
11 Likes
Related Questions
Prove that:
(i) the parallelogram, inscribed in a circle, is a rectangle.
(ii) the rhombus, inscribed in a circle, is a square.
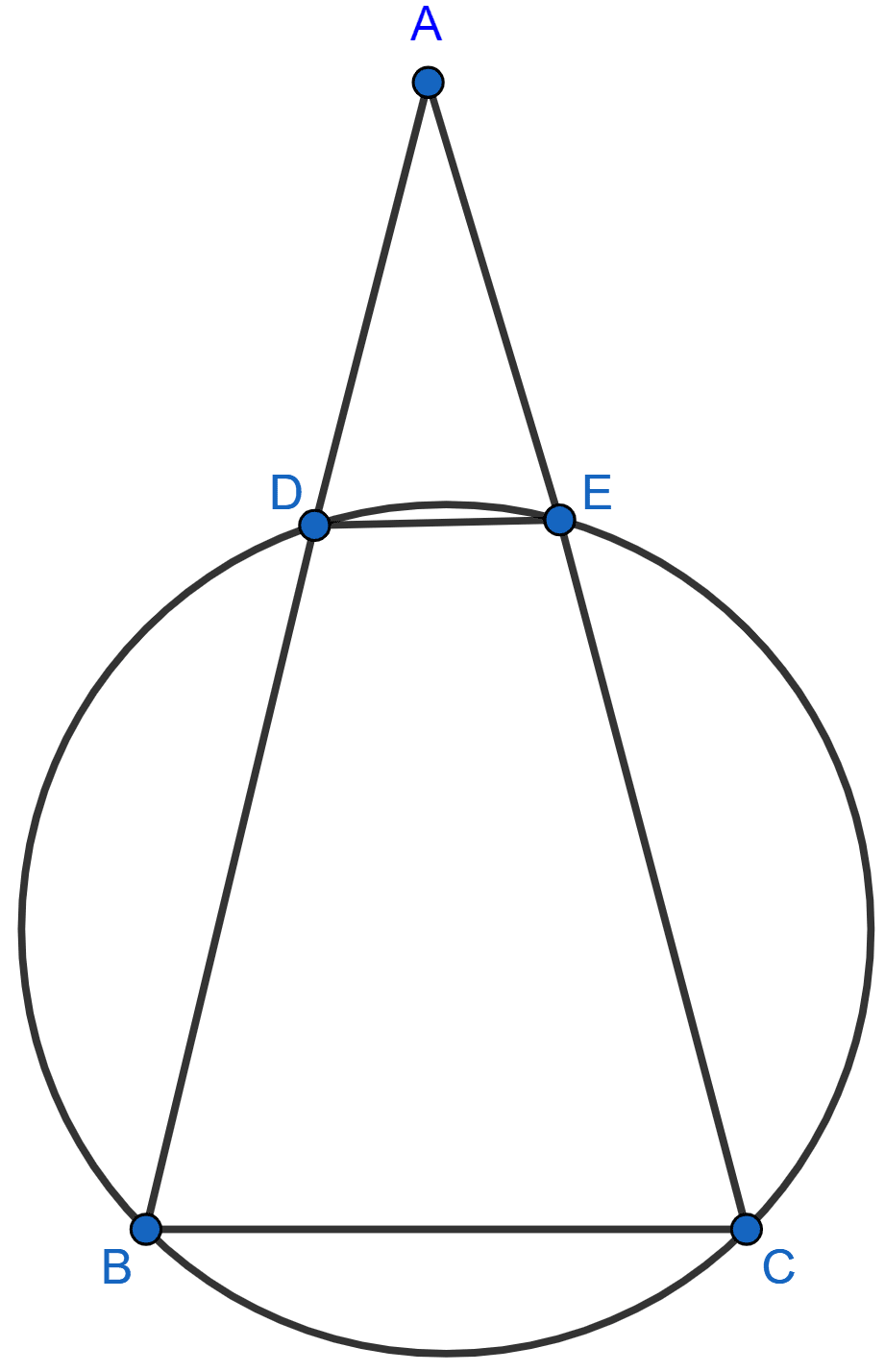
In the given figure, AB = AC. Prove that DECB is an isosceles trapezium.
In the figure given, RS is a diameter of the circle. NM is parallel to RS and ∠MRS = 29°.
Calculate :
(i) ∠RNM,
(ii) ∠NRM.

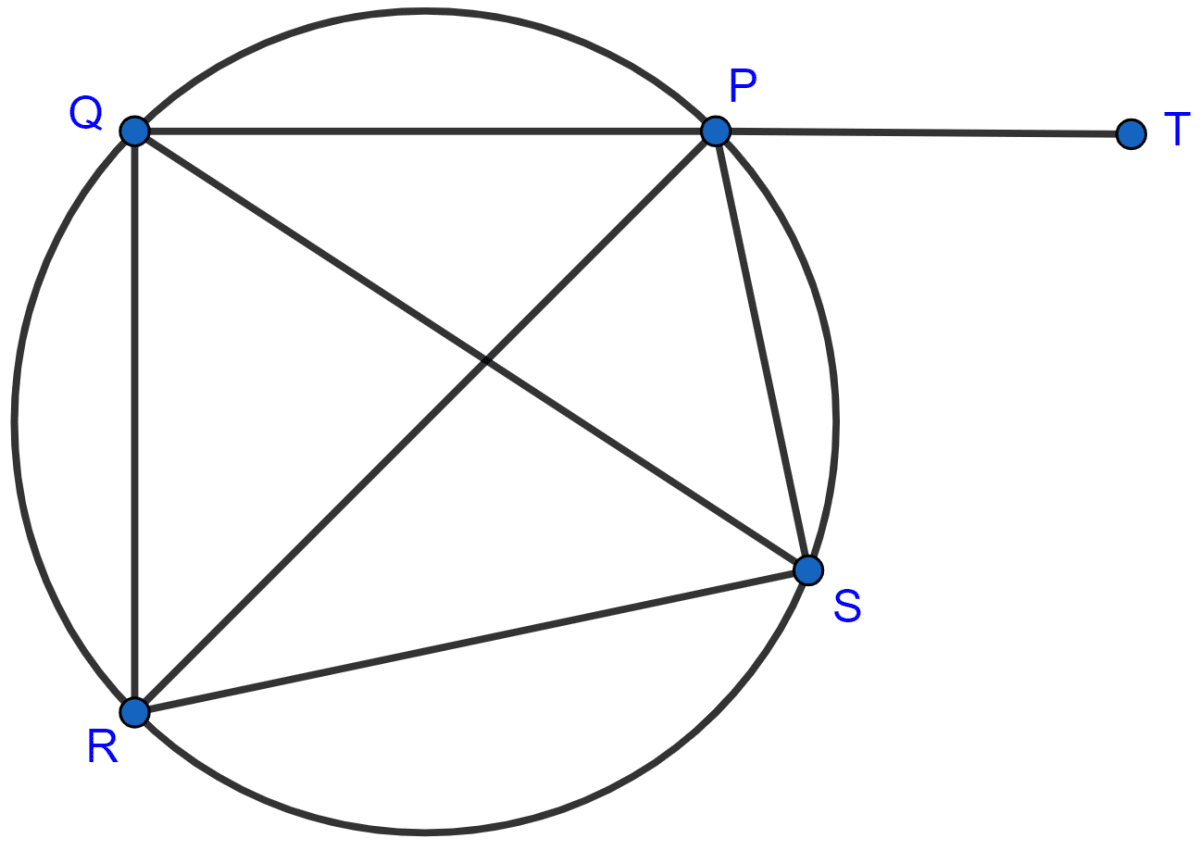
In the given figure, SP is the bisector of ∠RPT and PQRS is a cyclic quadrilateral. Prove that :
SQ = SR.