Mathematics
The difference between the outer and the inner curved surface areas of a hollow cylinder, 14 cm long, is 88 sq. cm. Find the outer and the inner radii of the cylinder, given that the volume of metal used is 176 cu. cm.
Mensuration
2 Likes
Answer
Given,
Height of cylinder (H) = 14 cm
Let outer radius of cylinder be R cm and inner radius be r cm.
Given,
Difference between the outer and the inner curved surface areas of a hollow cylinder is 88 sq. cm.
Given,
Volume of metal = 176 cu. cm
Adding equation (1) and (2), we get :
⇒ R - r + R + r = 1 + 4
⇒ 2R = 5
⇒ R = = 2.5 cm
Substituting value of R in equation (1), we get :
⇒ 2.5 - r = 1
⇒ r = 2.5 - 1 = 1.5 cm
Hence, outer radii = 2.5 cm and inner radii = 1.5 cm.
Answered By
2 Likes
Related Questions
A test tube consists of a hemisphere and a cylinder of the same radius. The volume of water required to fill the whole tube is cm3 and cm3 of water is required to fill the tube to a level which is 2 cm below the top of the tube. Find the radius of the tube and the length of its cylindrical part.

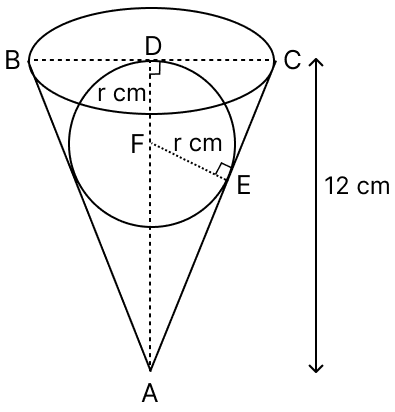
A sphere is placed in an inverted hollow conical vessel of base radius 5 cm and vertical height 12 cm. If the highest point of the sphere is at the level of the base of the cone, find the radius of the sphere. Show that the volume of the sphere and the conical vessel are as 40 : 81.
The surface area of a solid sphere is increased by 21% without changing its shape. Find the percentage increase in its :
(i) radius
(ii) volume
A wooden toy is in the shape of a cone mounted on a cylinder as shown alongside.
If the height of the cone is 24 cm, the total height of the toy is 60 cm and the radius of the base of the cone = twice the radius of the base of the cylinder = 10 cm; find the total surface area of the toy. [Take π = 3.14]