The center of a circle is C(2α - 1, 3α + 1) and it passes through the point A(-3, -1). If a diameter of the circle is of length 20 units, find the value(s) of α.

Given,

Diameter = 20 units

Radius = $\dfrac{\text{Diameter}}{2} = \dfrac{20}{2}$ = 10 units.

∴ AC = 10 units

$\Rightarrow \sqrt{[-3 - (2α - 1)]^2 + [-1 - (3α + 1)]^2} = 10 \\[1em] \Rightarrow \sqrt{(-3 - 2α + 1)^2 + (-1 - 3α - 1)^2} = 10 \\[1em] \Rightarrow \sqrt{(-2 - 2α)^2 + (-3α - 2)^2} = 10 \\[1em] \Rightarrow \sqrt{4 + 4α^2 + 8α + 9α^2 + 4 + 12α} = 10 \\[1em] \Rightarrow \sqrt{13α^2 + 20α + 8} = 10$

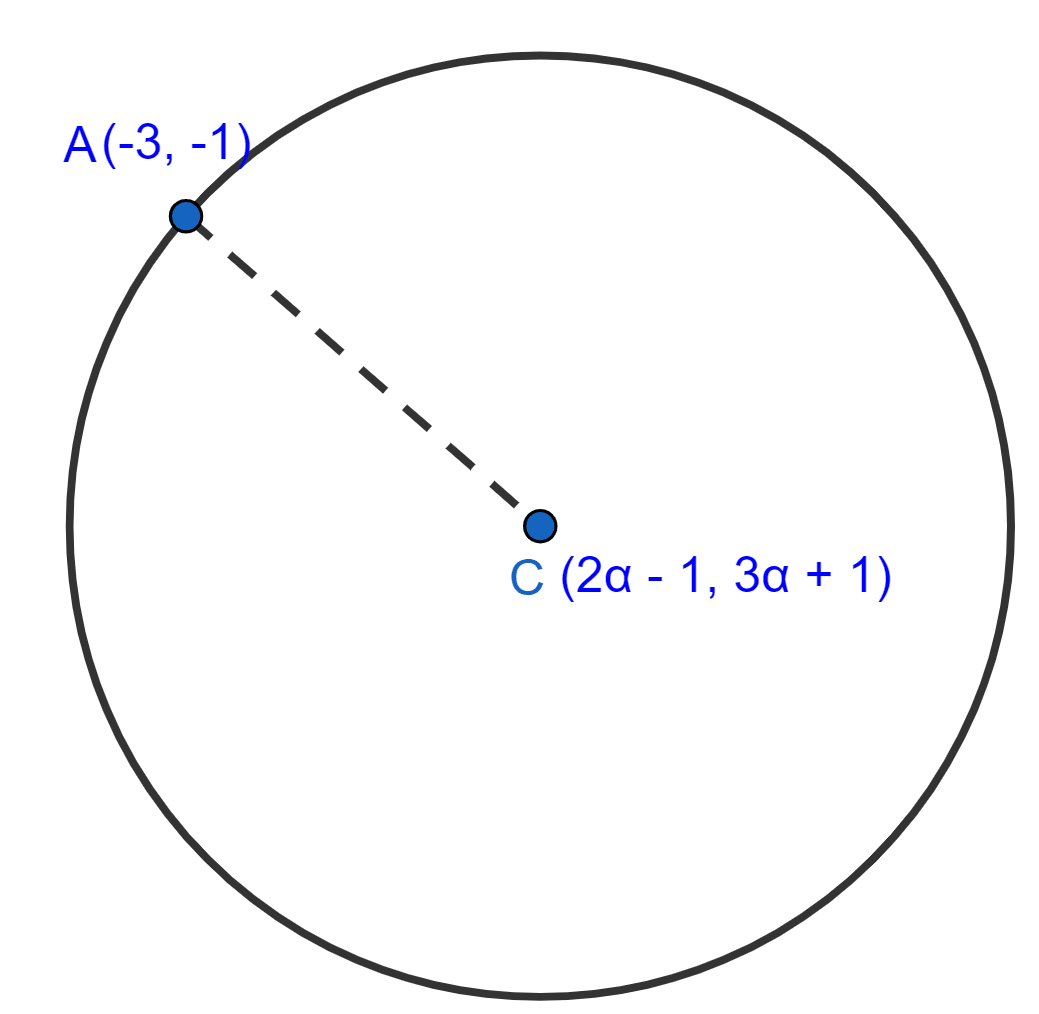
On squaring both sides,

$\Rightarrow 13α^2 + 20α + 8 = 10^2 \\[1em] \Rightarrow 13α^2 + 20α + 8 = 100 \\[1em] \Rightarrow 13α^2 + 20α + 8 - 100 = 0 \\[1em] \Rightarrow 13α^2 + 20α - 92 = 0 \\[1em] \Rightarrow 13α^2 + 46α - 26α - 92 =0 \\[1em] \Rightarrow α(13α + 46) - 2(13α + 46) = 0 \\[1em] \Rightarrow (13α + 46)(α - 2) = 0 \\[1em] \Rightarrow 13α + 46 = 0 \text{ or } α - 2 = 0 \\[1em] \Rightarrow 13α = -46 \text{ or } α = 2 \\[1em] \Rightarrow α = -\dfrac{46}{13} \text{ or } α = 2.$

Hence, α = $-\dfrac{46}{13}$ or α = 2.