Mathematics
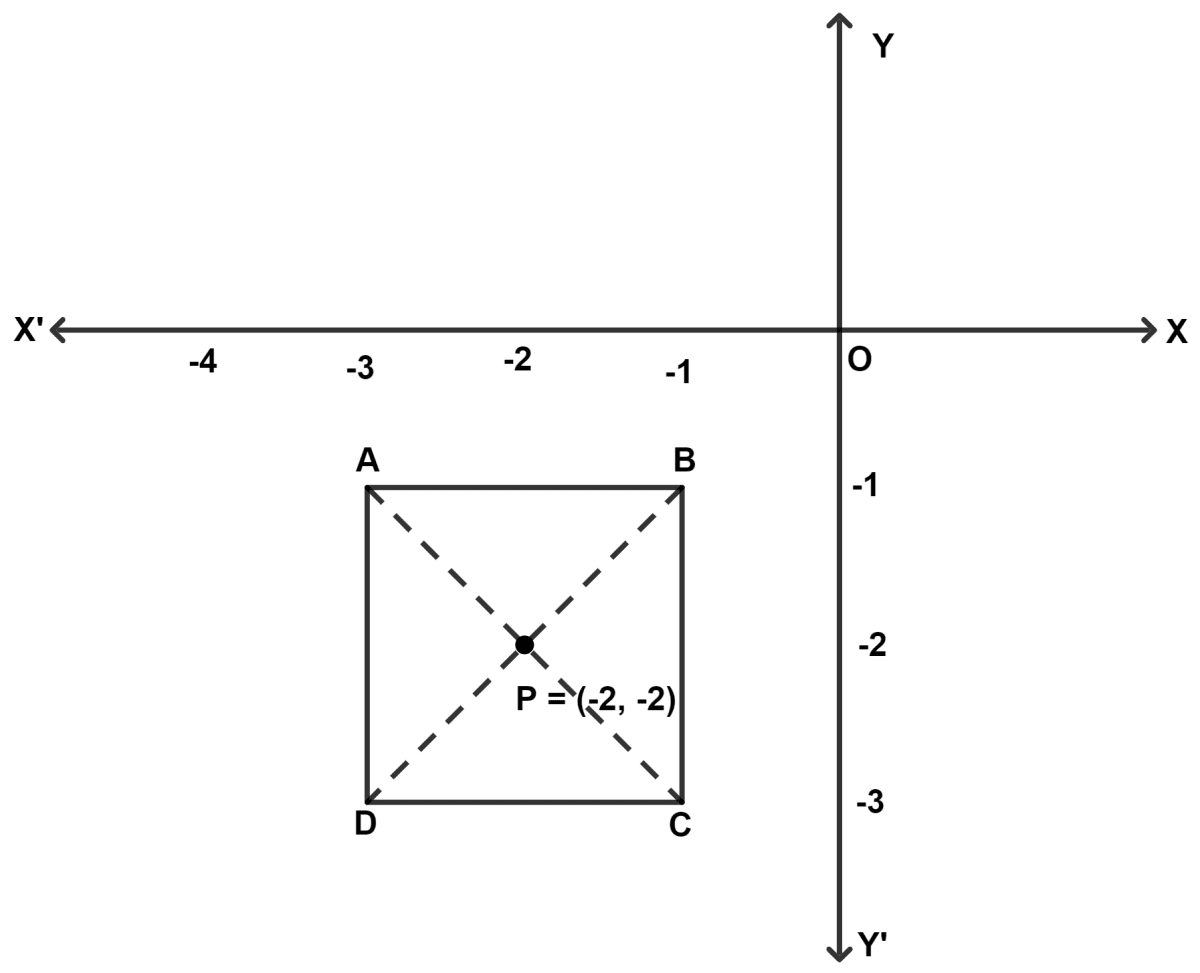
Square ABCD lies in the third quadrant of a XY plane such that its vertex A is at (-3, -1) and the diagonal DB produced is equally inclined to both the axes. The diagonals AC and BD meets at P(-2, -2). Find the :
(a) Slope of BD
(b) equation of AC
Answer
(a) Given,
BD is equally inclined to both axes.
∴ Slope of BD = tan 45° = 1.
Hence, slope of BD = 1.
(b) Since, diagonals of a square are perpendicular and product of slope of perpendicular lines equals to -1.
∴ Slope of BD × Slope of AC = -1
⇒ 1 × Slope of AC = -1
⇒ Slope of AC = -1.
Since, diagonals meet at point (-2, -2).
By point-slope form,
⇒ y - y1 = m(x - x1)
⇒ y - (-2) = -1[x - (-2)]
⇒ y + 2 = -1[x + 2]
⇒ y + 2 = -x - 2
⇒ x + y + 2 + 2 = 0
⇒ x + y + 4 = 0.
Hence, equation of AC is x + y + 4 = 0.
Related Questions
ABC is a triangle as shown in the figure below.
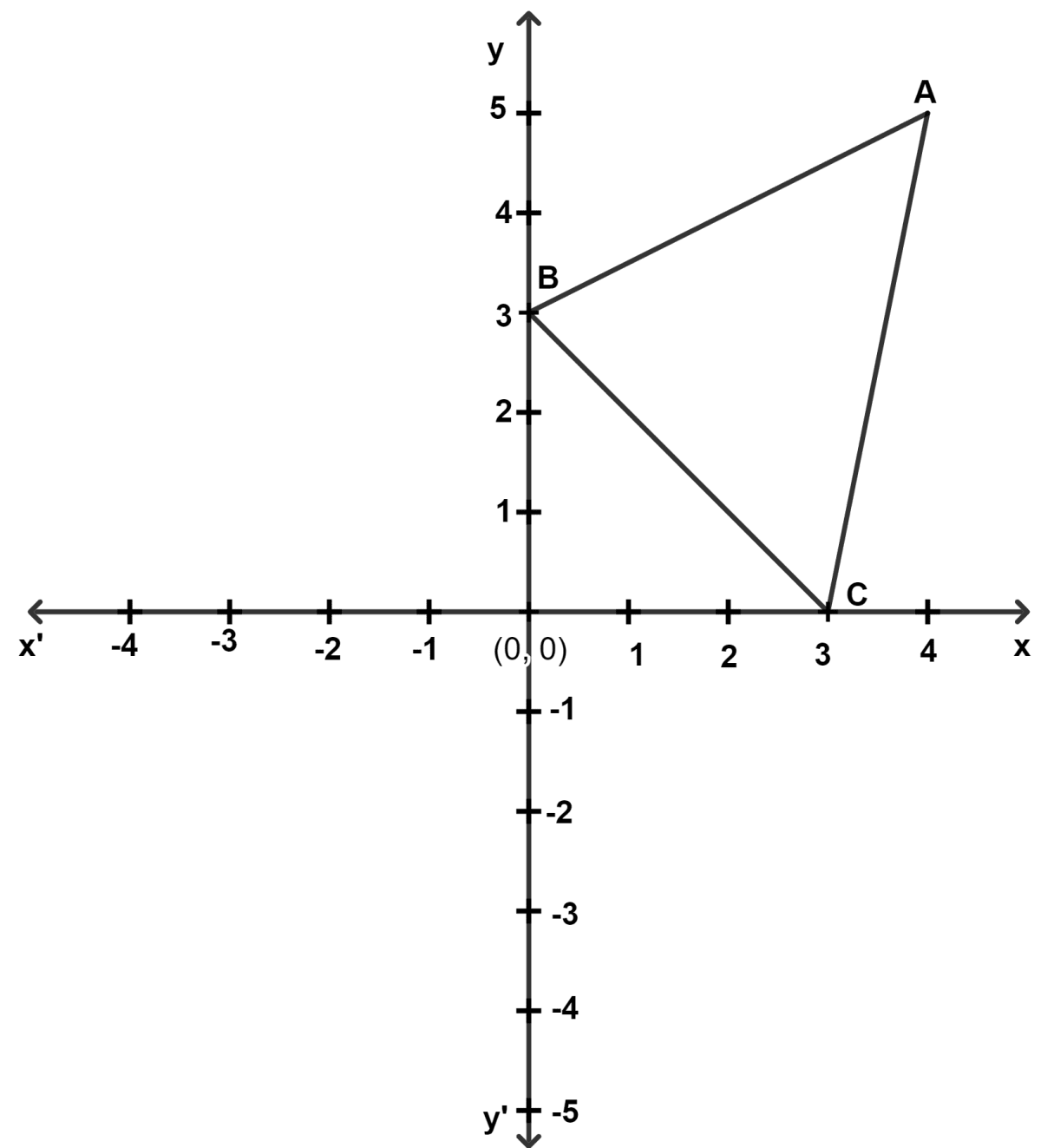
(a) Write down the coordinates of A, B and C on reflecting through the origin.
(b) Write down the coordinates of the point/s which remain invariant on reflecting the triangle ABC on the x-axis and y-axis respectively.
Determine the ratio in which the line y = 2 + 3x divides the line segment AB joining the points A(-3, 9) and B(4, 2).
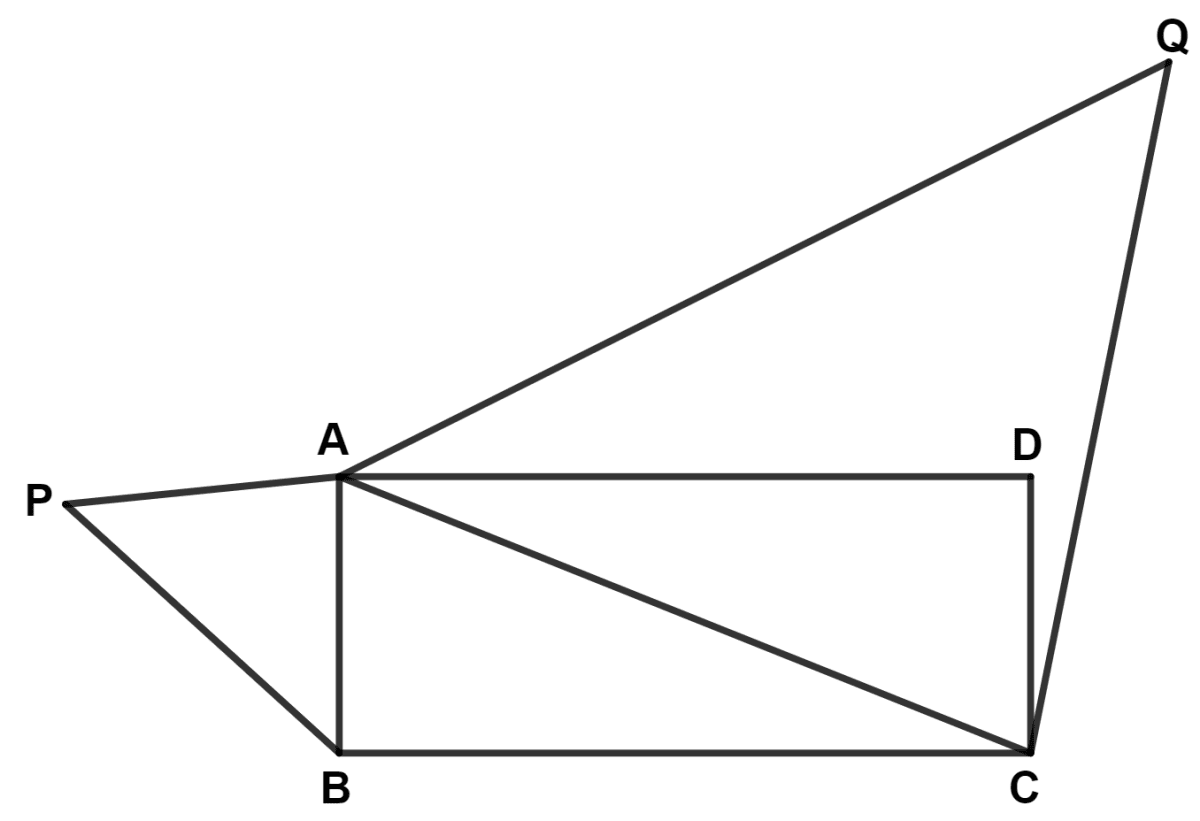
ABCD is a rectangle where side BC is twice side AB. If △ACQ ~ △BAP, find area of △BAP : area of △ACQ.
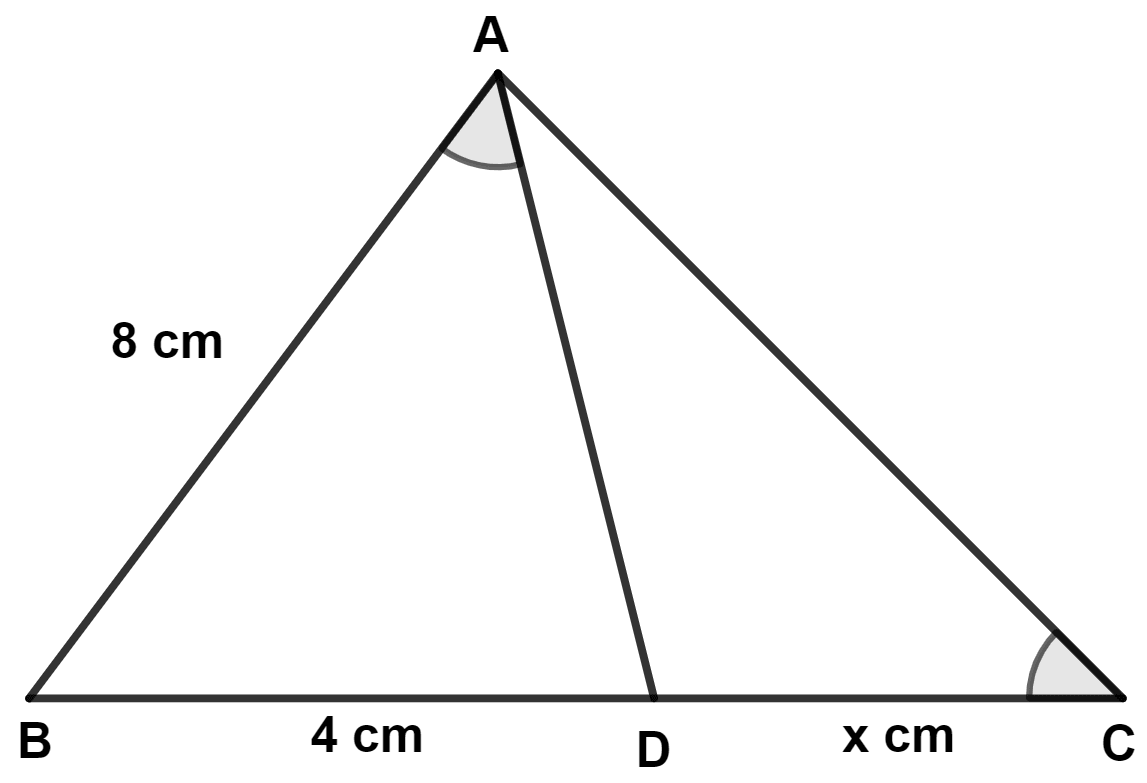
Given a triangle ABC, and D is a point on BC such that BD = 4 cm and DC = x cm. If ∠BAD = ∠C and AB = 8 cm, then,
(a) prove that triangle ABD is similar to triangle CBA.
(b) find the value of 'x'.