Mathematics
PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate :
(i) ∠CBT
(ii) ∠BAT
(iii) ∠APT
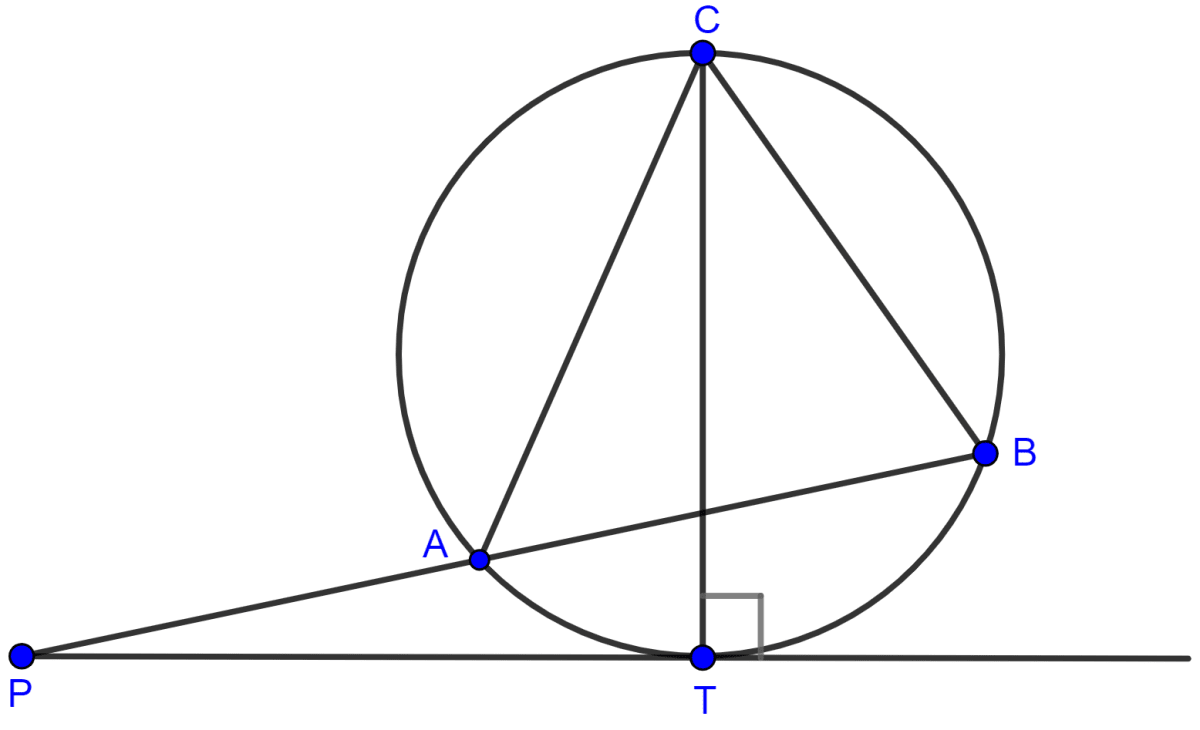
Answer
Join AT and BT.
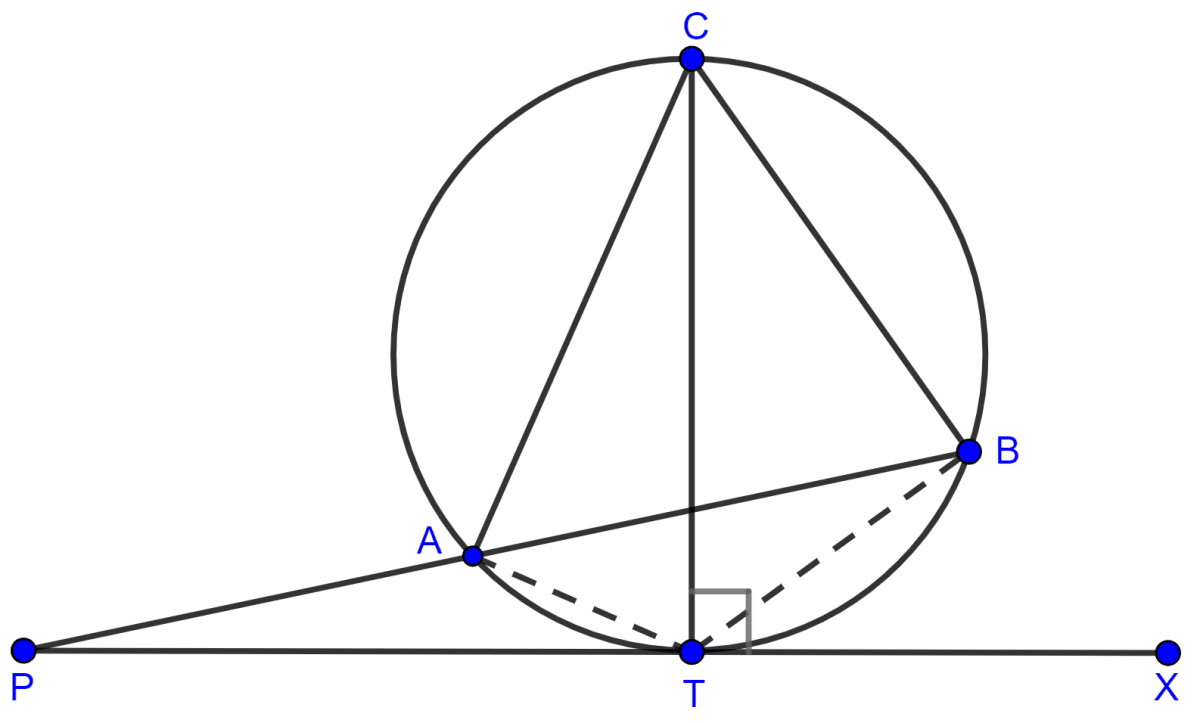
(i) TC is the diameter of the circle.
Since, angle in a semi-circle is a right angle.
∴ ∠CBT = 90°.
Hence, ∠CBT = 90°.
(ii) In cyclic quadrilateral ATBC,
⇒ ∠CBT + ∠CAT = 180° (∵ Sum of opposite angles of a cyclic quadrilateral = 180°)
⇒ 90° + ∠CAT = 180°
⇒ ∠CAT = 180° - 90°
⇒ ∠CAT = 90°.
In △ABC,
⇒ ∠CBA + ∠CAB + ∠ACB = 180° [By angle sum property of triangle]
⇒ 70° + ∠CAB + 50° = 180°
⇒ ∠CAB + 120° = 180°
⇒ ∠CAB = 180° - 120°
⇒ ∠CAB = 60°.
From figure,
∠BAT = ∠CAT - ∠CAB = 90° - 60° = 30°.
Hence, ∠BAT = 30°.
(iii) From figure,
∠BTX = ∠BAT = 30° [Angle in same segment are equal]
∠PBT = ∠CBT - ∠CBA = 90° - 70° = 20°.
⇒ ∠PTB = 180° - ∠BTX = 180° - 30° = 150°.
In △PBT,
⇒ ∠PBT + ∠PTB + ∠APT = 180° [By angle sum property of triangle]
⇒ 20° + 150° + ∠APT = 180°
⇒ ∠APT + 170° = 180°
⇒ ∠APT = 180° - 170°
⇒ ∠APT = 10°.
Hence, ∠APT = 10°.
Related Questions
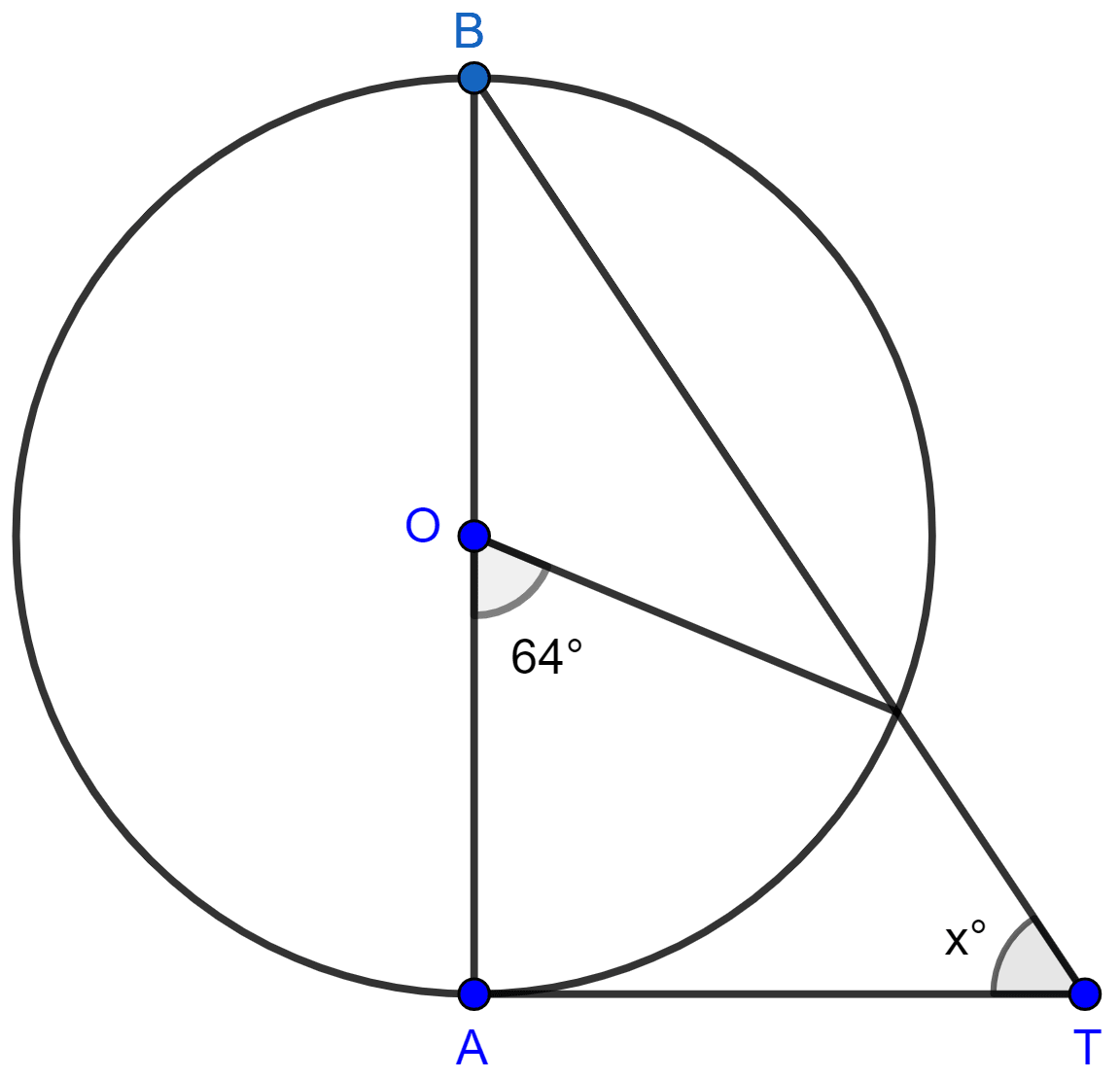
In the given figure, AB is the diameter of the circle, with center O, and AT is the tangent. Calculate the numerical value of x.
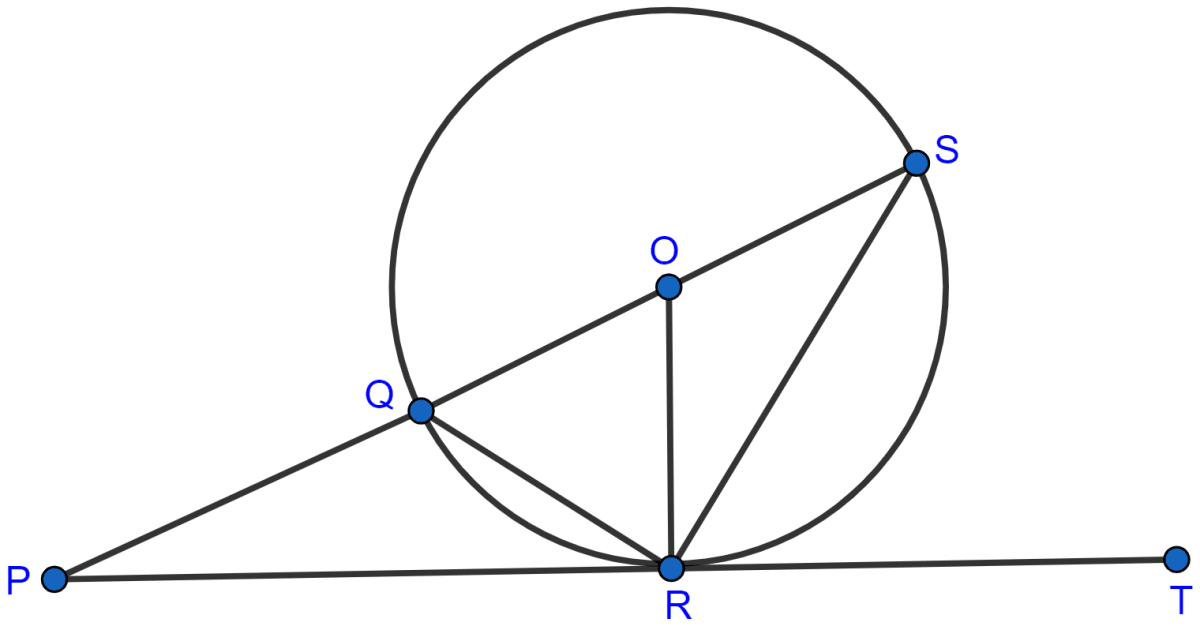
In the given figure, PT touches the circle with center O at point R. Diameter SQ is produced to meet the tangent TR at P.
Given ∠SPR = x° and ∠QRP = y°;
prove that :
(i) ∠ORS = y°
(ii) write an expression connecting x and y.
In the given figure, O is the center of the circumcircle ABC. Tangents A and C intersect at P. Given angle AOB = 140° and angle APC = 80°; find the angle BAC.

In the given figure, PQ is a tangent to the circle at A. AB and AD are bisectors of ∠CAQ and ∠PAC. If ∠BAQ = 30°, prove that : BD is diameter of the circle.