Points P(a, -4), Q(-2, b) and R(0, 2) are collinear. If Q lies between P and R, such that PR = 2QR, calculate the values of a and b.

From figure,

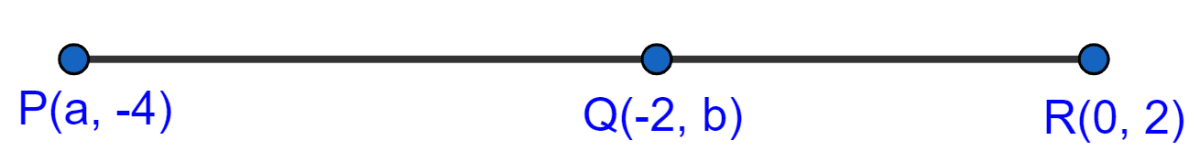
PR = PQ + QR

Given,

⇒ PR = 2QR

⇒ PQ +QR = 2QR

⇒ PQ = QR.

∴ Q is the mid-point of P and R.

By formula,

Mid-point = $\Big(\dfrac{x$1 + x2}{2}, \dfrac{y1 + y2}{2}\Big)

Substituting value we get,

$\Rightarrow Q = \Big(\dfrac{a + 0}{2}, \dfrac{-4 + 2}{2}\Big) \\[1em] \Rightarrow (-2, b) = \Big(\dfrac{a}{2}, \dfrac{-2}{2}\Big) \\[1em] \Rightarrow -2 = \dfrac{a}{2} \text{ and } b = \dfrac{-2}{2} \\[1em] \Rightarrow a = -4 \text{ and } b = -1.$

Hence, a = -4 and b = -1.