Mathematics
In the quadrilateral given below, AB || DC || EG. If E is mid-point of AD, prove that
(i) G is midpoint of BC
(ii) 2EG = AB + CD
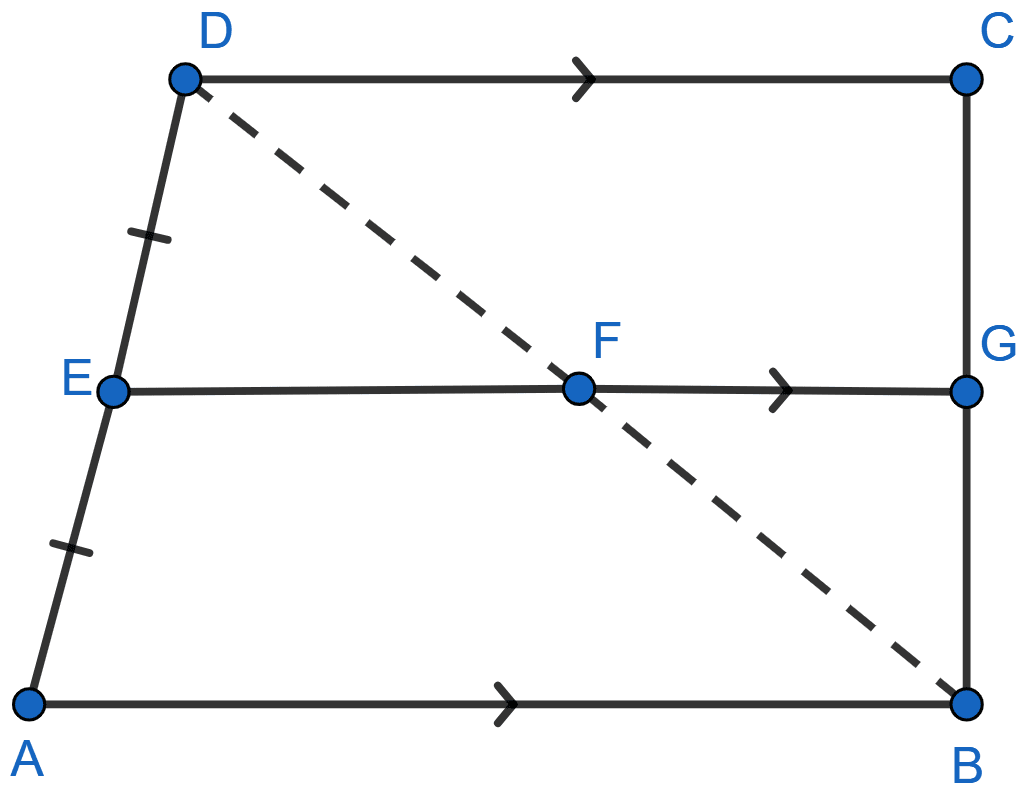
Mid-point Theorem
22 Likes
Answer
(i) Given,
EG || AB, we can say that
⇒ EF || AB
In △DAB,
E is midpoint of AD and EF || AB
∴ F is midpoint of BD (By converse of mid-point theorem).
EF = AB …….(1)
Given,
EG || DC we can say that,
FG || DC
In △BCD,
F is midpoint of BD and FG || DC
∴ G is midpoint of BC (By converse of mid-point theorem).
Hence, proved that G is midpoint of BC.
(ii) In △BCD,
F is midpoint of BD and G is midpoint of BC
∴ FG = DC …….(2)
Adding eqn. 1 from part (i) and eqn. 2 we get,
EF + FG = AB + DC
EG = (AB + CD)
2EG = AB + CD.
Hence, proved that 2EG = AB + CD.
Answered By
15 Likes
Related Questions
ABC is an isosceles triangle with AB = AC. D, E and F are mid-points of the sides BC, AB and AC respectively. Prove that line segment AD is perpendicular to EF and is bisected by it.
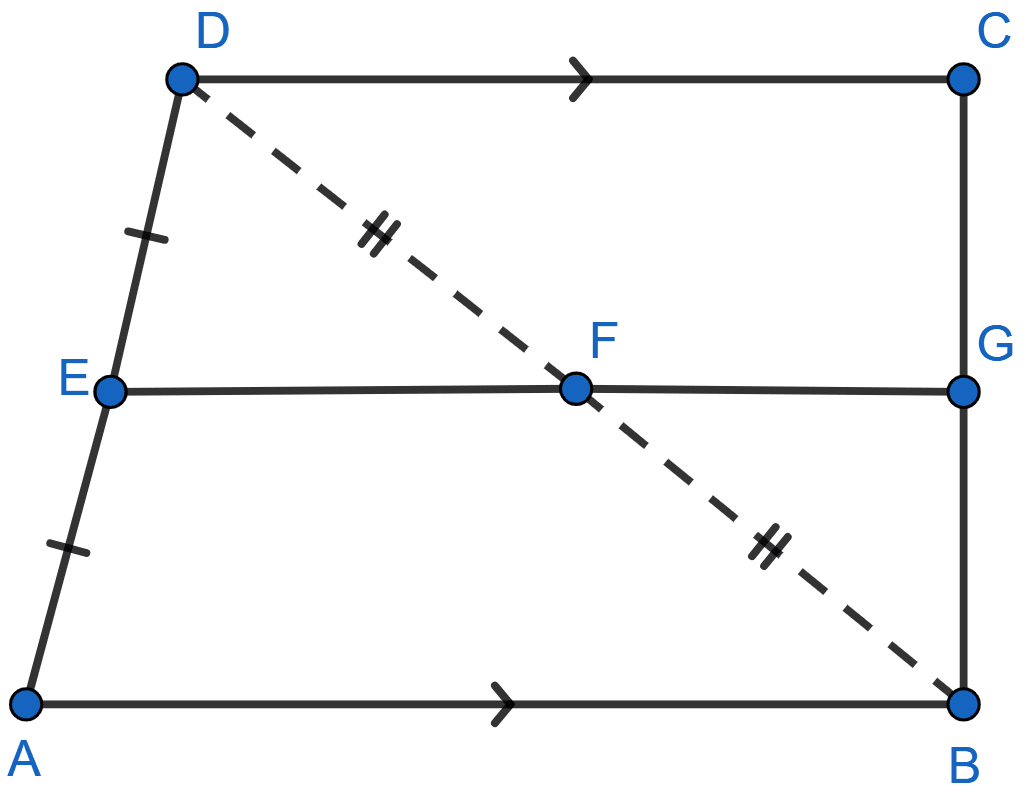
In the quadrilateral given below, AB || DC, E and F are mid-points of AD and BD respectively. Prove that
(i) G is the mid-point of BC
(ii) EG = (AB + DC).
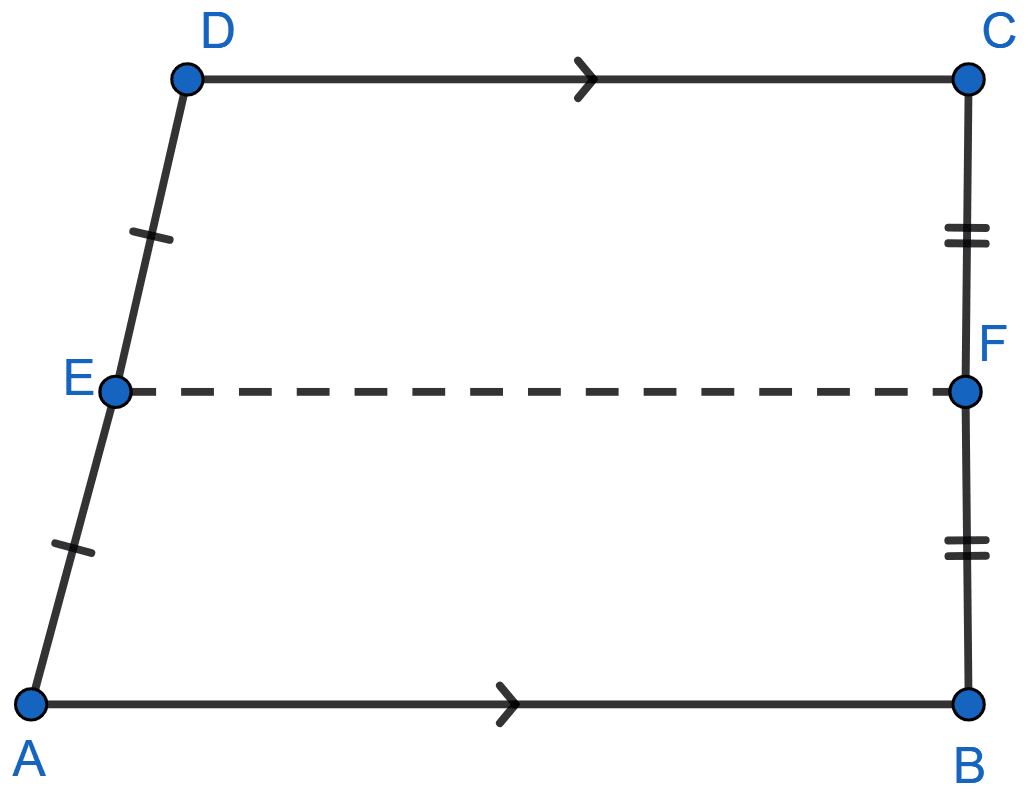
In the quadrilateral given below, AB || DC. E and F are mid-points of non-parallel sides AD and BC respectively. Calculate :
(i) EF if AB = 6 cm and DC = 4 cm
(ii) AB if DC = 8 cm and EF = 9 cm.
In the quadrilateral given below, AD = BC, P, Q, R and S are mid-points of AB, BD, CD and AC respectively. Prove that PQRS is a rhombus.
