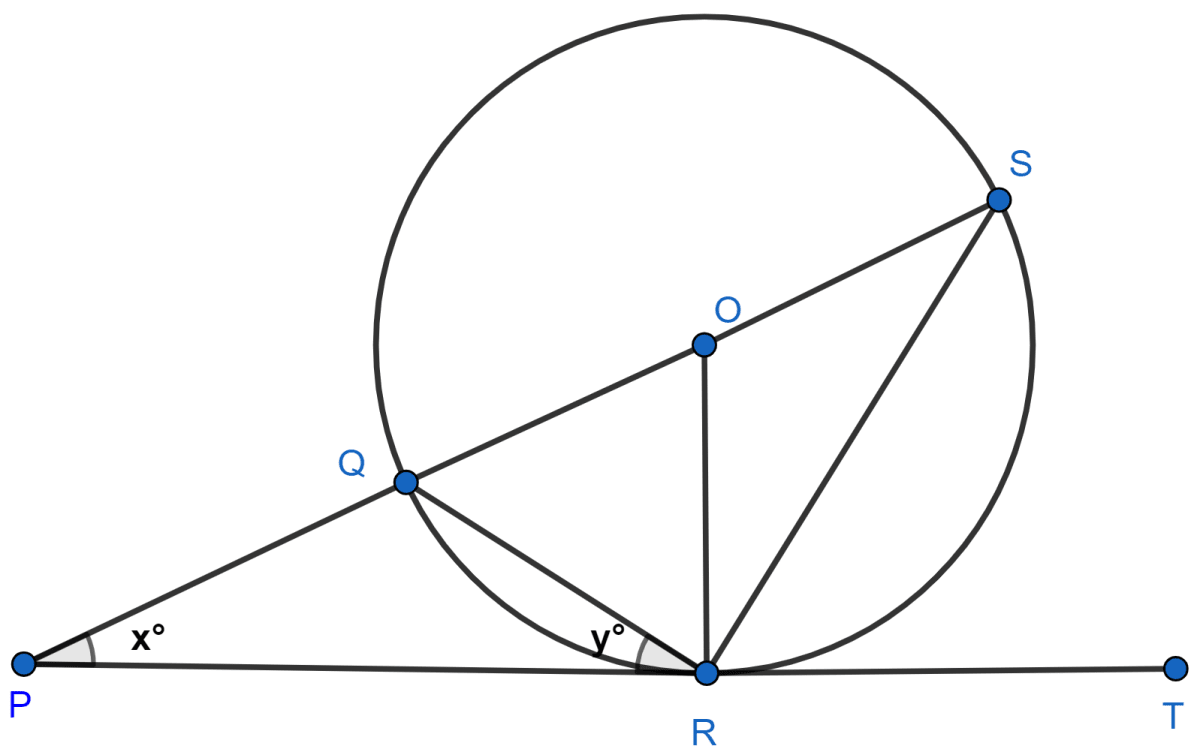
In the given figure, PT touches the circle, whose center is at point O, at point R. Diameter SQ produced meets tangent PT at point P. If angle SPR = x° and angle QRP = y°; find :

(i) angle ORQ in terms of y°.

(ii) a relation between x and y.

(i) Given,

∠QRP = y°

∠ORP = 90° (As radius from center to point of contact of tangent are perpendicular to each other)

From figure,

∠ORQ = ∠ORP - ∠QRP = 90° - y°.

Hence, ∠ORQ = 90° - y°.

(ii) From figure,

∠QRS = 90° (Angle in semi-circle is a right angle)

∠QRO + ∠ORS = ∠QRS

(90 - y)° + ∠ORS = 90°

∠ORS = 90° - (90 - y)°

∠ORS = y°

We know that,

Angles opposite to equal sides are equal.

∠ORS = ∠OSR = y° [∵ OR = OS radius of same circle]

We know that,

Angle subtended at the center by an arc is twice the angle subtended by it on any other part of circumference.

⇒ ∠QOR = 2∠QSR

⇒ ∠QOR = 2∠OSR = 2y°

We know that,

An exterior angle of the triangle is equal to the sum of two opposite interior angles.

⇒ ∠OQR = ∠QPR + ∠QRP

⇒ ∠OQR = x° + y°

⇒ ∠ORQ = ∠OQR = x° + y° [∵ OR = OQ radius of same circle]

In △OQR,

⇒ ∠OQR + ∠ORQ + ∠QOR = 180° (By angle sum property of triangle)

⇒ x° + y° + x° + y° + 2y° = 180°

⇒ 2x° + 4y° = 180°

⇒ 2(x° + 2y°) = 180°

⇒ x° + 2y° = 90°

Hence, equation connecting x and y is x° + 2y° = 90°.