Mathematics
If the mean of following distribution is . Find the missing frequency p.
| C.I. | f |
|---|---|
| 25-35 | 10 |
| 35-45 | 6 |
| 45-55 | 4 |
| 55-65 | p |
| 65-75 | 4 |
| 75-85 | 12 |
| 85-95 | 26 |
Statistics
3 Likes
Answer
| C.I. | f | Class mark (x) | fx |
|---|---|---|---|
| 25-35 | 10 | 30 | 300 |
| 35-45 | 6 | 40 | 240 |
| 45-55 | 4 | 50 | 200 |
| 55-65 | p | 60 | 60p |
| 65-75 | 4 | 70 | 280 |
| 75-85 | 12 | 80 | 960 |
| 85-95 | 26 | 90 | 2340 |
| Total | Σf = 62 + p | Σfx = 4320 + 60p |
By formula,
Mean =
Given,
Mean =
Hence, p = 10.
Answered By
1 Like
Related Questions
A solid, in the form of a right circular cone, is mounted on a hemisphere of the same radius. The radius of the hemisphere is 2.1 cm and the height of the cone is 4 cm. The solid is placed in a cylindrical tub full of water in such a way that the whole solid is submerged in water. If the radius of the tub is 5 cm and its height is 9.8 cm, find the volume of water left in the tub.
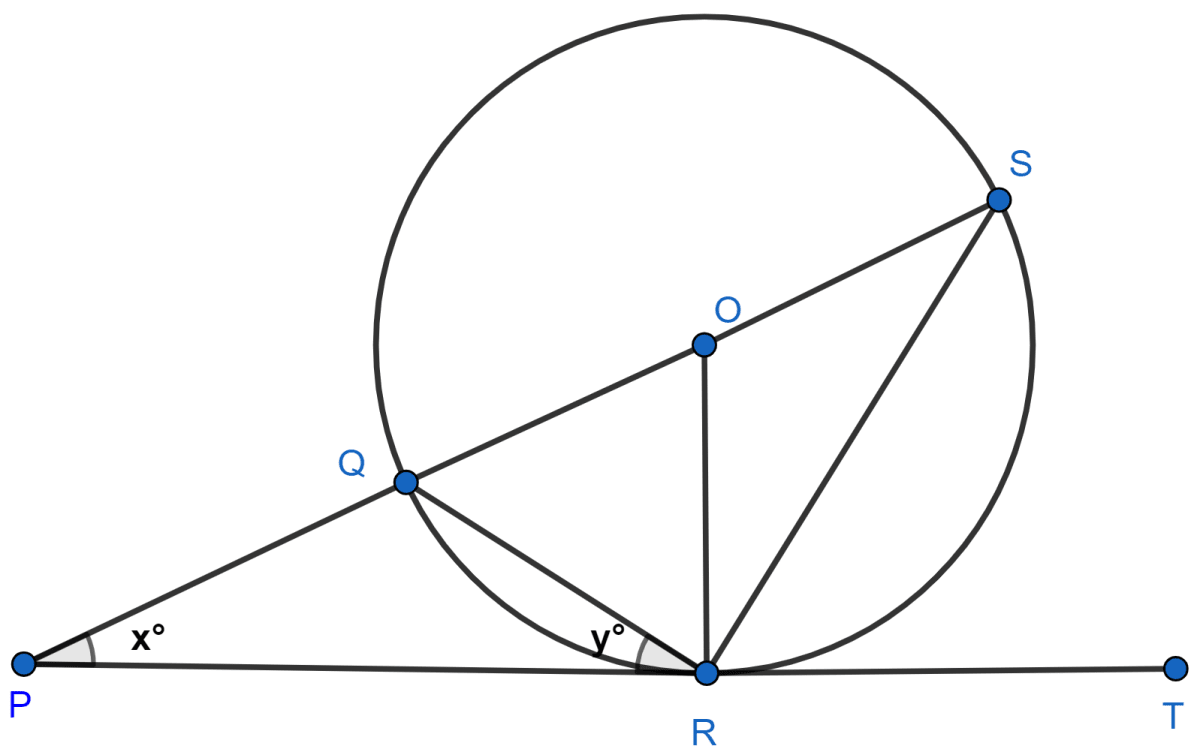
In the given figure, PT touches the circle, whose center is at point O, at point R. Diameter SQ produced meets tangent PT at point P. If angle SPR = x° and angle QRP = y°; find :
(i) angle ORQ in terms of y°.
(ii) a relation between x and y.
Solve the inequation 3 ≥ , x ∈ I (integers). Graph the solution on a real number line.
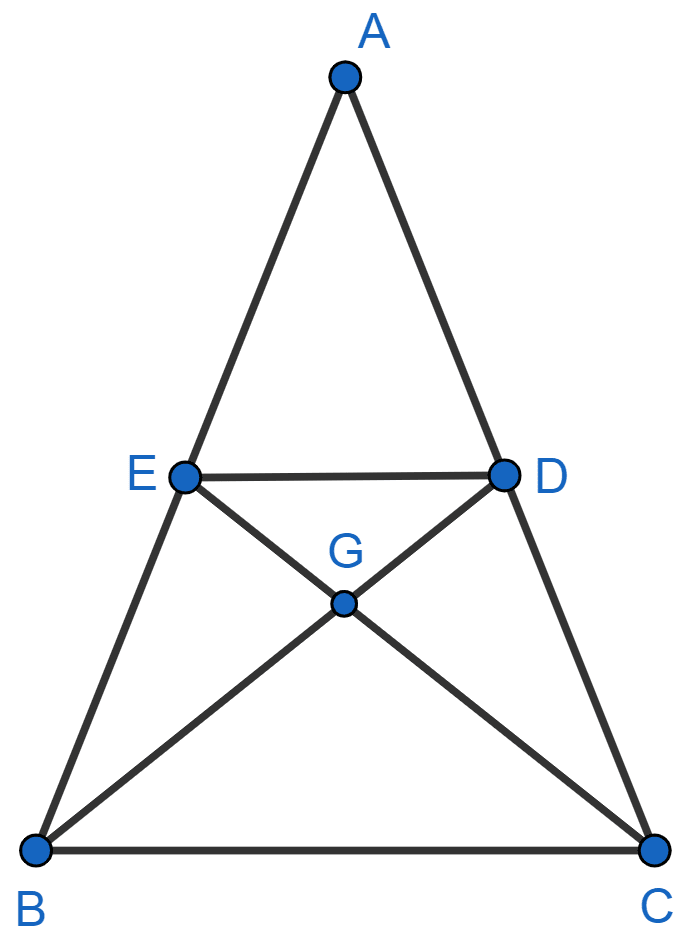
In the given figure, the median BD and CE of triangle ABC meet at point G. Show that BG = 2GD.