Mathematics
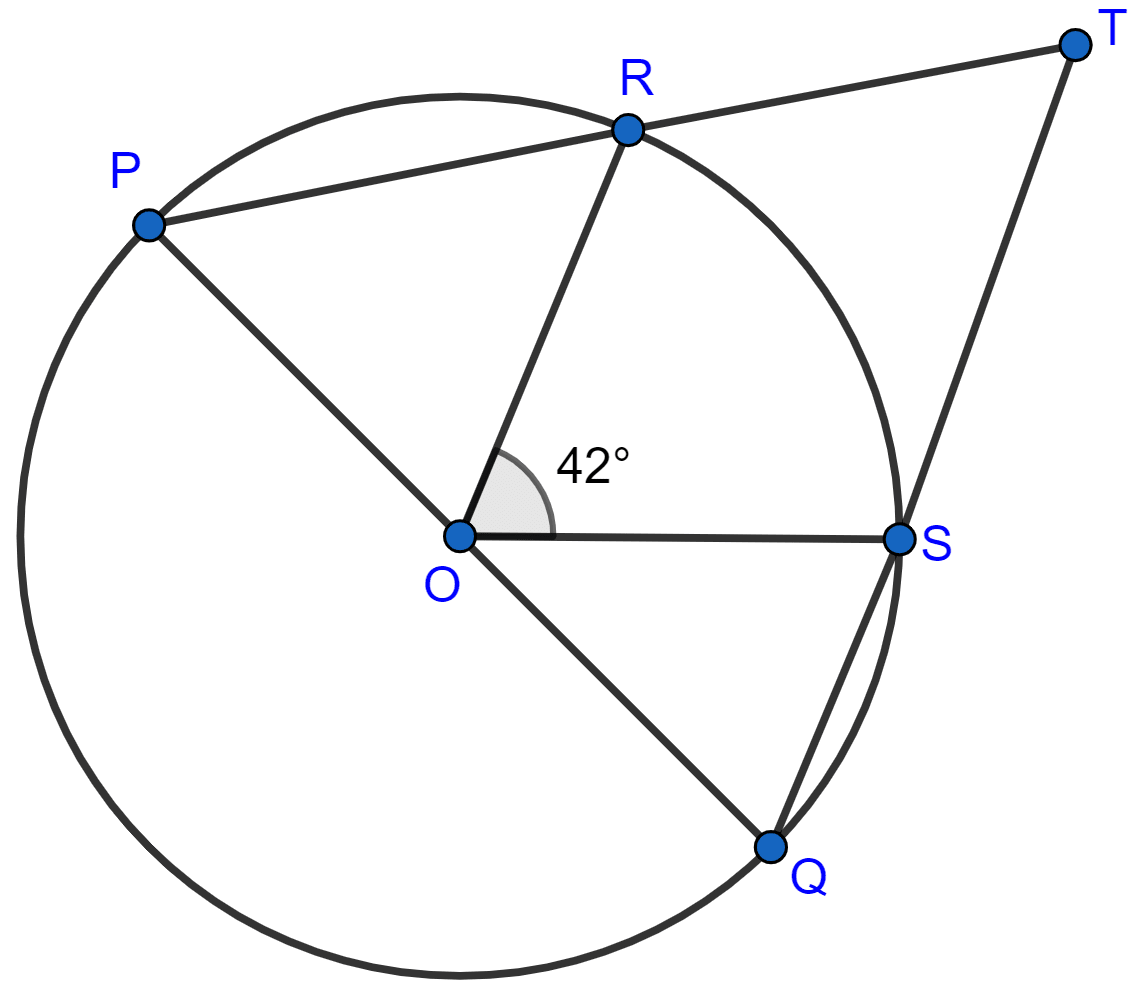
In the given figure, PQ is the diameter of the circle whose center is O. Given, ∠ROS = 42°, calculate ∠RTS.
Circles
32 Likes
Answer
Join PS.
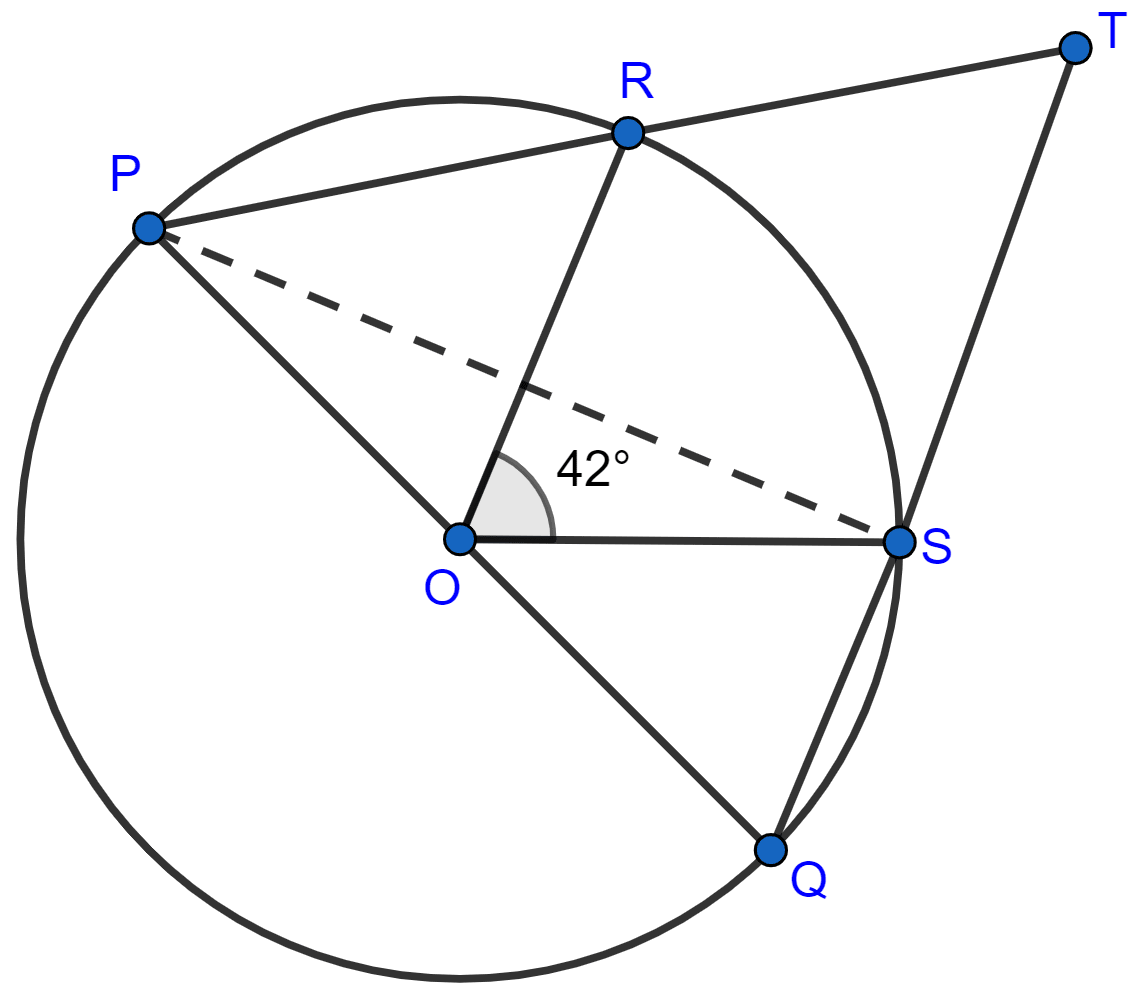
∠PSQ = 90° [Angle in semi-circle is a right angle.]
We know that,
Angle at the centre is double the angle at the circumference subtended by the same chord.
⇒ ∠ROS = 2∠SPR
⇒ ∠SPR = ∠ROS = = 21°
From figure,
⇒ ∠SPT = ∠SPR = 21°.
From figure,
⇒ ∠PSQ = 90° [Angle in a semi-circle is a right angle.]
Since, QST is a straight line.
⇒ ∠PSQ + ∠PST = 180°
⇒ 90° + ∠PST = 180°
⇒ ∠PST = 90°.
In △PST,
⇒ ∠PTS + ∠PST + ∠SPT = 180° [Angle sum property of triangle]
⇒ ∠PTS + 90° + 21° = 180°
⇒ ∠PTS + 111° = 180°
⇒ ∠PTS = 180° - 111° = 69°.
From figure,
∠RTS = ∠PTS = 69°.
Hence, ∠RTS = 69°.
Answered By
19 Likes
Related Questions
In the given figure, AC is the diameter of circle, centre O. Chord BD is perpendicular to AC. Write down the angles p, q and r in terms of x.

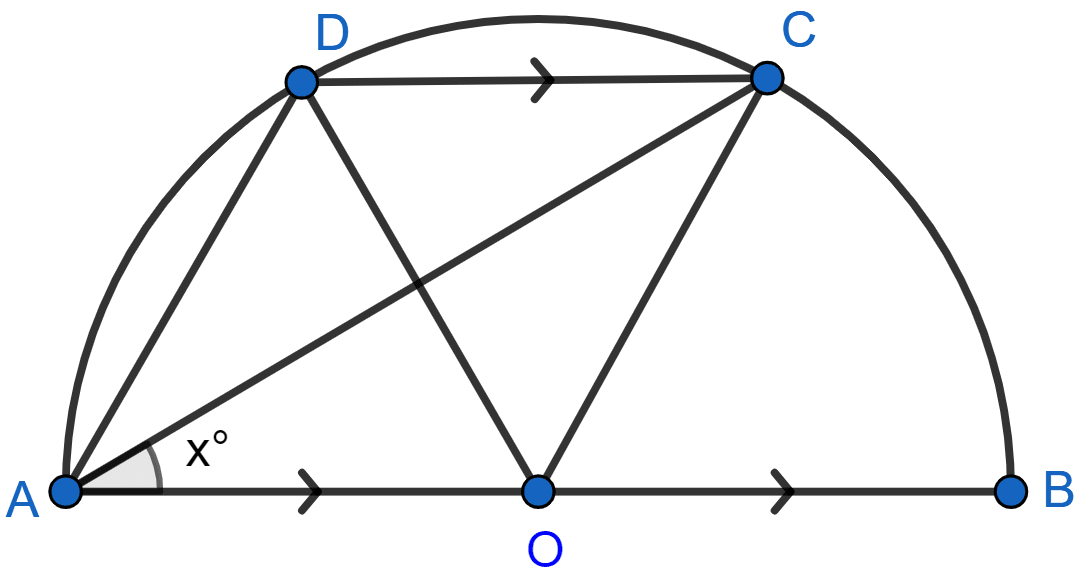
In the given figure, AOB is a diameter and DC is parallel to AB. If ∠CAB = x°; find (in terms of x) the values of :
(i) ∠COB,
(ii) ∠DOC,
(iii) ∠DAC,
(iv) ∠ADC.
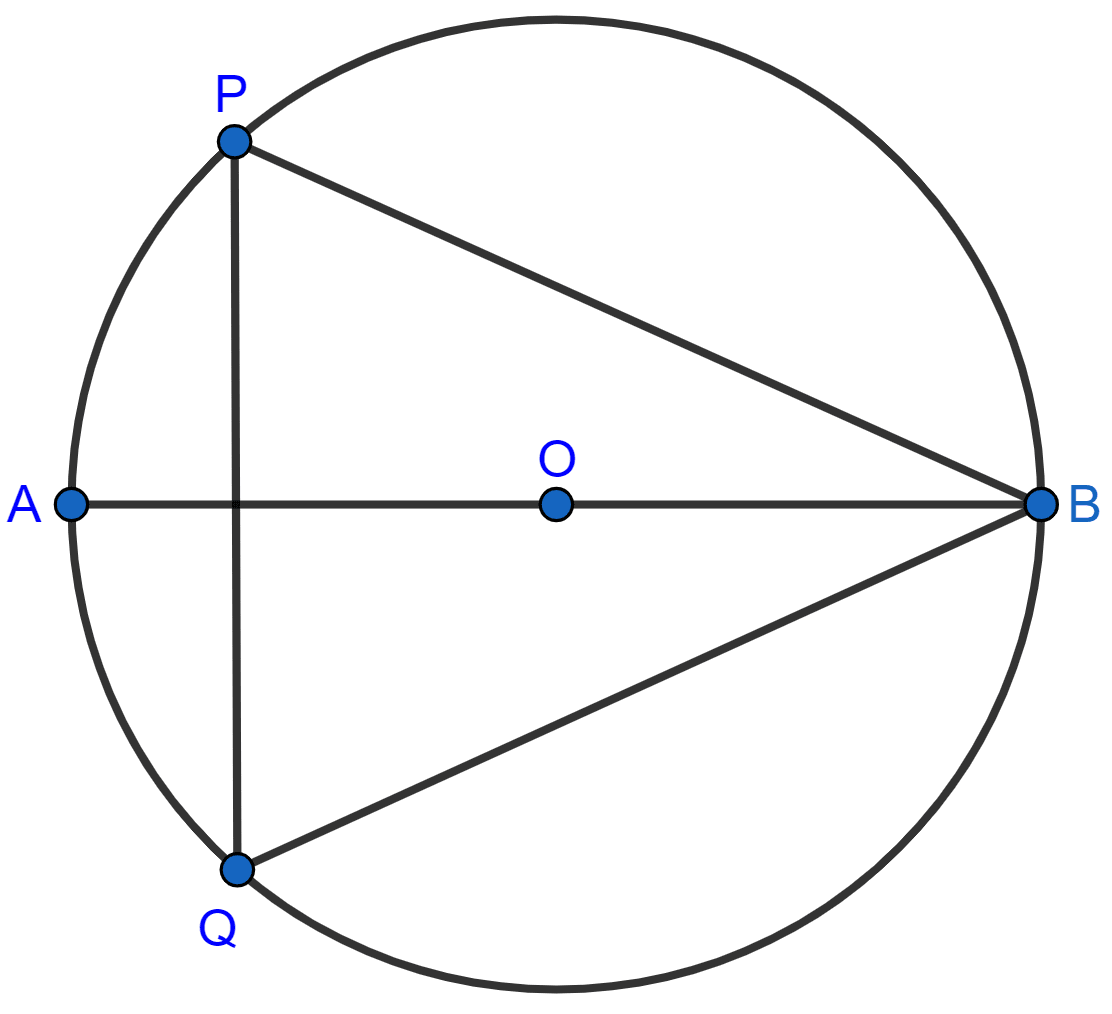
The given figure shows a circle with center O and ∠ABP = 42°. Calculate the measure of :
(i) ∠PQB
(ii) ∠QPB + ∠PBQ
In the given figure, M is the centre of the circle. Chords AB and CD are perpendicular to each other. If ∠MAD = x and ∠BAC = y :
(i) express ∠AMD in terms of x.
(ii) express ∠ABD in terms of y.
(iii) prove that : x = y.
