Mathematics
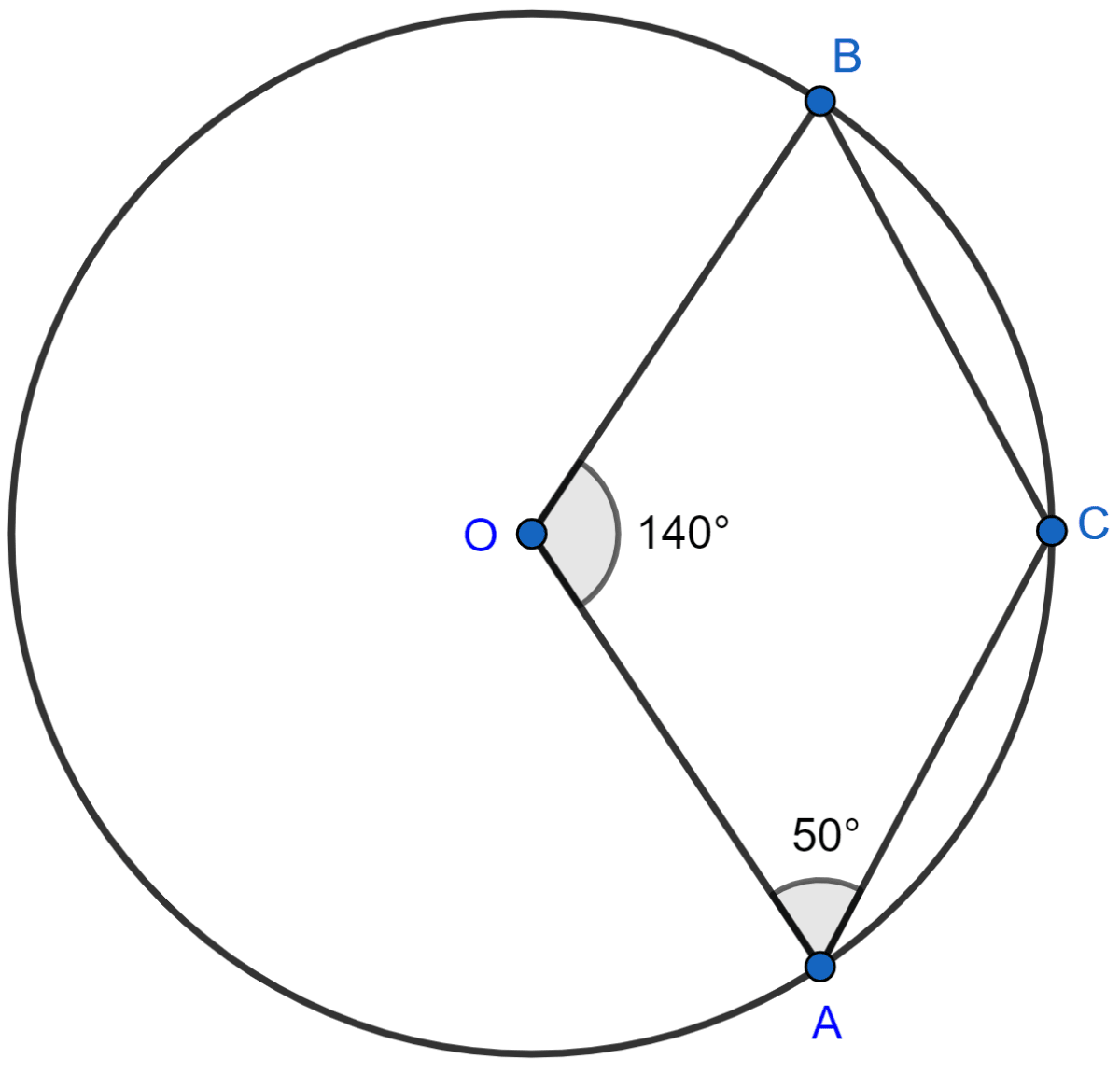
In the given figure, O is the centre of the circle. If ∠AOB = 140° and ∠OAC = 50°; find :
(i) ∠ACB,
(ii) ∠OBC,
(iii) ∠OAB,
(iv) ∠CBA.
Circles
52 Likes
Answer
Given, ∠AOB = 140° and ∠OAC = 50°
(i) We know that,
Angle at the center is double the angle at the circumference subtended by the same chord.
∠ACB = Reflex ∠AOB
= (360° - 140°)
= = 110°.
Hence, ∠ACB = 110°.
(ii) We know that,
The sum of angles in a quadrilateral is 360°
In quadrilateral OBCA,
∠OBC + ∠ACB + ∠OAC + ∠AOB = 360°
⇒ ∠OBC + 110° + 50° + 140° = 360°
⇒ ∠OBC + 300° = 360°
⇒ ∠OBC = 360° - 300° = 60°.
Hence, ∠OBC = 60°.
(iii) Join AB.
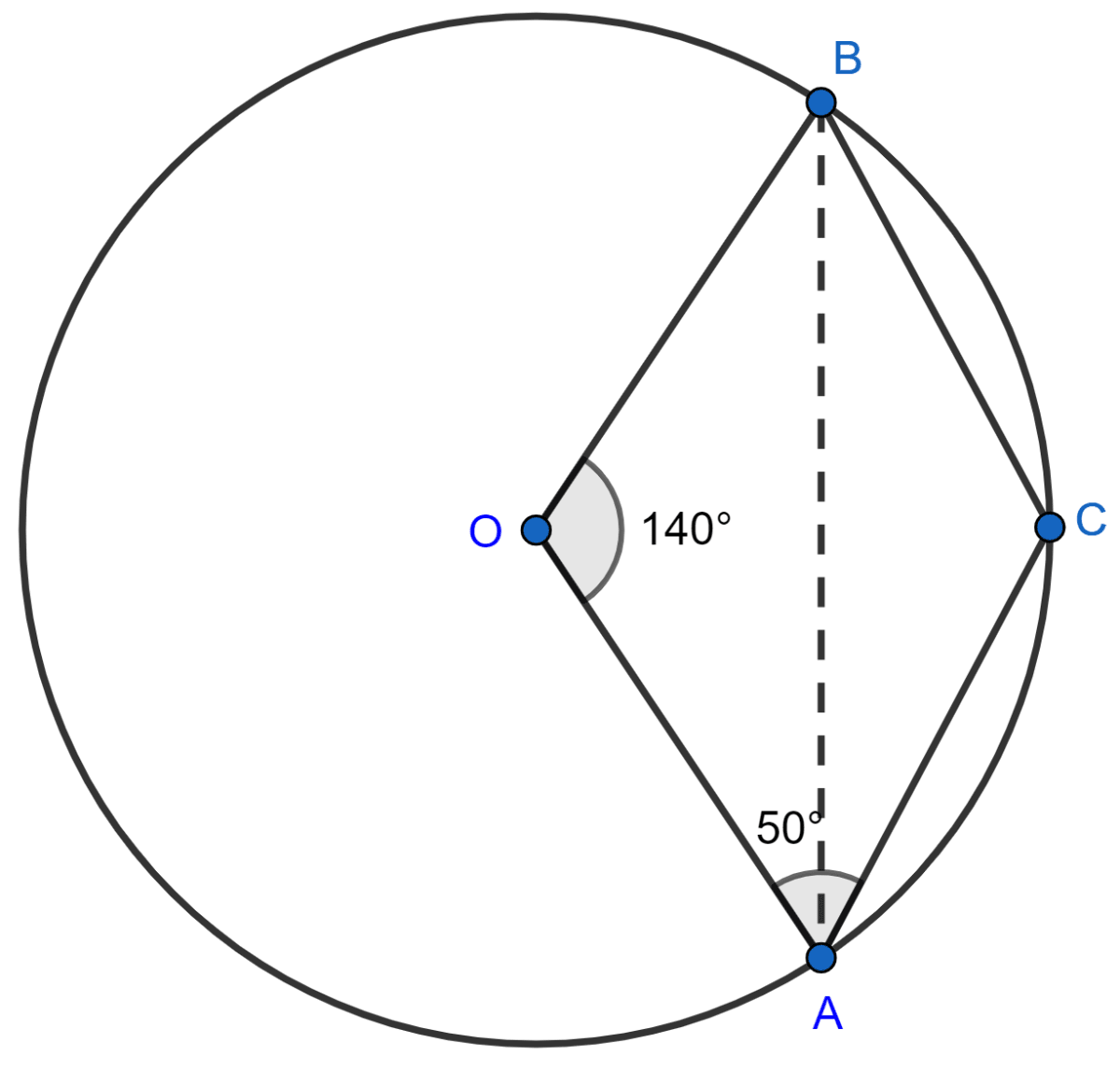
In ∆AOB, we have
OA = OB (radius of circle)
So, ∠OBA = ∠OAB (As angles opposite to equal sides are equal)
By angle sum property of a triangle we get,
⇒ ∠OBA + ∠OAB + ∠AOB = 180°
⇒ 2∠OAB + 140° = 180°
⇒ 2∠OAB = 40°
⇒ ∠OAB = = 20°
Hence, ∠OAB = 20°.
(iv) We already found, ∠OBC = 60°.
⇒ ∠OBC = ∠CBA + ∠OBA
⇒ 60° = ∠CBA + 20°
⇒ ∠CBA = 60° - 20° = 40°
Hence, ∠CBA = 40°.
Answered By
31 Likes
Related Questions
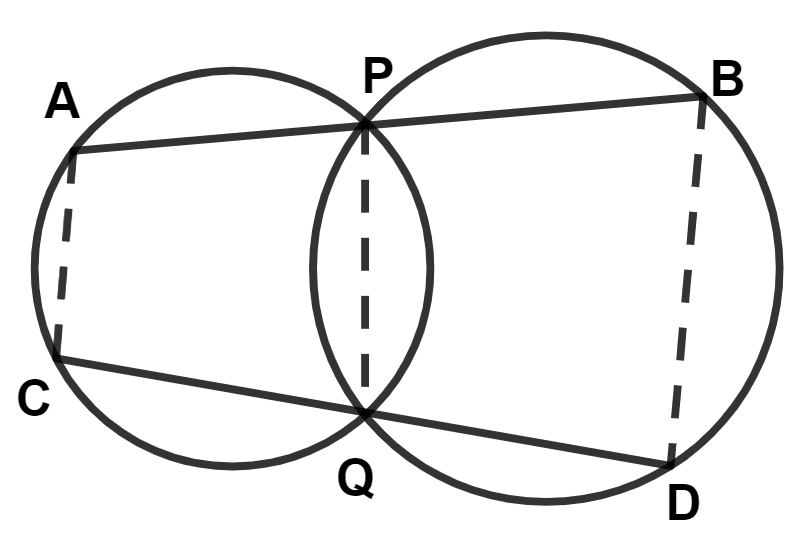
In the given figure APB and CQD are two straight lines, then :
AB || CD
AC || PQ
PQ || BD
AC || BD
In the figure, given below, ∠ABC is equal to :
105°
75°
90°
45°

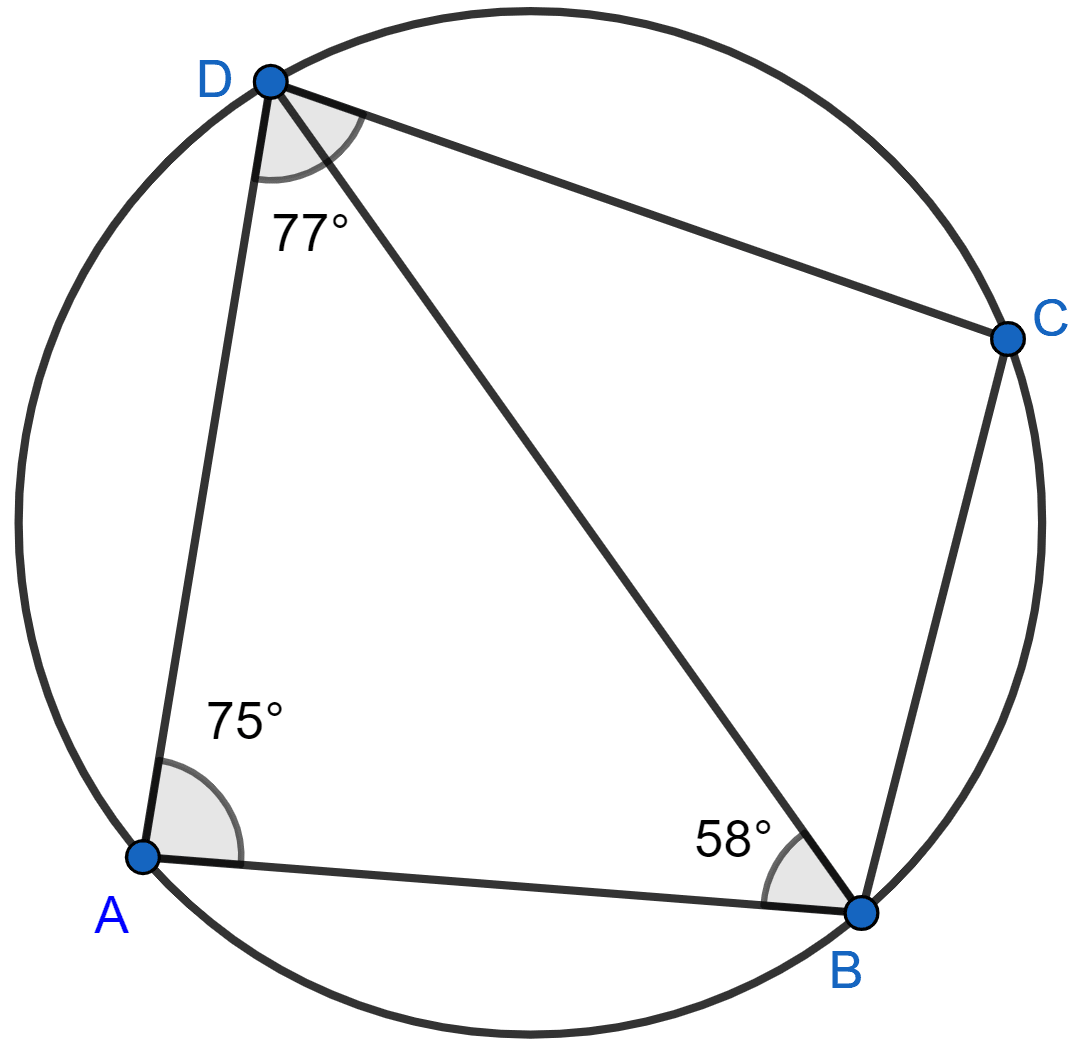
In the figure, given below, ABCD is a cyclic quadrilateral in which ∠BAD = 75°; ∠ABD = 58° and ∠ADC = 77°. Find :
(i) ∠BDC,
(ii) ∠BCD,
(iii) ∠BCA.
In the following figure, O is the centre of the circle and ΔABC is equilateral.
Find:
(i) ∠ADB, (ii) ∠AEB.