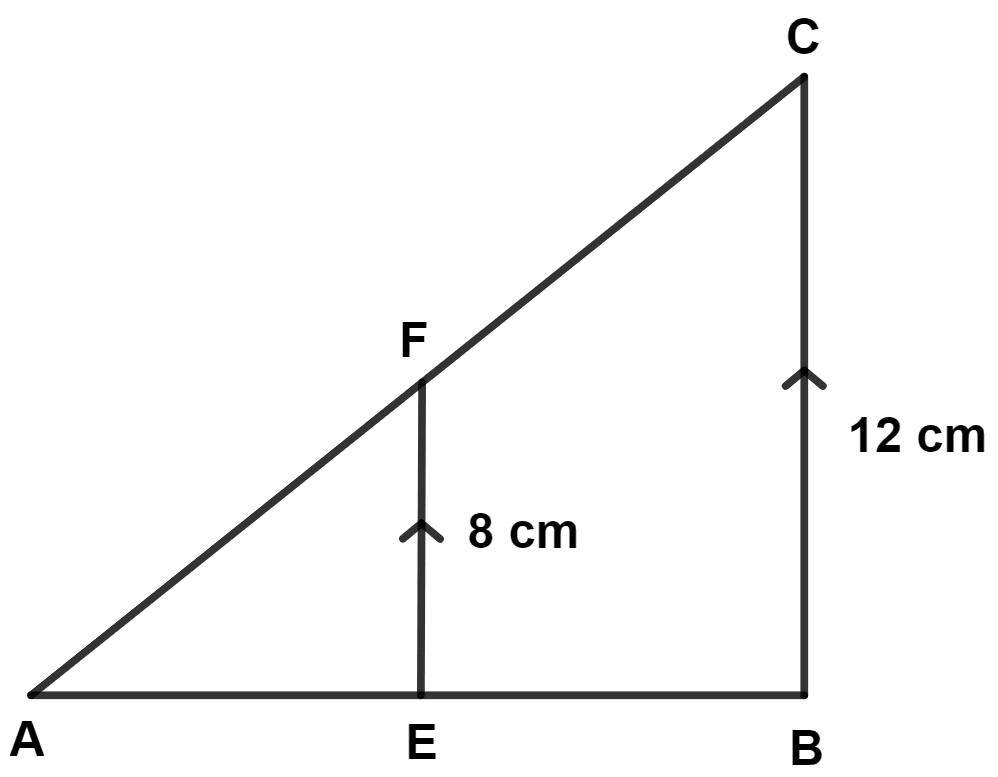
In the given figure, EF is parallel to BC. If area of triangle ABC is 576 cm², the area of triangle AEF is :

1. 128 cm²

2. 288 cm²

3. 256 cm²

4. 768 cm²

From figure,

In △ AEF and △ ABC,

⇒ ∠EAF = ∠BAC (Common angle)

⇒ ∠AEF = ∠ABC (Corresponding angle)

∴ △ AEF ~ △ ABC (By A.A. axiom)

We know that,

The areas of two similar triangles are proportional to the squares of their corresponding sides.

$\therefore \dfrac{\text{Area of △ AEF}}{\text{Area of △ ABC}} = \dfrac{EF^2}{BC^2} \\[1em] \Rightarrow \dfrac{\text{Area of △ AEF}}{576} = \dfrac{8^2}{12^2} \\[1em] \Rightarrow \dfrac{\text{Area of △ AEF}}{576} = \dfrac{64}{144} \\[1em] \Rightarrow \text{Area of △ AEF} = \dfrac{64}{144} \times 576 \\[1em] \Rightarrow \text{Area of △ AEF} = 64 \times 4 = 256 \text{ cm}^2.$

Hence, Option 3 is the correct option.