Mathematics
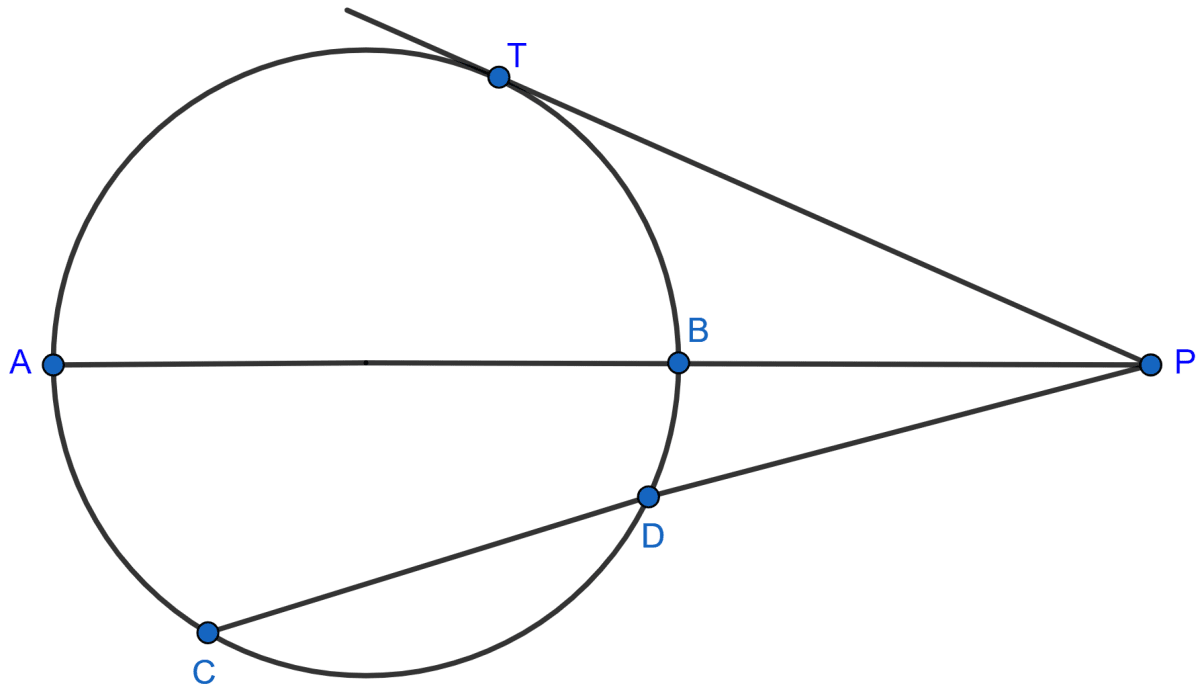
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find :
(i) AB
(ii) the length of tangent PT.
Circles
6 Likes
Answer
(i) From figure,
PC = PD + CD = 5 + 7.8 = 12.8 cm.
We know that,
If two chords of a circle intersect internally of externally then the product of the lengths of their segments is equal.
Since, here AB and CD intersect externally at P.
∴ AP x PB = CP x PD
⇒ AP x 4 = 12.8 x 5
⇒ AP = = 16 cm.
From figure,
AB = PA - PB = 16 - 4 = 12 cm.
Hence, AB = 12 cm.
(ii) We know that,
If a chord and a tangent intersect externally, then the product of lengths of the segments of the chord is equal to the square of the length of the tangent from the point of contact to the point of intersection.
∴ PA x PB = PT2
⇒ 16 x 4 = PT2
⇒ PT = = 8 cm.
Hence, PT = 8 cm.
Answered By
4 Likes
Related Questions
In the given circle, PA is tangent and PBC is secant, PA = 8 cm and PB = 4 cm. The length of BC is :
8 cm
12 cm
16 cm
2 cm

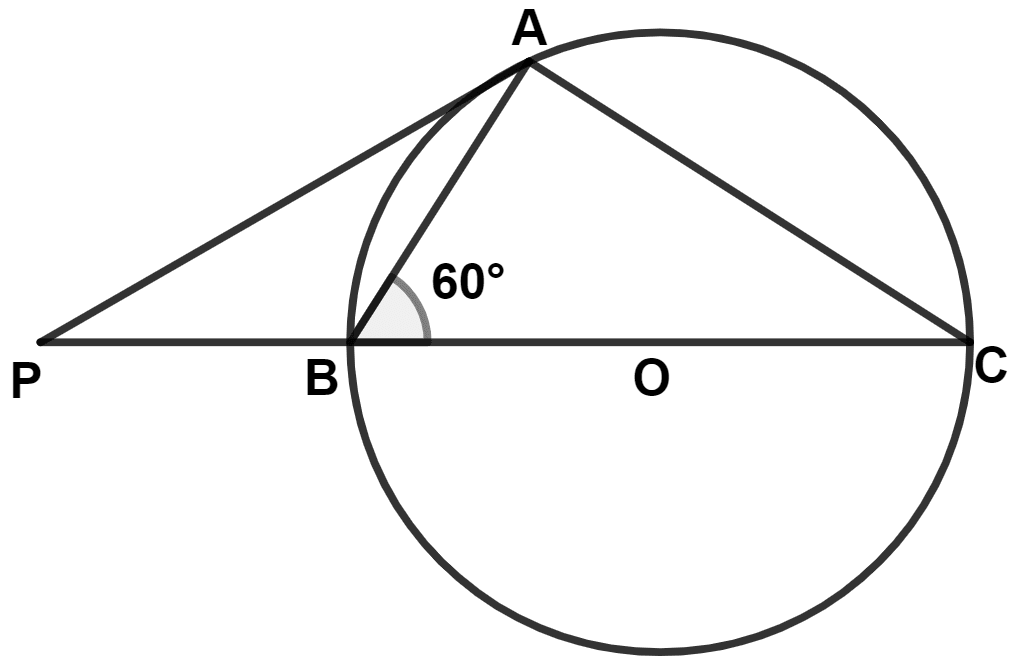
In the given figure, O is the center of the circle, PA is tangent and PBC is secant. If ∠ABC = 60°; ∠P is :
30°
60°
120°
90°
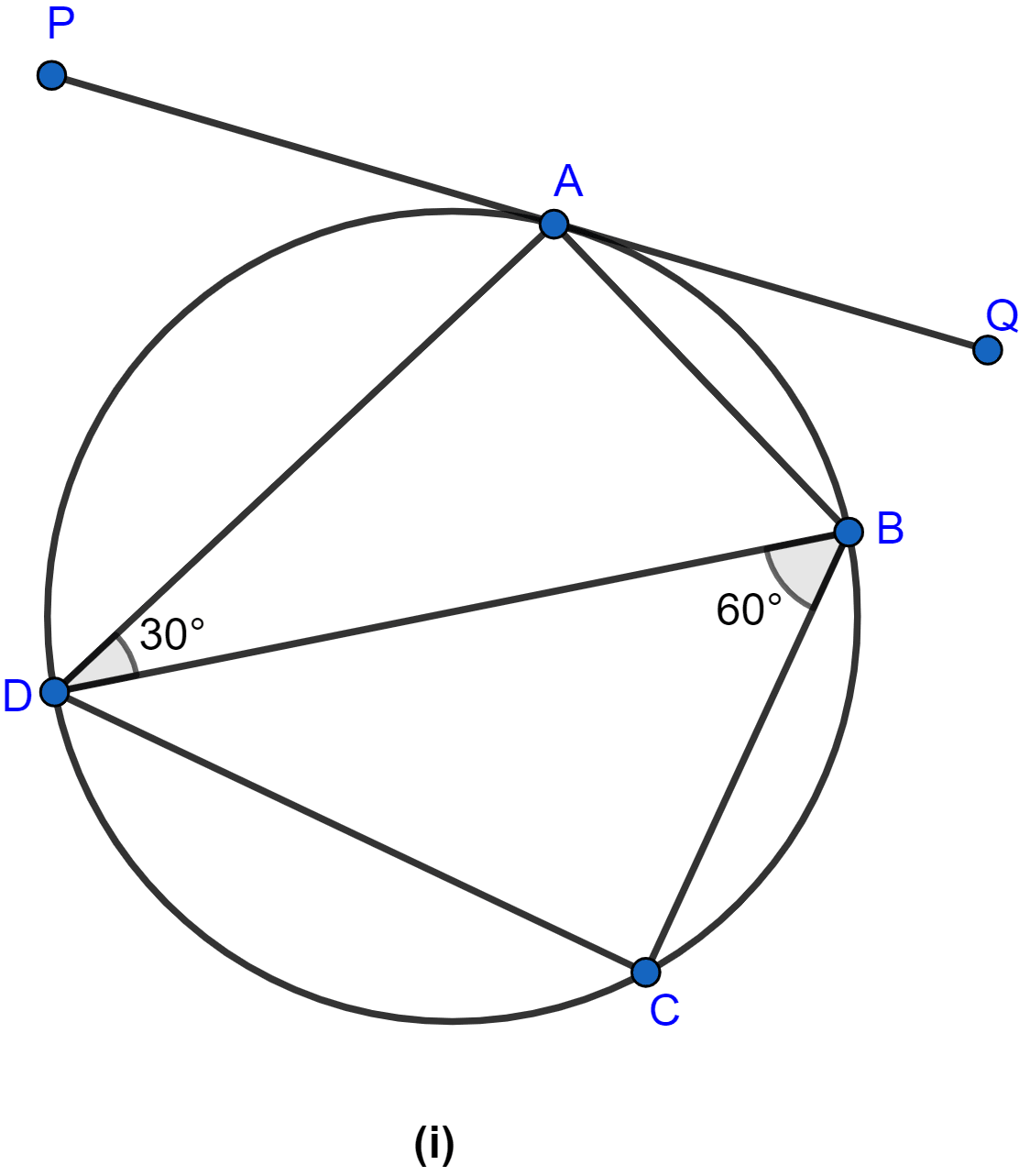
In the figure (i) given below, PQ is a tangent to the circle at A, DB is a diameter, ∠ADB = 30° and ∠CBD = 60°, calculate
(i) ∠QAB
(ii) ∠PAD
(iii) ∠CDB.
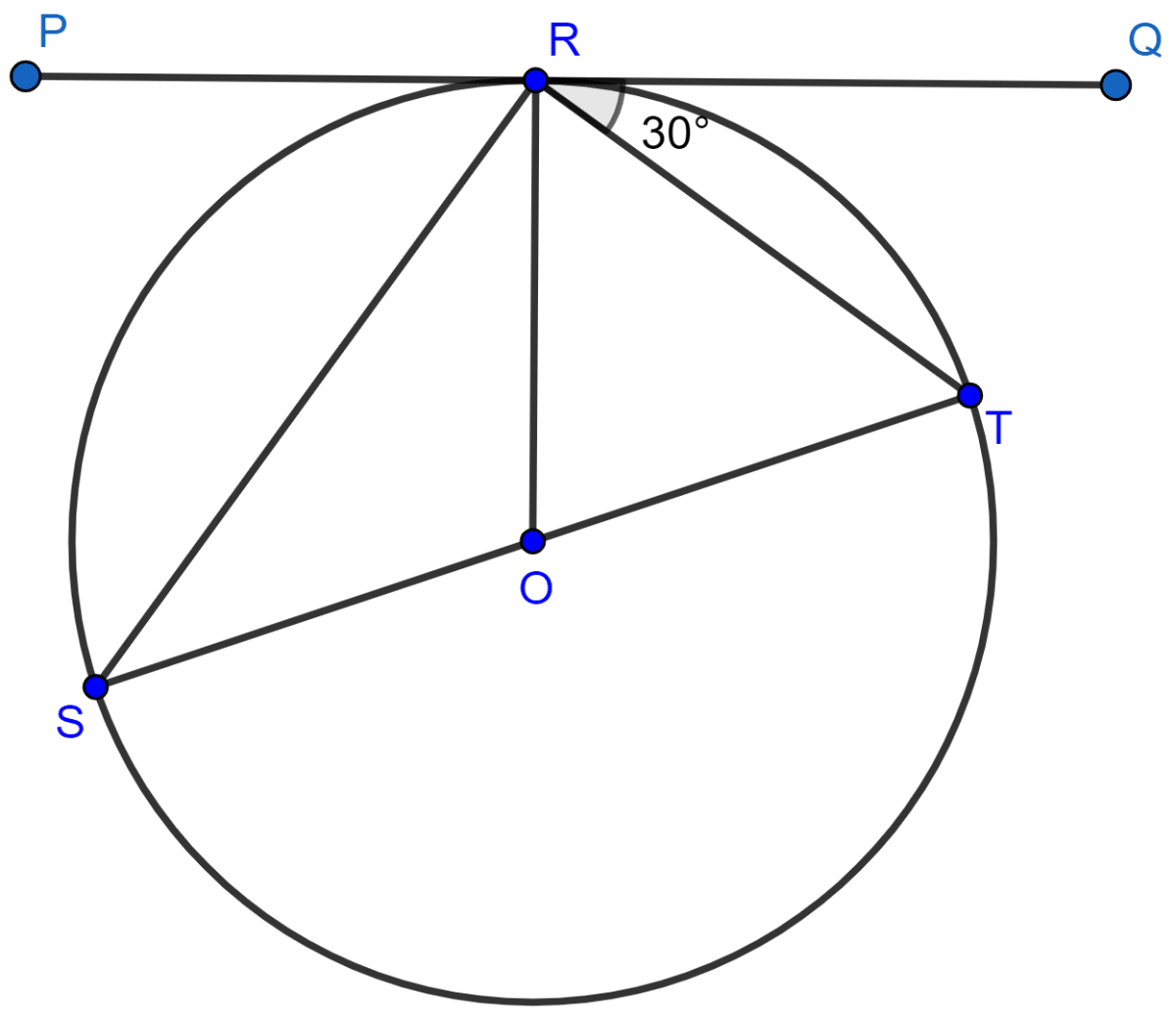
If PQ is a tangent to the circle at R; calculate :
(i) ∠PRS,
(ii) ∠ROT.
Given, O is the center of the circle and angle TRQ = 30°.