Mathematics
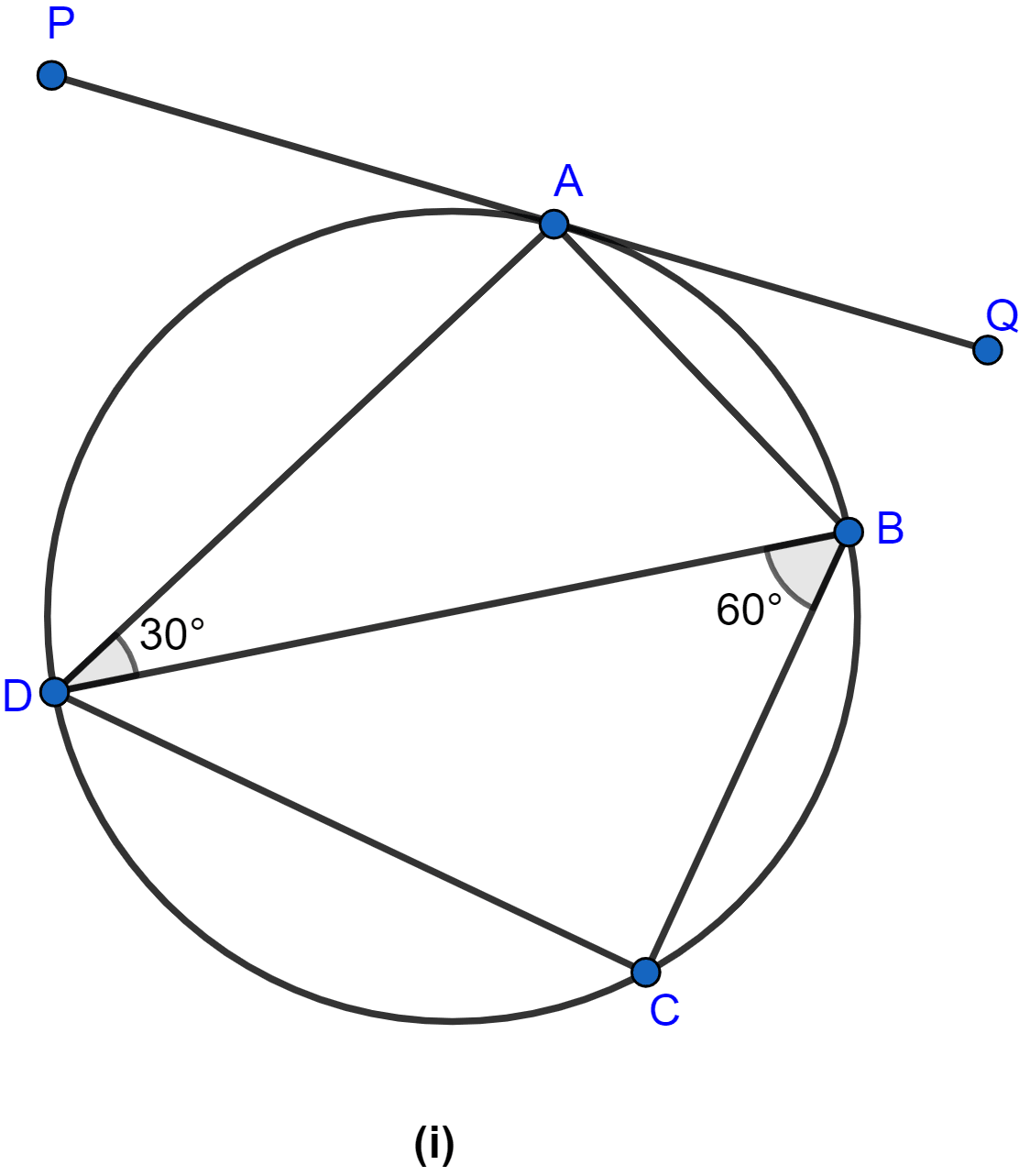
In the figure (i) given below, PQ is a tangent to the circle at A, DB is a diameter, ∠ADB = 30° and ∠CBD = 60°, calculate
(i) ∠QAB
(ii) ∠PAD
(iii) ∠CDB.
Circles
17 Likes
Answer
(i) ∠QAB = ∠ADB [∵ angles in alternate segment are equal.]
∴ ∠QAB = 30°.
Hence, the value of ∠QAB = 30°.
(ii) In △DAO,
OA = OD [∵ radii of the same circle]
So, ∠OAD = ∠ODA = 30° [∵ angles opposite to equal sides are equal.]
We know that,
The tangent at any point of a circle is perpendicular to the radius through the point of contact.
∴ ∠OAP = 90°.
From figure,
⇒ ∠PAD = ∠OAP - ∠OAD = 90° - 30° = 60°.
Hence, ∠PAD = 60°.
(iii) In △BCD,
∠BCD = 90° [∵ angle in a semi-circle is a right angle.]
∠CBD = 60°
∠CDB + ∠CBD + ∠BCD = 180° [By angle sum property of triangle]
⇒ ∠CDB + 60° + 90° = 180°
⇒ ∠CDB = 180° - 150° = 30°.
Hence, ∠CDB = 30°.
Answered By
9 Likes
Related Questions
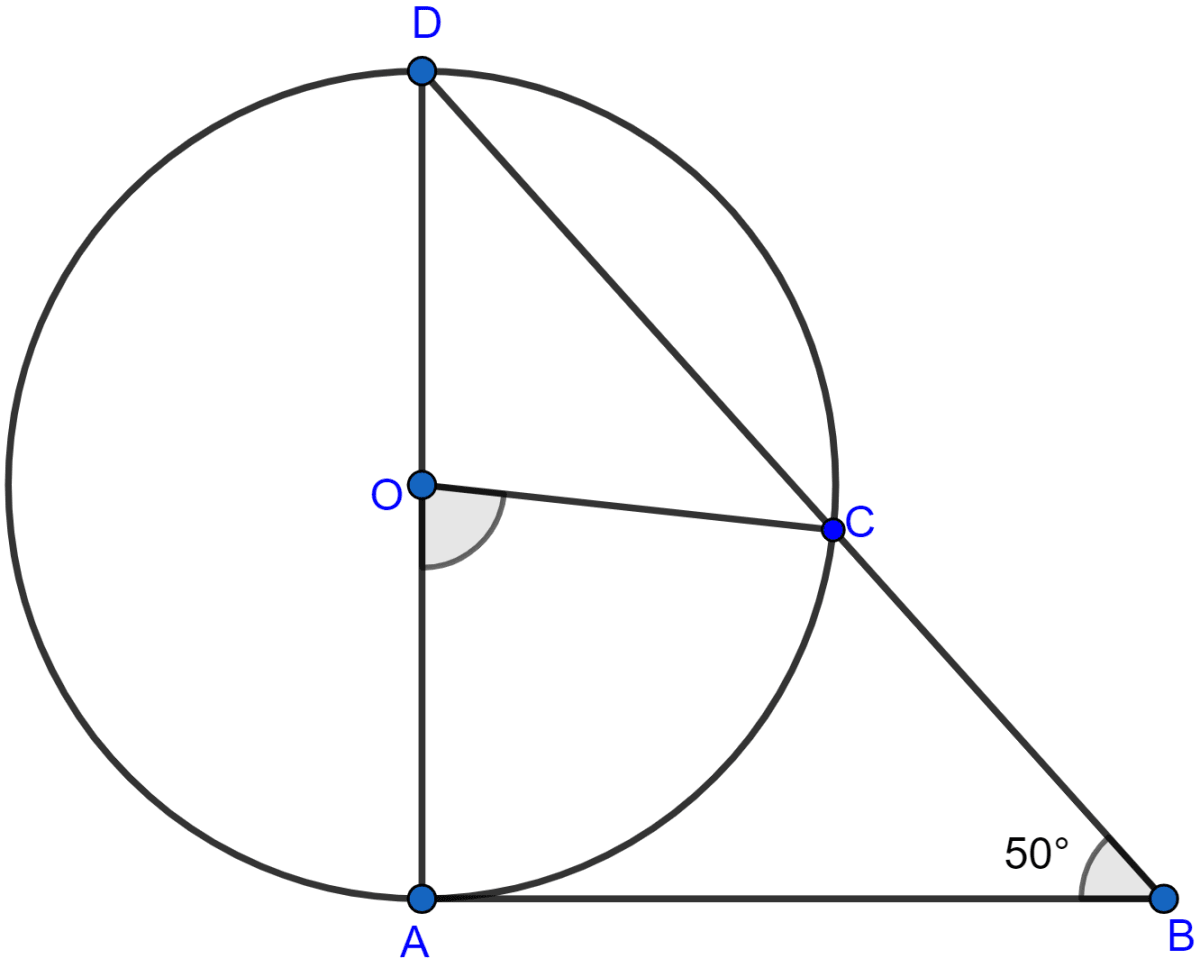
In the given figure, tangents PQ and PR are drawn from an external point P to a circle such that ∠RPQ = 30°. A chord RS is drawn parallel to the tangent PQ. Find ∠RQS.
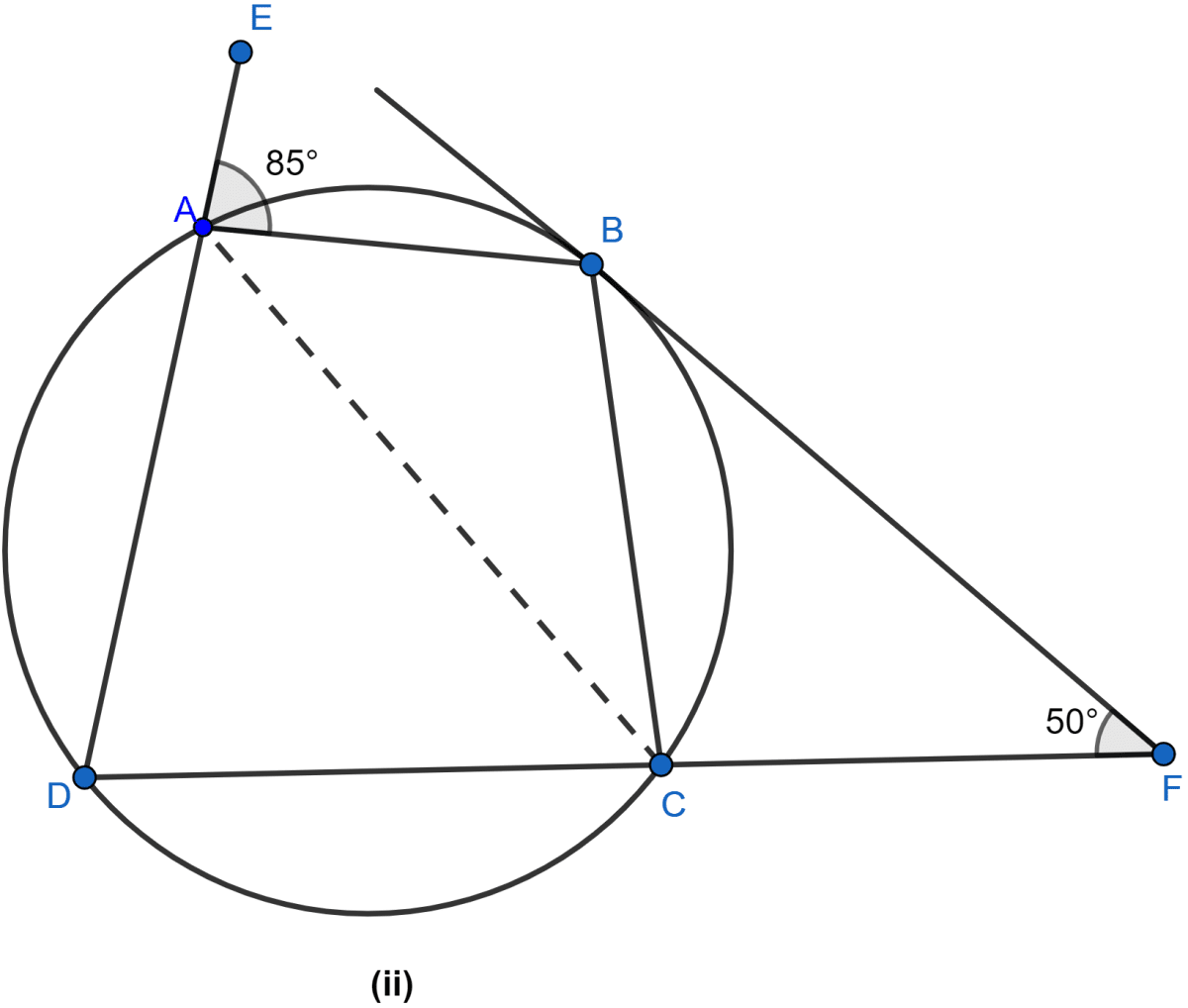
In the figure (ii) given below, ABCD is a cyclic quadrilateral. The tangent to the circle at B meets DC produced at F. If ∠EAB = 85° and ∠BFC = 50°, find ∠CAB.
In the figure (i) given below, O is the centre of the circle and SP is a tangent. If ∠SRT = 65°, find the values of x, y and z.
In the given figure, AD is a diameter of a circle with centre O and AB is tangent at A. C is a point on the circle such that DC produced intersects the tangent at B. If ∠ABC = 50°, find ∠AOC.
