Mathematics
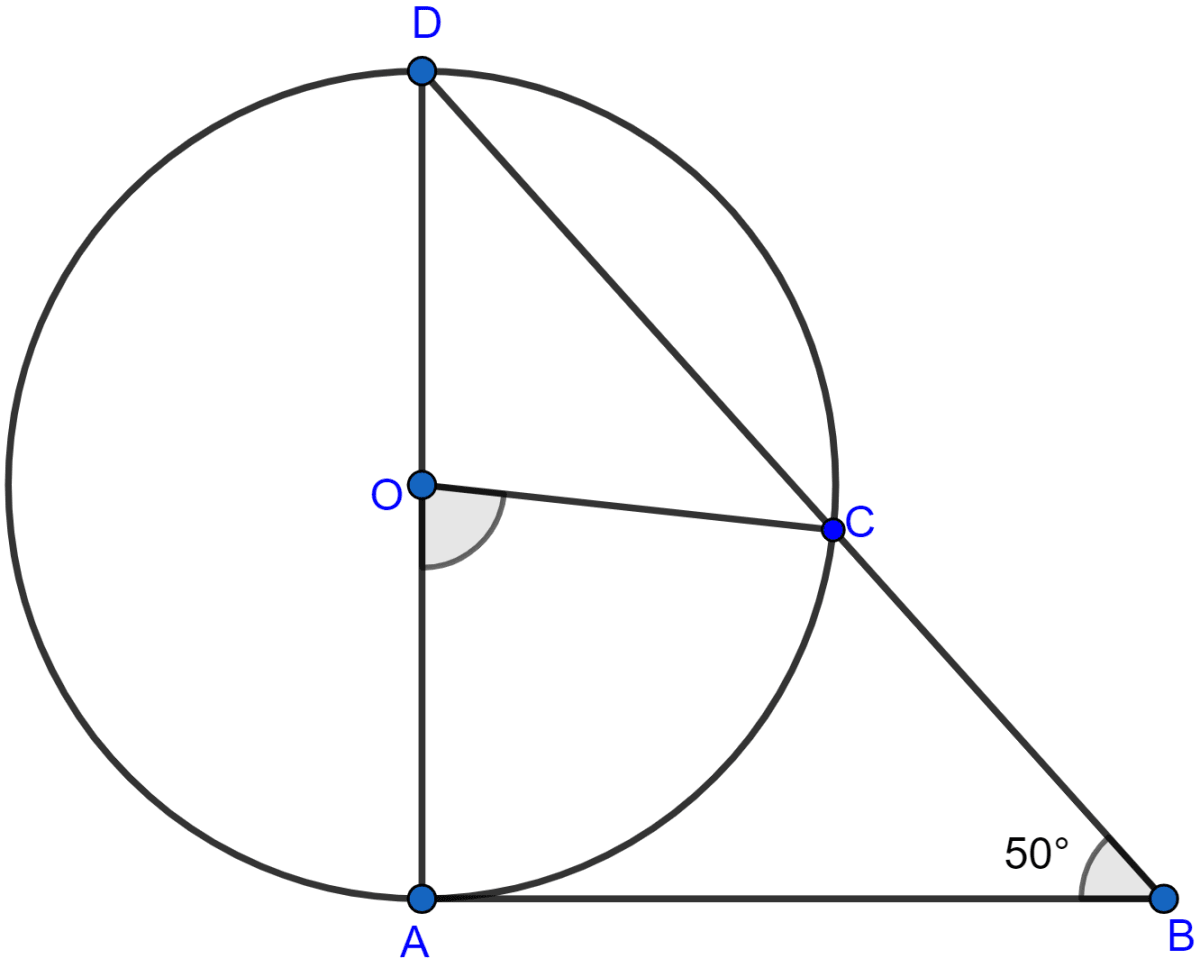
In the given figure, AD is a diameter of a circle with centre O and AB is tangent at A. C is a point on the circle such that DC produced intersects the tangent at B. If ∠ABC = 50°, find ∠AOC.
Answer
In the figure,
AB ⊥ AD. (∵ tangent at a point and radius through the point are perpendicular to each other.)
From figure,
∠ABD = ∠ABC = 50°
In △ABD,
∠ABD + ∠BDA + ∠DAB = 180°
⇒ 50° + ∠BDA + 90° = 180°
⇒ ∠BDA + 140° = 180°
⇒ ∠BDA = 180° - 140°
⇒ ∠BDA = 40°.
From figure,
∠ADC = ∠BDA = 40°.
Arc AC subtends ∠AOC at the centre and ∠ADC on point D.
∴ ∠AOC = 2∠ADC (∵ angle subtended at centre by an arc is double the angle subtended at remaining part of circle.)
∠AOC = 2 × 40° = 80°.
Hence, value of ∠AOC = 80°.
Related Questions
In the figure (i) given below, PA and PB are tangents at the points A and B respectively of a circle with centre O. Q and R are points on the circle If ∠APB = 70°, find
(i) ∠AOB
(ii) ∠AQB
(iii) ∠ARB

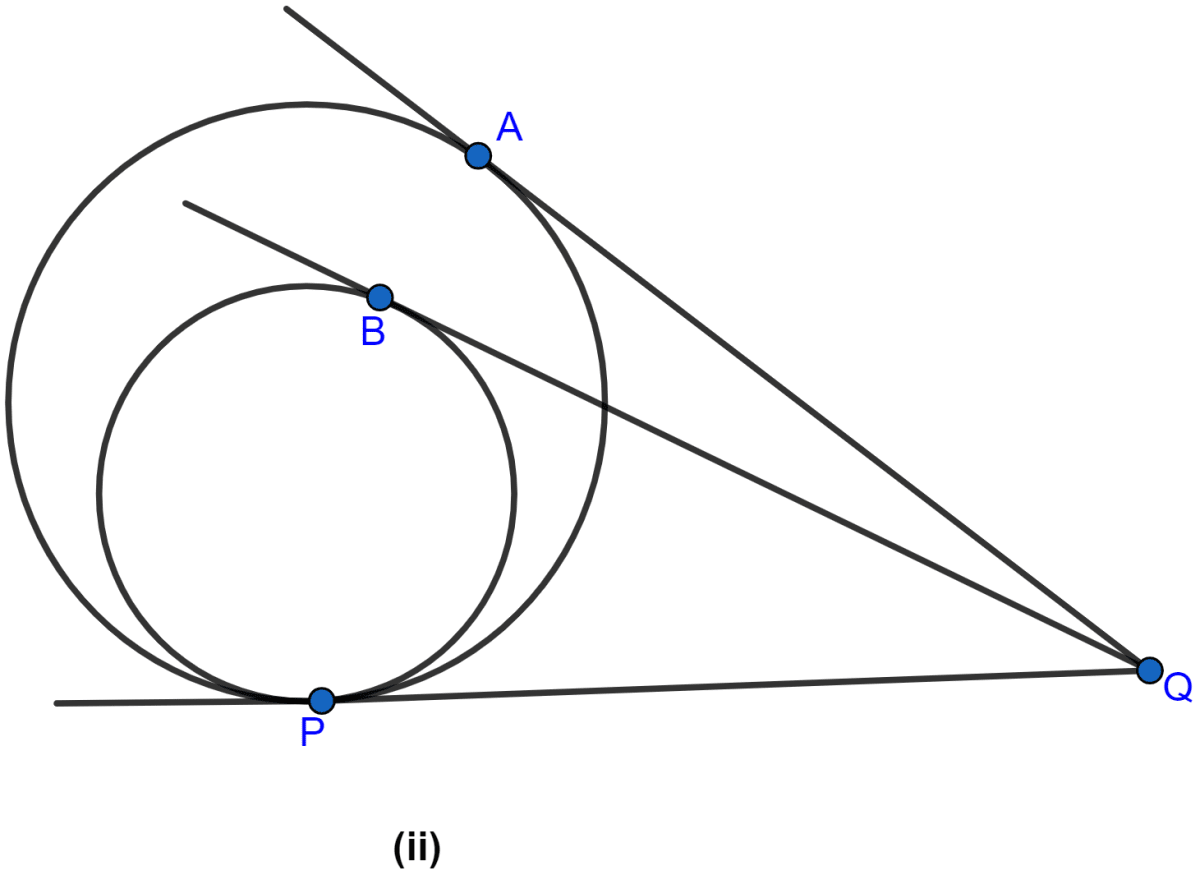
In the figure (ii) given below, two circles touch internally at P from an external point Q on the common tangent at P, two tangents QA and QB are drawn to the two circles. Prove that QA = QB.
In the given figure, tangents PQ and PR are drawn from an external point P to a circle such that ∠RPQ = 30°. A chord RS is drawn parallel to the tangent PQ. Find ∠RQS.

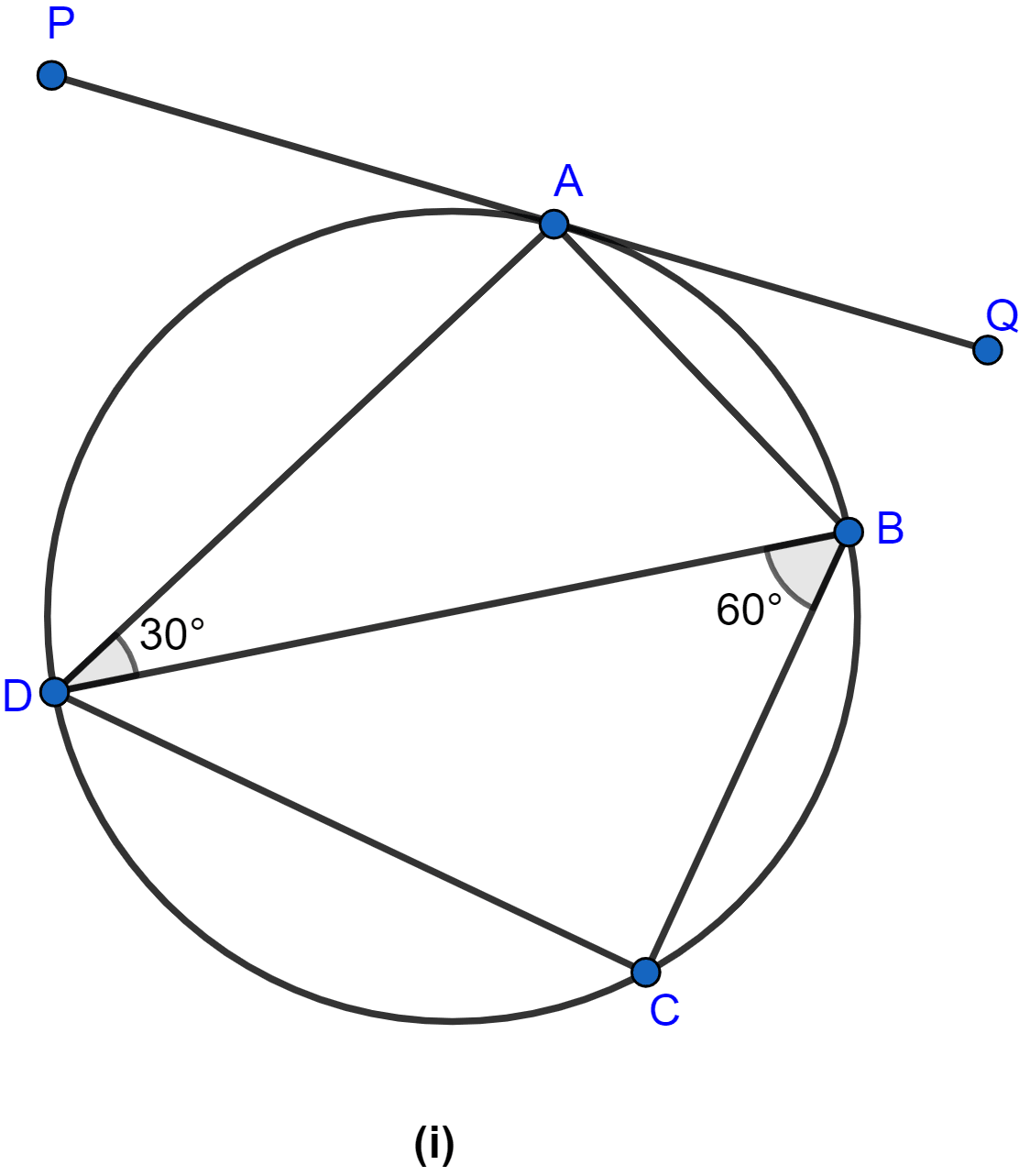
In the figure (i) given below, PQ is a tangent to the circle at A, DB is a diameter, ∠ADB = 30° and ∠CBD = 60°, calculate
(i) ∠QAB
(ii) ∠PAD
(iii) ∠CDB.