Mathematics
In the given figure; ABC, AEQ and CEP are straight lines. Show that ∠APE and ∠CQE are supplementary.
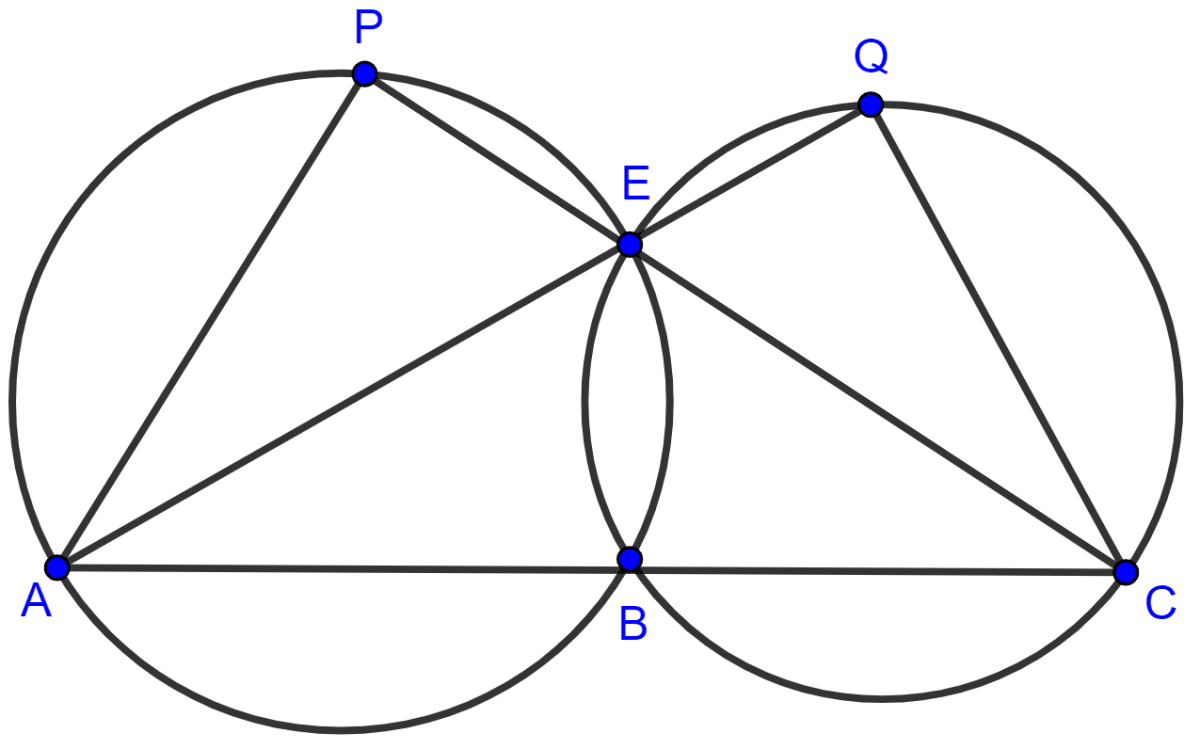
Circles
8 Likes
Answer
Join EB.
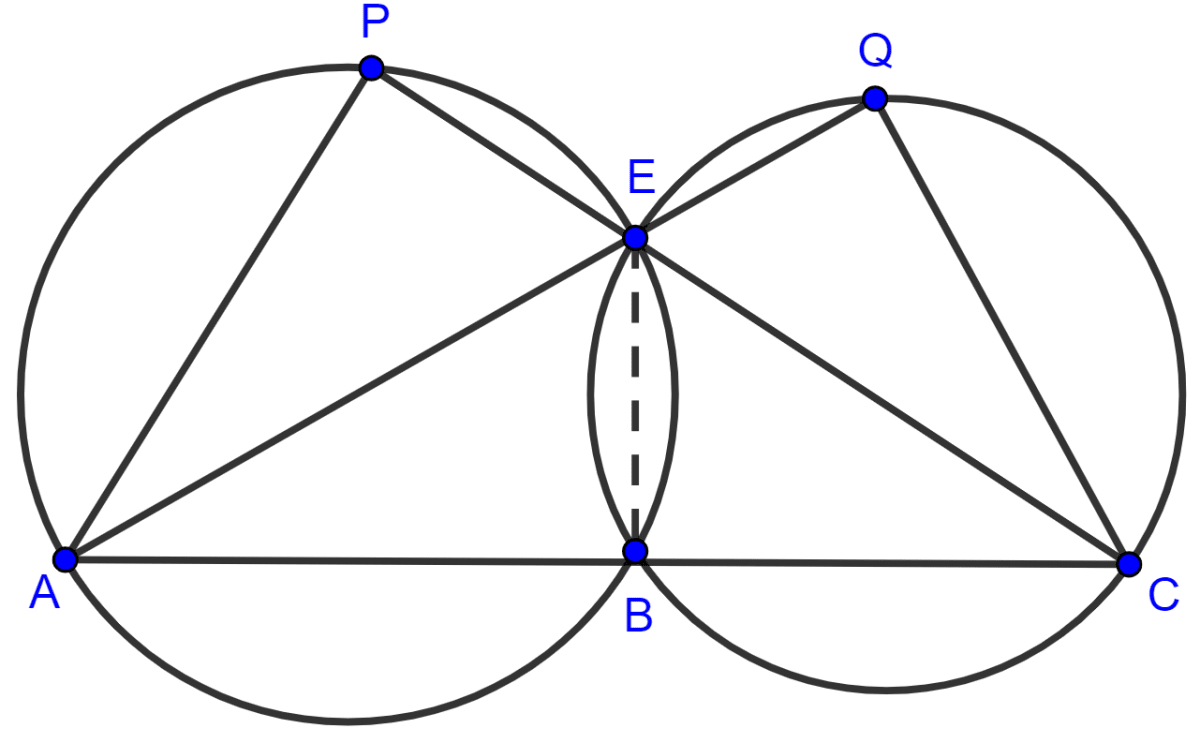
We know that,
In cyclic quadrilateral sum of opposite angles = 180°.
In cyclic quad. ABEP
⇒ ∠APE + ∠ABE = 180° …..(1) [Opposite angles of a cyclic quad. are supplementary]
Similarly, in cyclic quad. BCQE
⇒ ∠CQE + ∠CBE = 180° …..(2)
Adding (1) and (2), we have
⇒ ∠APE + ∠ABE + ∠CQE + ∠CBE = 180° + 180°
⇒ ∠APE + ∠ABE + ∠CQE + ∠CBE = 360° …..(3)
From figure,
∠ABE + ∠CBE = 180° [Linear pair]
Putting this value of ∠ABE + ∠CBE in Eq 3 we get,
∠APE + ∠CQE + 180° = 360°
⇒ ∠APE + ∠CQE = 360° - 180°
⇒ ∠APE + ∠CQE = 180°
Hence, proved that ∠APE and ∠CQE are supplementary.
Answered By
6 Likes
Related Questions
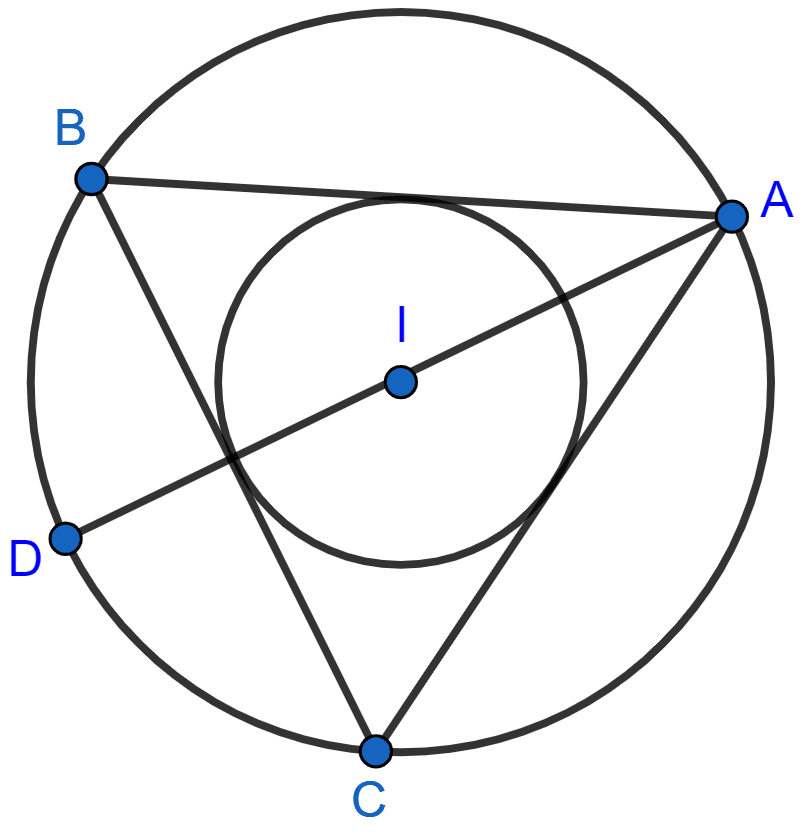
If I is the incentre of triangle ABC and AI when produced meets the circumcircle of triangle ABC in point D. If ∠BAC = 66° and ∠ABC = 80°. Calculate :
(i) ∠DBC,
(ii) ∠IBC,
(iii) ∠BIC.
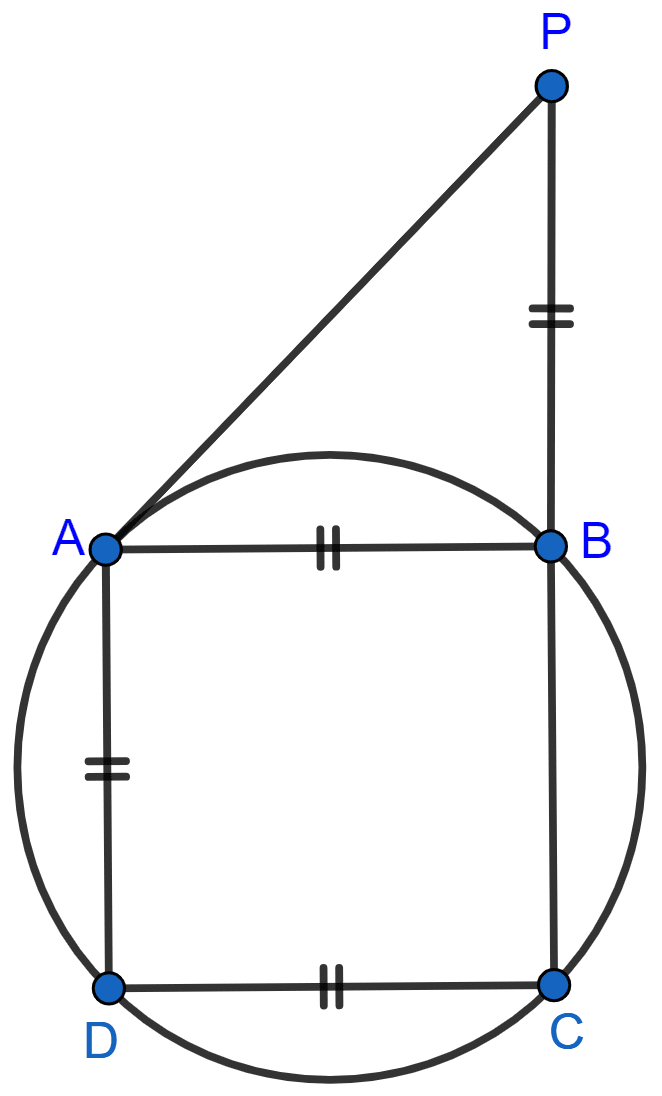
In the given figure, AB = AD = DC = PB and ∠DBC = x°. Determine, in terms of x :
(i) ∠ABD, (ii) ∠APB.
Hence or otherwise, prove that AP is parallel to DB.
In the given figure, AB is the diameter of the circle with centre O.
If ∠ADC = 32°, find angle BOC.

In a cyclic quadrilateral PQRS, angle PQR = 135°. Sides SP and RQ produced meet at point A whereas sides PQ and SR produced meet at point B.
If ∠A : ∠B = 2 : 1, find angles A and B.