Mathematics
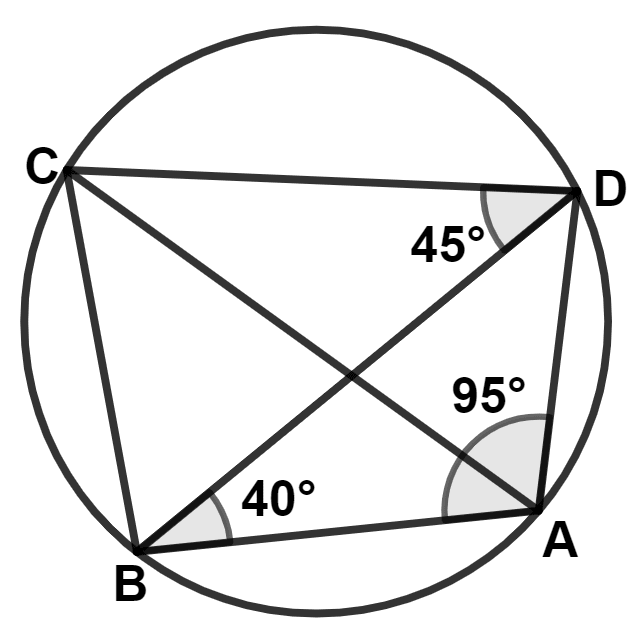
In the given circle, ∠BAD = 95°, ∠ABD = 40° and ∠BDC = 45°.
Assertion (A) : To show that AC is a diameter, the angle ADC or angle ABC need to be proved to be 90°.
Reason (R) : In △ADB,
∠ADB = 180° - 95° - 40° = 45°
∴ Angle ADC = 45° + 45° = 90°
(i) A is true, R is false
(ii) A is true, R is true
(iii) A is false, R is false
(iv) A is false, R is true
Answer
We know that,
Angle in semicircle is a right angle.
If AC is the diameter, then ∠ADC = ∠ABC = 90°.
∴ Assertion (A) is true.
From figure,
In △ADB,
By angle sum property of triangle,
∴ ∠ADB + ∠DBA + ∠BAD = 180°
⇒ ∠ADB + 40° + 95° = 180°
⇒ ∠ADB + 135° = 180°
⇒ ∠ADB = 180° - 135° = 45°.
From figure,
⇒ ∠ADC = ∠ADB + ∠BDC = 45° + 45° = 90°.
∴ Reason (R) is true.
Hence, Option 2 is the correct option.
Related Questions
The figure shows a circle with centre O. AB is the side of regular pentagon and AC is the side of regular hexagon.
Find the angles of triangle ABC.

In the given figure, BD is a side of regular hexagon, DC is a side of a regular pentagon and AD is a diameter. Calculate :
(i) ∠ADC,
(ii) ∠BDA,
(iii) ∠ABC,
(iv) ∠AEC.
In the given figure x°, y°, z° and p° are exterior angles of cyclic quadrilateral ABCD, then x° + y° + z° + p° is :
180°
270°
360°
720°

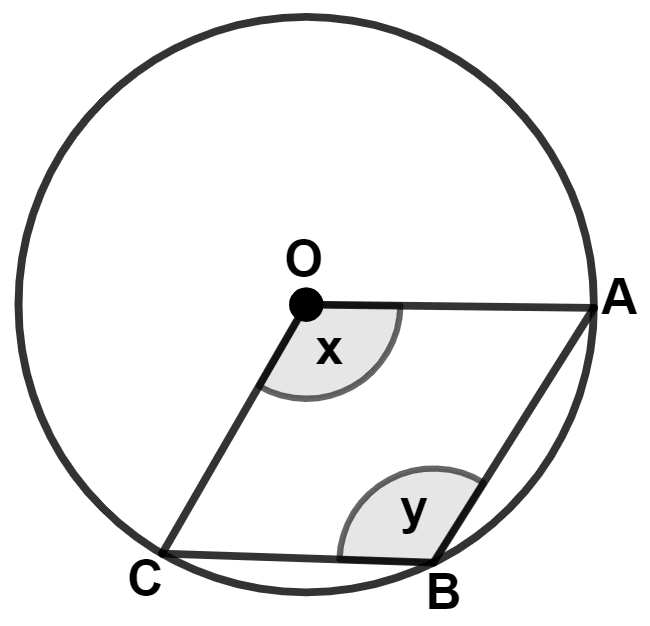
In the given figure, O is center of the circle and OABC is a rhombus, then :
x° + y° = 180°
x° = y° = 90°
x° + 2y° = 360°
x° = y° = 45°