Mathematics
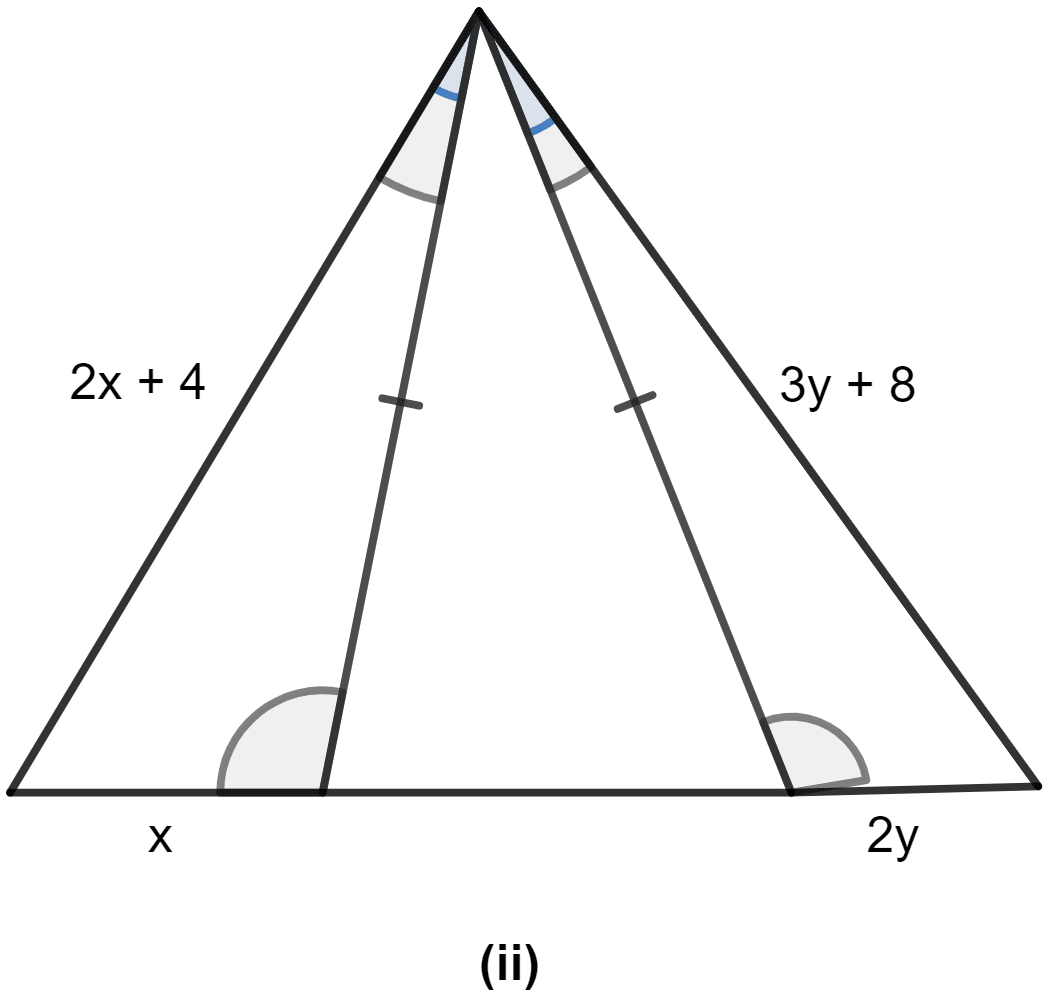
In the following figure, find the values of x and y.
Triangles
54 Likes
Answer
In △ABC and △ADE,
∠BAC = ∠DAE (Given)
∠BCA = ∠EDA (Given)
AC = AD (Given)
∴ △ABC ≅ △ADE (By ASA axiom)
We know that corresponding parts of congruent triangles are equal.
∴ BC = DE
⇒ x = 2y ……(i)
∴ AB = AE
⇒ 2x + 4 = 3y + 8
Substituting value of x from (i) in above equation we get,
⇒ 2(2y) + 4 = 3y + 8
⇒ 4y + 4 = 3y + 8
⇒ 4y - 3y = 8 - 4
⇒ y = 4.
∴ x = 2y = 2(4) = 8.
Hence, x = 8 and y = 4.
Answered By
24 Likes
Related Questions
In the figure (3) given below, BA || DF and CA || EG and BD = EC. Prove that
(i) BG = DF
(ii) EG = CF.

In the figure (2) given below, AB || DC and ∠C = ∠D. Prove that
(i) AD = BC
(ii) AC = BD.

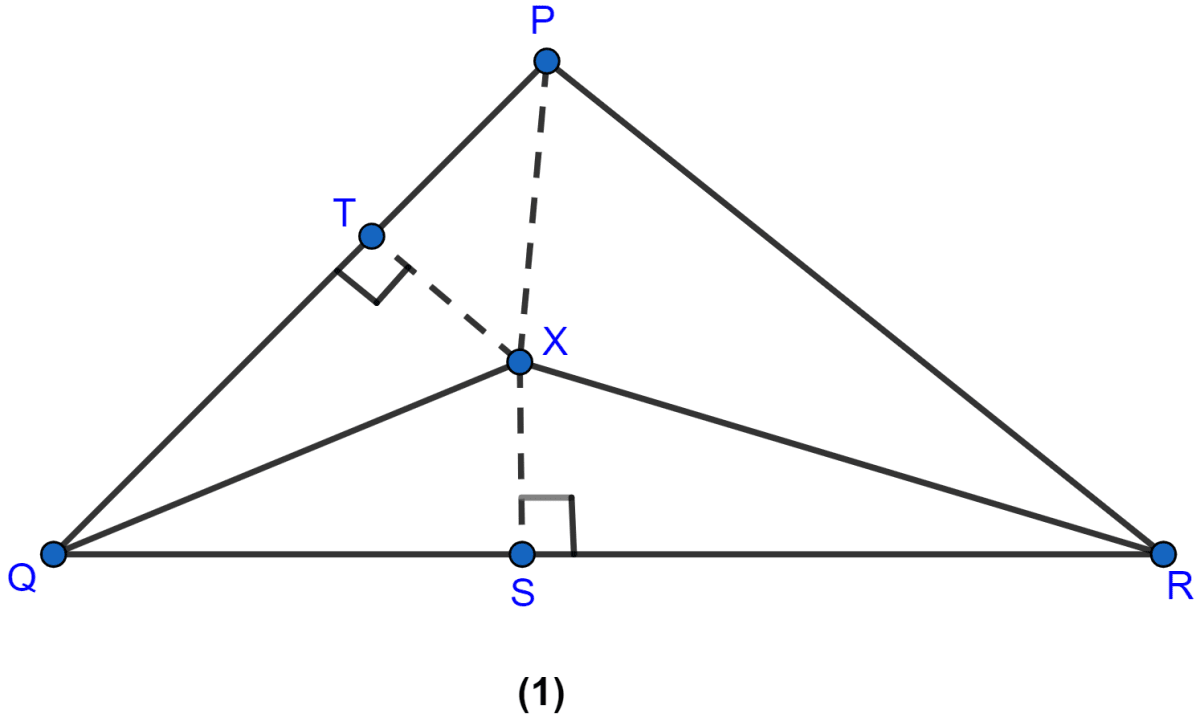
In the figure (1) given below, QX, RX are bisectors of angles PQR and PRQ respectively of △PQR. If XS ⊥ QR and XT ⊥ PQ, prove that
(i) △XTQ ≅ △XSQ
(ii) PX bisects the angle P.
In the following figure, find the values of x and y.
