Mathematics
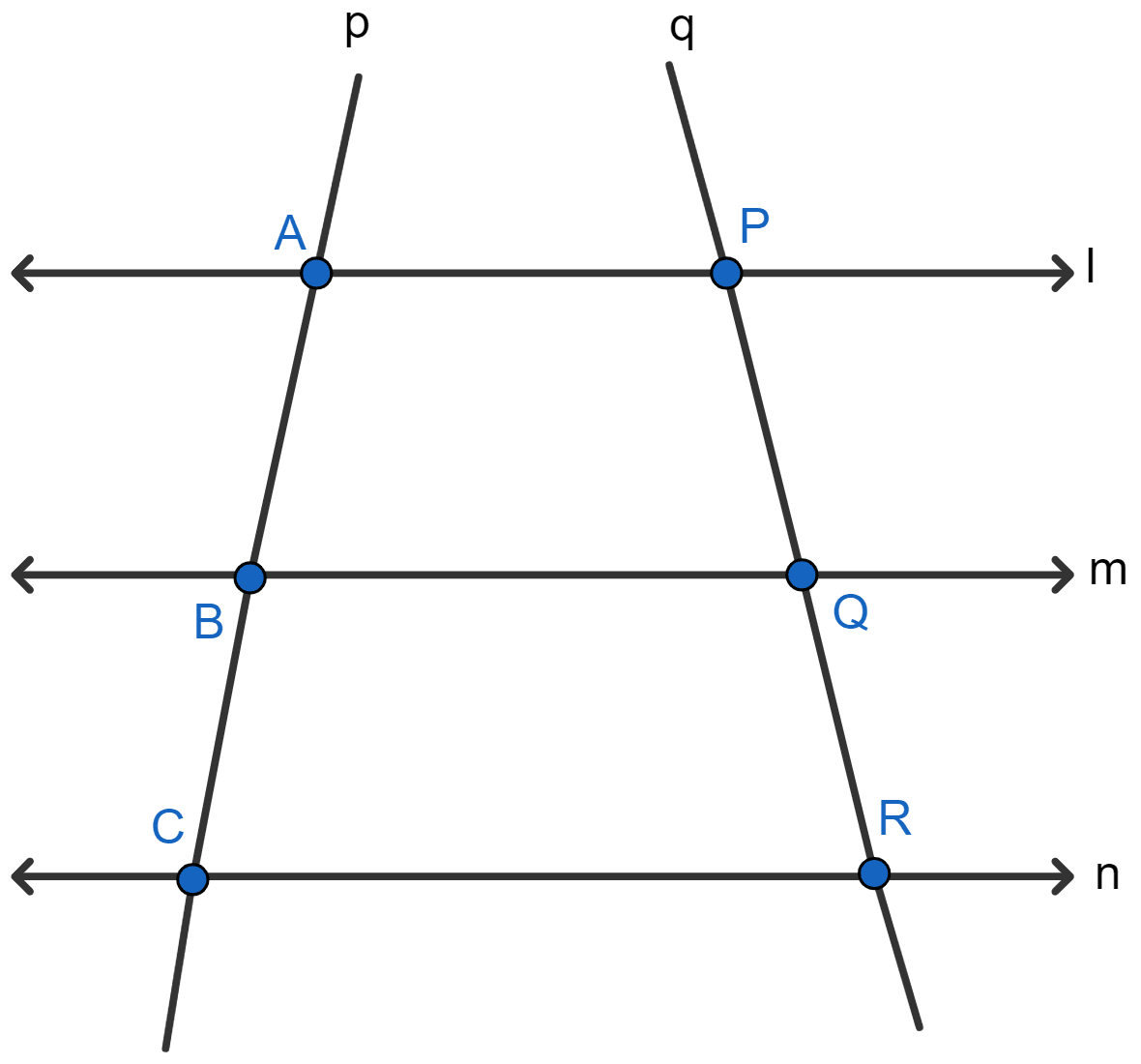
In the following diagram, lines l, m and n are parallel to each other. Two transversals p and q intersect the parallel lines at points A, B, C and P, Q, R as shown.
Prove that:
Similarity
5 Likes
Answer
Join A and R. Let AR meet BQ at point D.
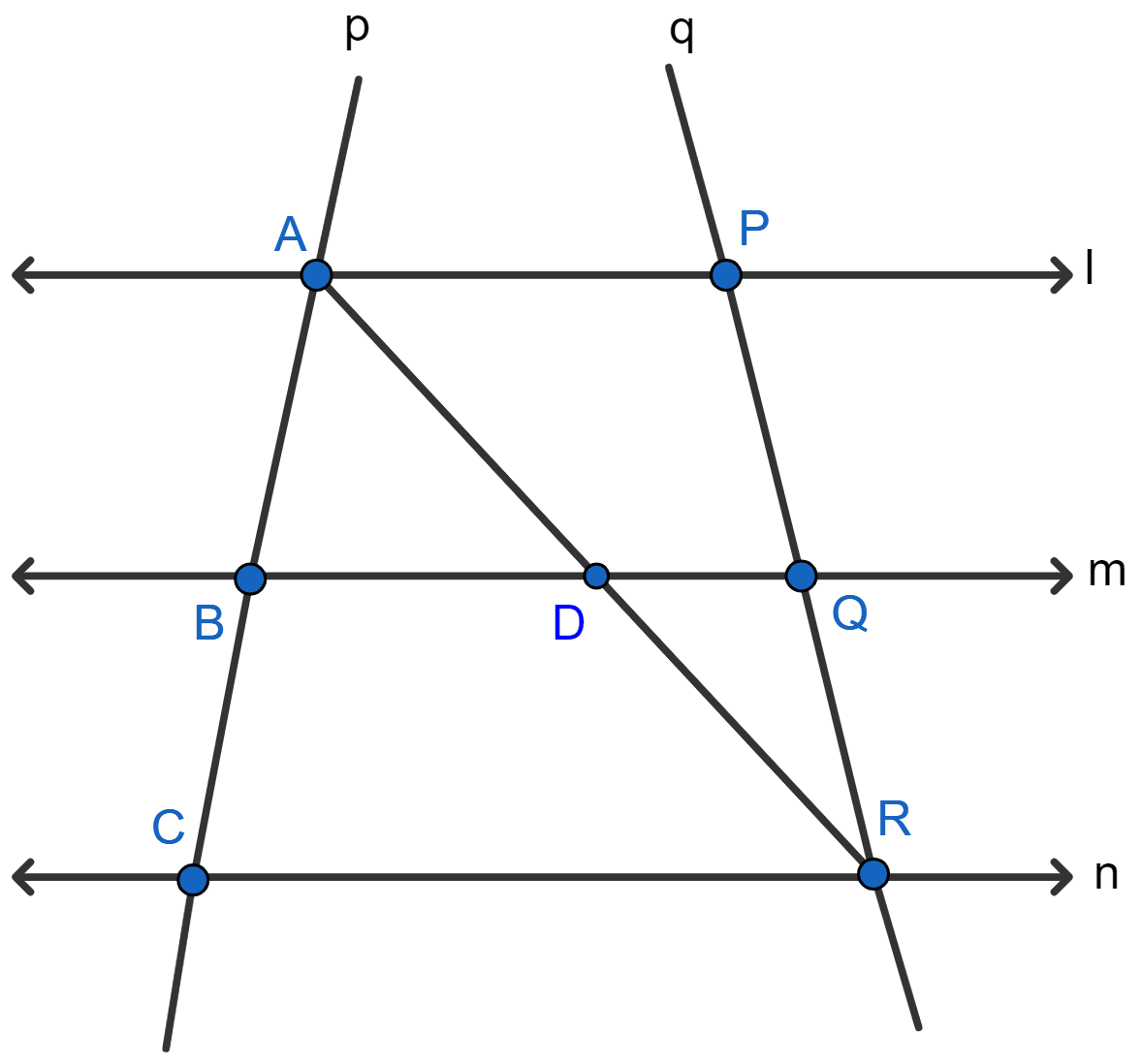
In ∆ACR, BD || CR.
By Basic proportionality theorem, we get
…..(1)
In ∆APR, DQ || AP.
By Basic proportionality theorem, we get
…..(2)
From (1) and (2) we get :
Hence proved that .
Answered By
2 Likes
Related Questions
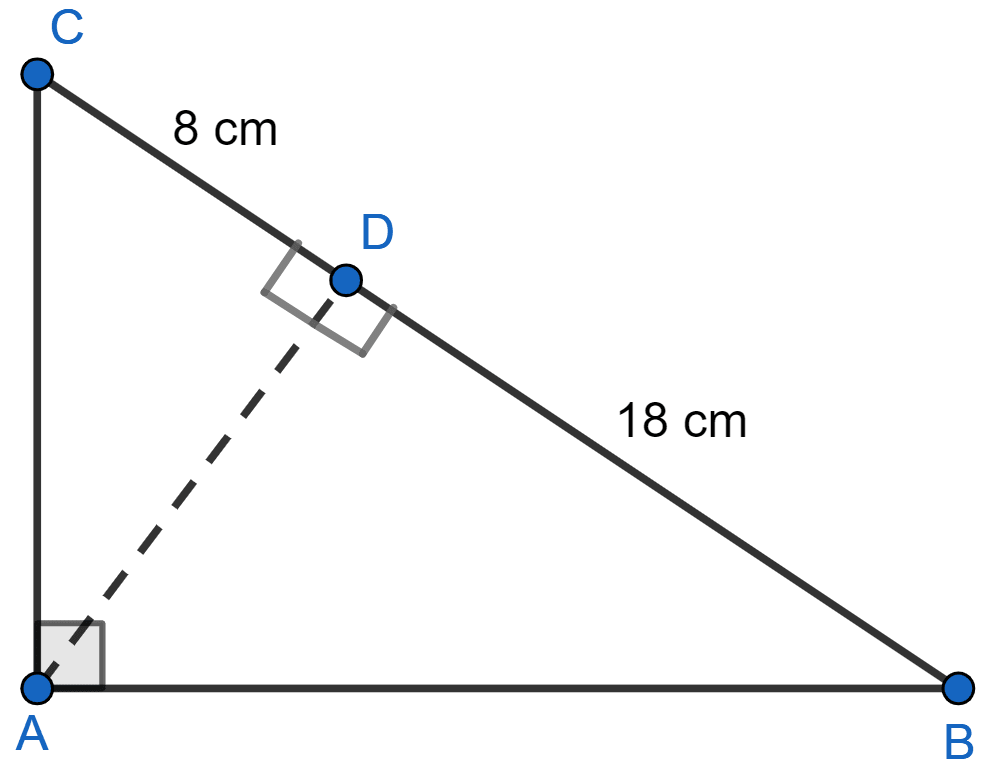
In the given figure, ABC is a right angled triangle with ∠BAC = 90°.
(i) Prove that : △ADB ~ △CDA.
(ii) If BD = 18 cm and CD = 8 cm, find AD.
(iii) Find the ratio of the area of △ADB is to area of △CDA.
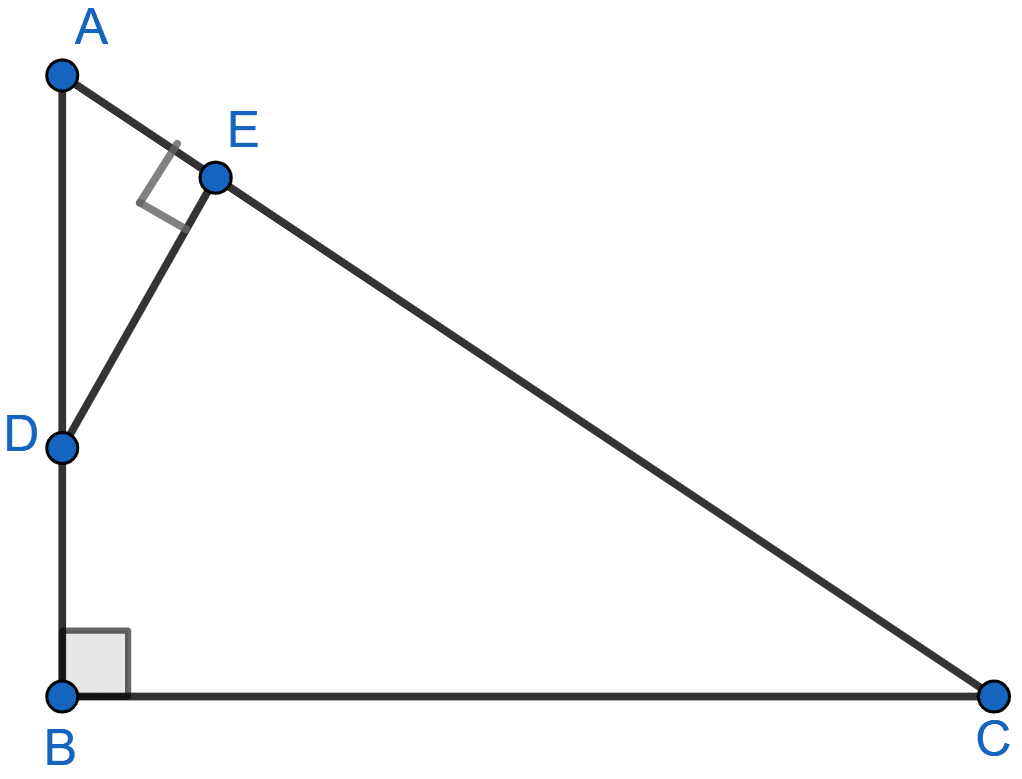
ABC is a right angled triangle with ∠ABC = 90°. D is any point on AB and DE is perpendicular to AC. Prove that :
(i) △ADE ~ △ACB
(ii) If AC = 13 cm, BC = 5 cm and AE = 4 cm. Find DE and AD.
(iii) Find, area of △ADE : area of quadrilateral BCED.
Given : AB || DE and BC || EF. Prove that :
(i)
(ii) △DFG ~ △ACG.

PQR is a triangle. S is a point on the side QR of △PQR such that ∠PSR = ∠QPR. Given QP = 8 cm, PR = 6 cm and SR = 3 cm.
(i) Prove △PQR ~ △SPR.
(ii) Find the lengths of QR and PS.
(iii)
