Mathematics
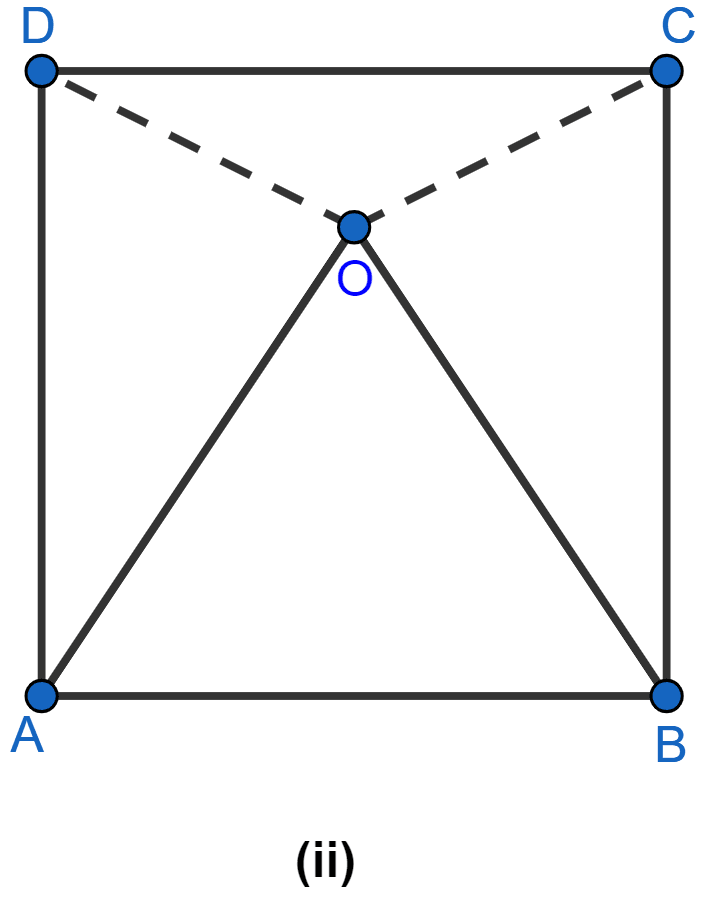
In the figure (ii) given below, O is the point in the interior of a square ABCD such that OAB is an equilateral triangle. Show that OCD is an isosceles triangle.
Triangles
16 Likes
Answer
∠OAD = ∠DAB - ∠OAB = 90° - 60° = 30°.
Similarly,
∠OBC = ∠CBA - ∠OBA = 90° - 60° = 30°.
⇒ ∠OAD = ∠OBC.
AD = BC (As sides of squares are equal).
OA = OB (As OAB is equilateral triangle).
∴ △OAD ≅ △OBC by SAS axiom.
We know that corresponding parts of congruent triangles are equal.
∴ OD = OC.
Hence, proved that OD = OC i.e. OCD is an isosceles triangle.
Answered By
11 Likes
Related Questions
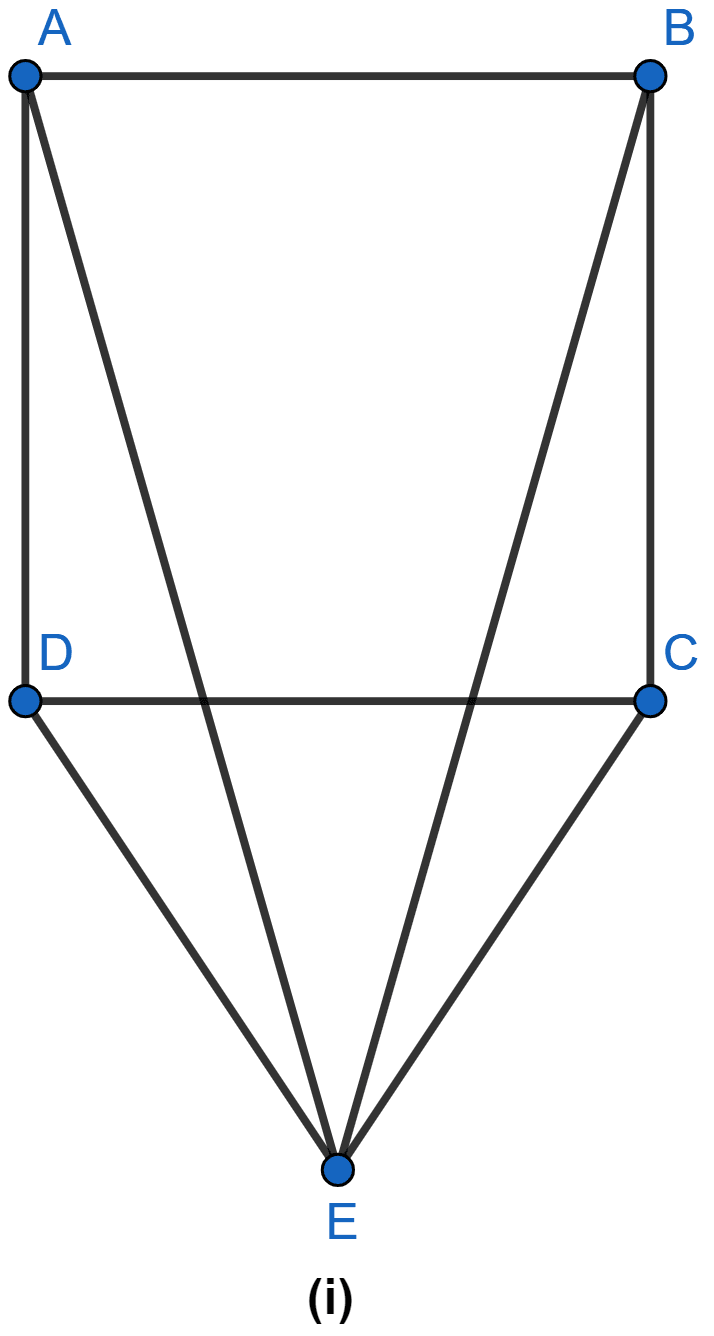
In the figure (i) given below, CDE is an equilateral triangle formed on a side CD of a square ABCD. Show that △ADE ≅ △BCE and hence, AEB is an isosceles triangle.
In the adjoining figure, D and E are points on the side BC of △ABC such that BD = EC and AD = AE. Show that △ABD ≅ △ACE.
ABC is an isosceles triangle in which AB = AC. P is any point in the interior of △ABC such that ∠ABP = ∠ACP. Prove that
(a) BP = CP
(b) AP bisects ∠BAC.
In the adjoining figure, ABC is a right triangle with AB = AC. Bisector of ∠A meets BC at D. Prove that BC = 2AD.
