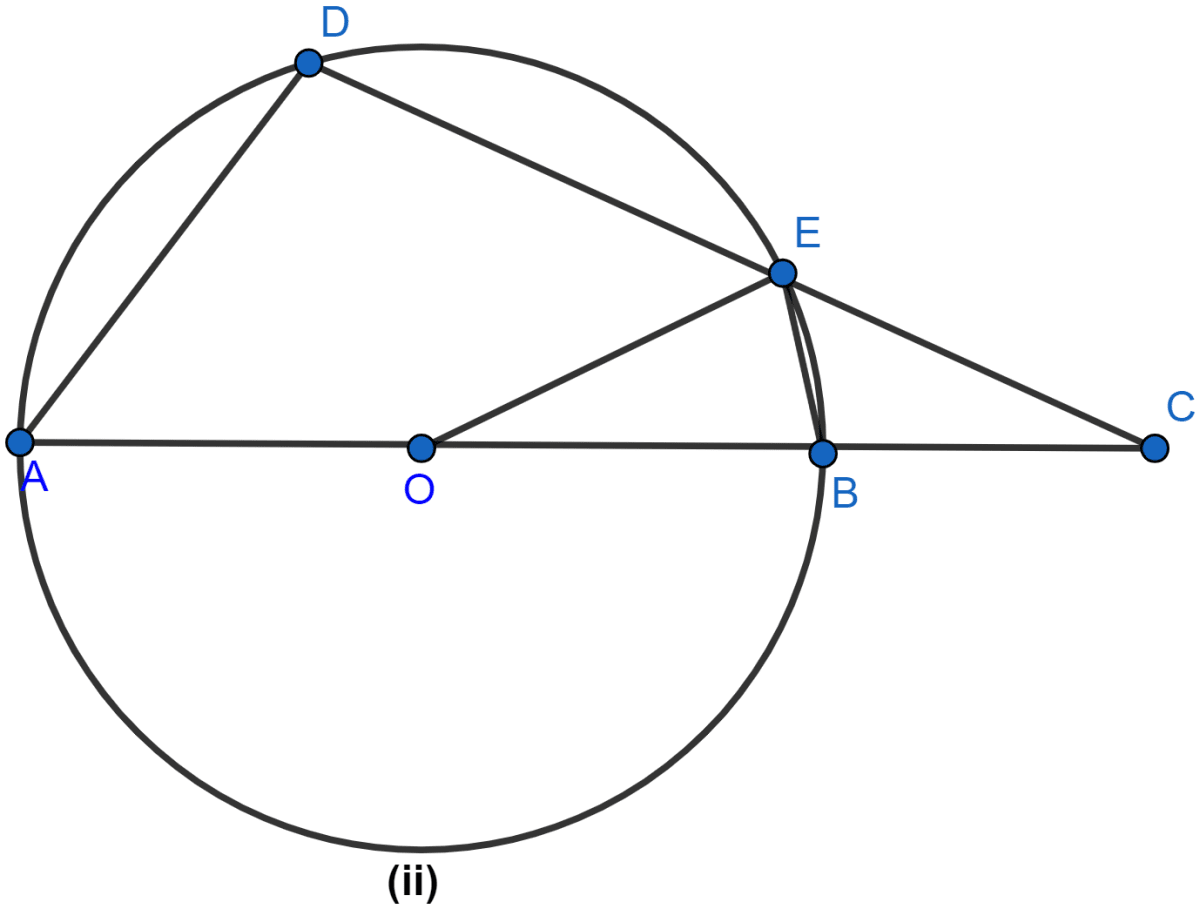
In the figure (ii) given below, O is the center of the circle. ∠AOE = 150°, ∠DAO = 51°. Calculate the sizes of ∠BEC and ∠EBC.

From figure,

∠DAB = ∠DAO = 51°.

ABED is a cyclic quadrilateral as all vertices lie on the circumference of the circle.

∠BEC = ∠DAB = 51° (∵ exterior angle of a cyclic quadrilateral is equal to the opposite interior angle.)

Reflex ∠AOE = 360° - ∠AOE = 360° - 150° = 210°.

Arc AE subtends ∠ADE at point D and Reflex ∠AOE at center.

Reflex ∠AOE = 2∠ADE (∵ angle subtended by an arc at center is double the angle subtended at any other point of the circle.)

210° = 2∠ADE
∠ADE = $\dfrac{210°}{2}$
∠ADE = 105°.

∠EBC = ∠ADE = 105° (∵ exterior angle of a cyclic quadrilateral is equal to the opposite interior angle.)

Hence, ∠BEC = 51° and ∠EBC = 105°.