Mathematics
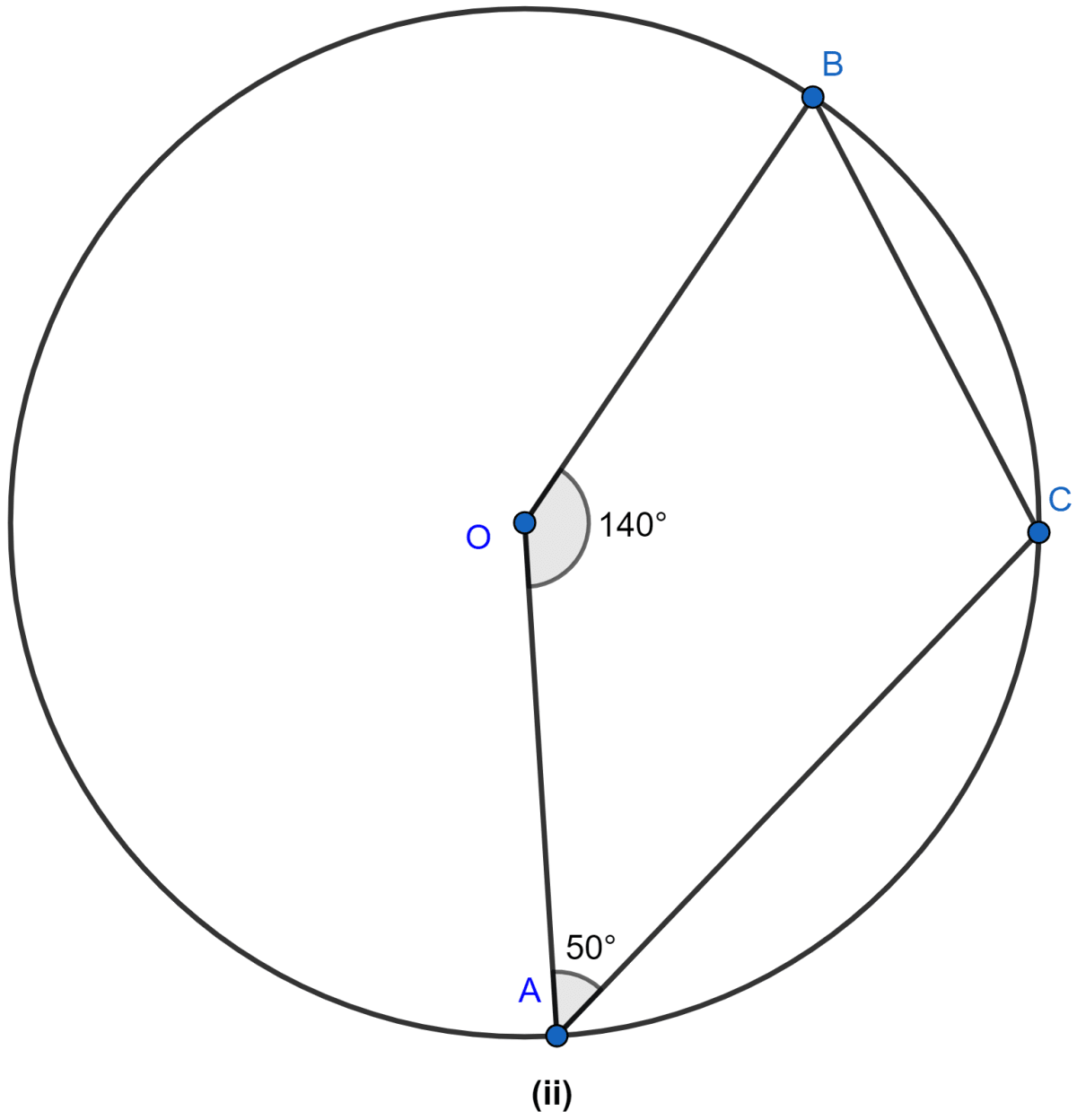
In the figure (ii) given below, O is the center of the circle. If ∠AOB = 140° and ∠OAC = 50°, find
(i) ∠ACB
(ii) ∠OBC
(iii) ∠OAB
(iv) ∠CBA.
Circles
22 Likes
Answer
(i) From figure,
⇒ ∠AOB + Reflex ∠AOB = 360°
⇒ 140° + Reflex ∠AOB = 360°
⇒ Reflex ∠AOB = 360° - 140°
⇒ Reflex ∠AOC = 220°.
Arc AB subtends Reflex ∠AOB at center and ∠ACB at point C of circle.
⇒ Reflex ∠AOB = 2 ∠ACB
⇒ 2∠ACB = 220°
⇒ ∠ACB =
⇒ ∠ACB = 110°.
Hence, the value of ∠ACB = 110°.
(ii) In Quadrilateral OABC,
⇒ ∠OAC + ∠ACB + ∠BOA + ∠OBC = 360°
⇒ 50° + 110° + 140° + ∠OBC = 360°
⇒ 300° + ∠OBC = 360°
⇒ ∠OBC = 360° - 300°
⇒ ∠OBC = 60°
Hence, the value of ∠OBC = 60°.
(iii) In △OAB,
OA = OB (Radius of the circle)
∠OAB = ∠OBA = x (∵ angles of equal sides in isosceles triangle are equal.)
Sum of angles in a triangle are equal,
⇒ ∠AOB + ∠OAB + ∠OBA = 180°.
⇒ 140° + x + x = 180°
⇒ 140° + 2x = 180°
⇒ 2x = 40°
⇒ x = 20°.
Hence, the value of ∠OAB = 20°.
(iv) ∠CBA = ∠OBC - ∠OBA
⇒ ∠CBA = 60° - 20° ⇒ ∠CBA = 40°
Hence, the value of ∠CBA = 40°.
Answered By
15 Likes
Related Questions
In the figure (ii) given below, O is the center of the circle. Calculate the values of x and y.

In the figure (i) given below, M, A, B, N are points on a circle having center O. AN and MB cut at Y. If ∠NYB = 50° and ∠YNB = 20°, find ∠MAN and the reflex angle MON.

In the figure (i) given below, O is the center of the circle and ∠PBA = 42°. Calculate the value of ∠PQB.
In the figure (ii) given below, AB is a diameter of the circle whose center is O. Given that ∠ECD = ∠EDC = 32°, calculate
(i) ∠CEF
(ii) ∠COF
