Mathematics
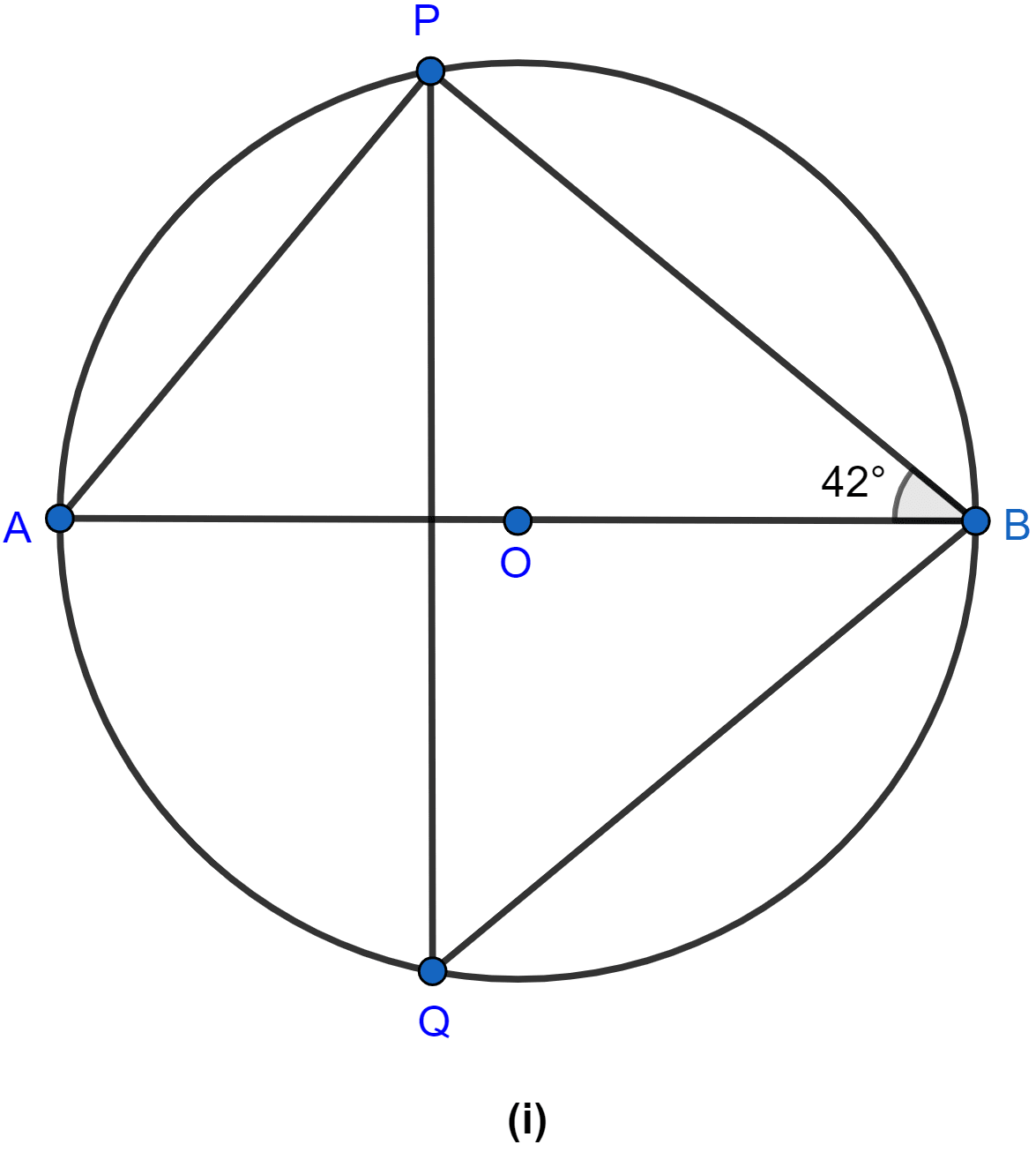
In the figure (i) given below, O is the center of the circle and ∠PBA = 42°. Calculate the value of ∠PQB.
Circles
5 Likes
Answer
Considering △APB,
∠APB = 90° (∵ angle in a semicircle is 90°.)
We know that sum of angles of triangle is 180°.
⇒ ∠APB + ∠PBA + ∠PAB = 180°.
⇒ 90° + 42° + ∠PAB = 180°
⇒ 132° + ∠PAB = 180°
⇒ ∠PAB = 180° - 132°
⇒ ∠PAB = 48°.
Considering △PAB and △PQB,
∠PAB = ∠PQB = 48° (∵ angles in same segment are equal.)
Hence, the value of ∠PQB = 48°.
Answered By
2 Likes
Related Questions
In the figure (ii) given below, AB is a diameter of the circle whose center is O. Given that ∠ECD = ∠EDC = 32°, calculate
(i) ∠CEF
(ii) ∠COF
In the figure (i) given below, M, A, B, N are points on a circle having center O. AN and MB cut at Y. If ∠NYB = 50° and ∠YNB = 20°, find ∠MAN and the reflex angle MON.
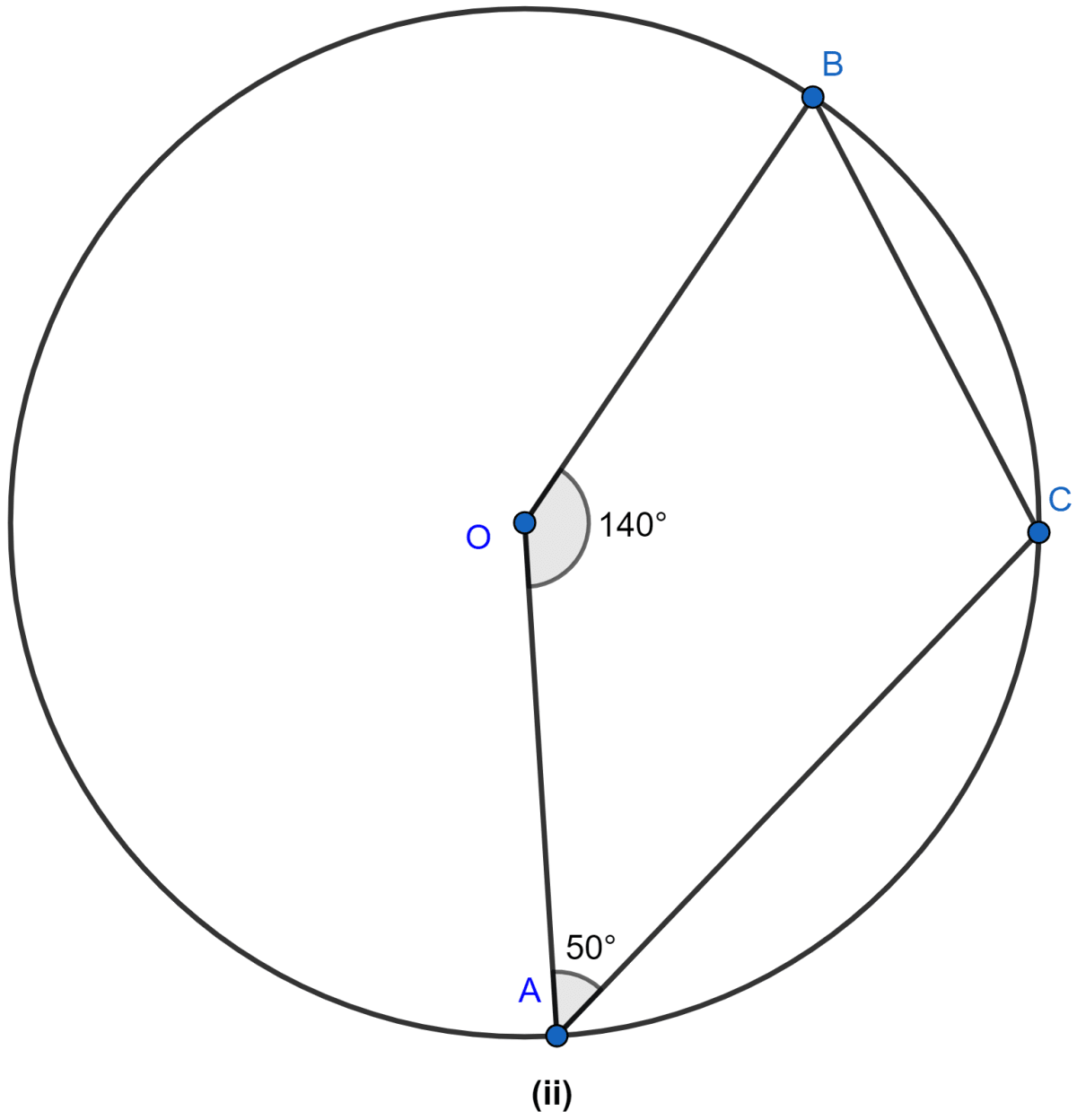
In the figure (ii) given below, O is the center of the circle. If ∠AOB = 140° and ∠OAC = 50°, find
(i) ∠ACB
(ii) ∠OBC
(iii) ∠OAB
(iv) ∠CBA.
In the figure (i) given below, AB is a diameter of the circle APBR. APQ and RBQ are straight lines, ∠A = 35°, ∠Q = 25°. Find :
(i) ∠PRB
(ii) ∠PBR
(iii) ∠BPR
