Mathematics
In the figure (ii) given below, O is the center of a circle. If AB and AC are chords of the circle such that AB = AC and OP ⊥ AB, OQ ⊥ AC, prove that PB = QC.
Circles
15 Likes
Answer
Let AB = AC = x
Given,
OM ⊥ AB and ON ⊥ AC
Since, the perpendicular to a chord from the centre of the circle bisects the chord,
∴ AM = MB =
and
AN = NC =
∴ MB = NC ……….(1)
Since, equal chords of a circle are equidistant from the centre,
∴ ON = OM = y (let).
Let radius of circle be r.
From figure,
OQ = OP = r
QN = OQ - ON = r - y
PM = OP - OM = r - y
∴ QN = PM ……….(2)
In △QNC and △PMB,
NC = MB [From (1)]
QN = PM [From (2)]
∠QNC = ∠PMB (Both equal to 90°)
△QNC ≅ △PMB by SAS axiom.
∴ PB = QC (By C.P.C.T.)
Hence, proved that PB = QC.
Answered By
10 Likes
Related Questions
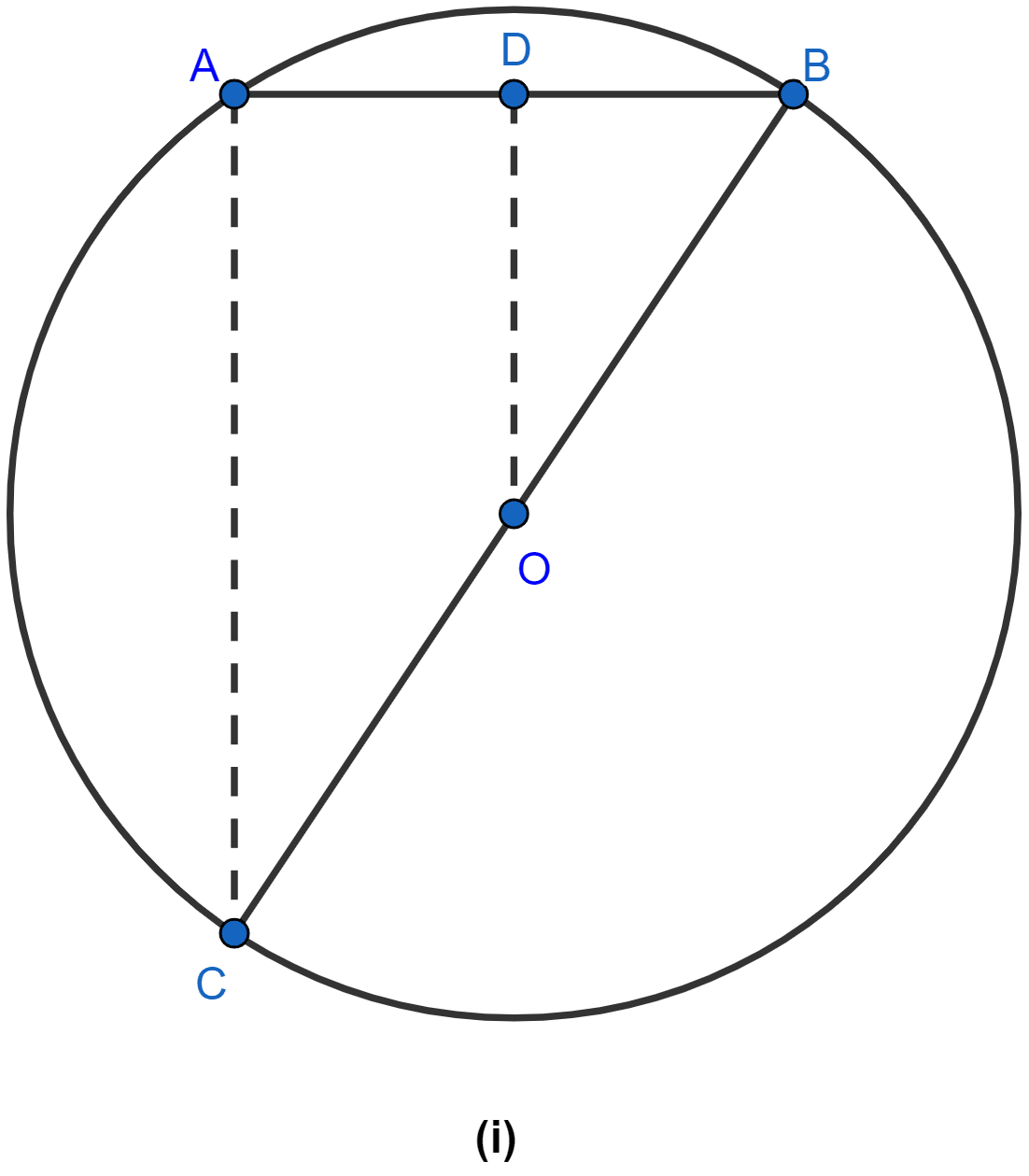
In the figure (i) given below, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2OD.
In an equilateral triangle, prove that the centroid and the circumcentre of the triangle coincide.
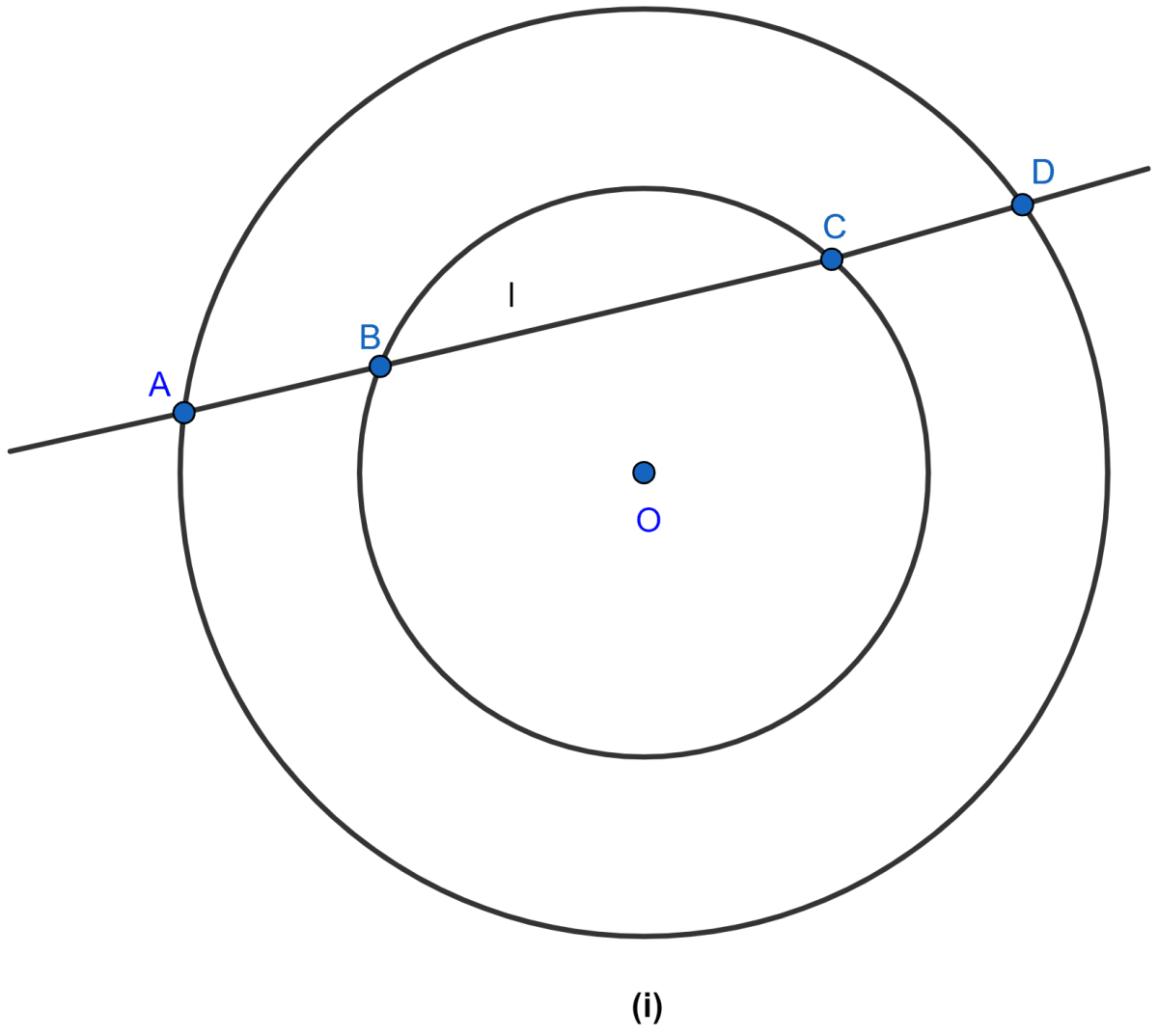
In the figure (i) given below, a line l intersects two concentric circles at the points A, B, C and D. Prove that AB = CD.
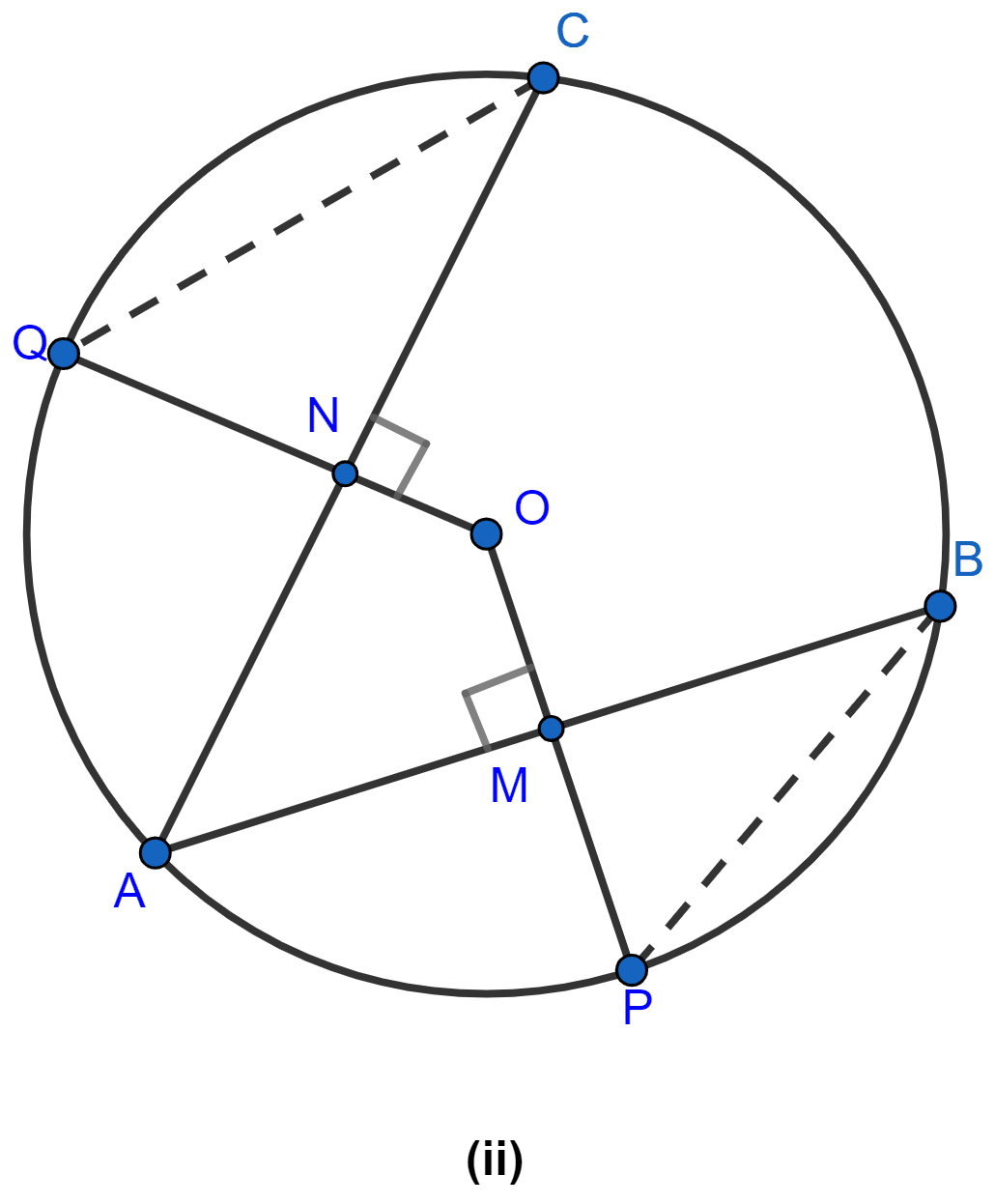
In the figure (ii) given below, chords AB and CD of a circle with centre O intersect at E. If OE bisects ∠AED, prove that AB = CD.
