Mathematics
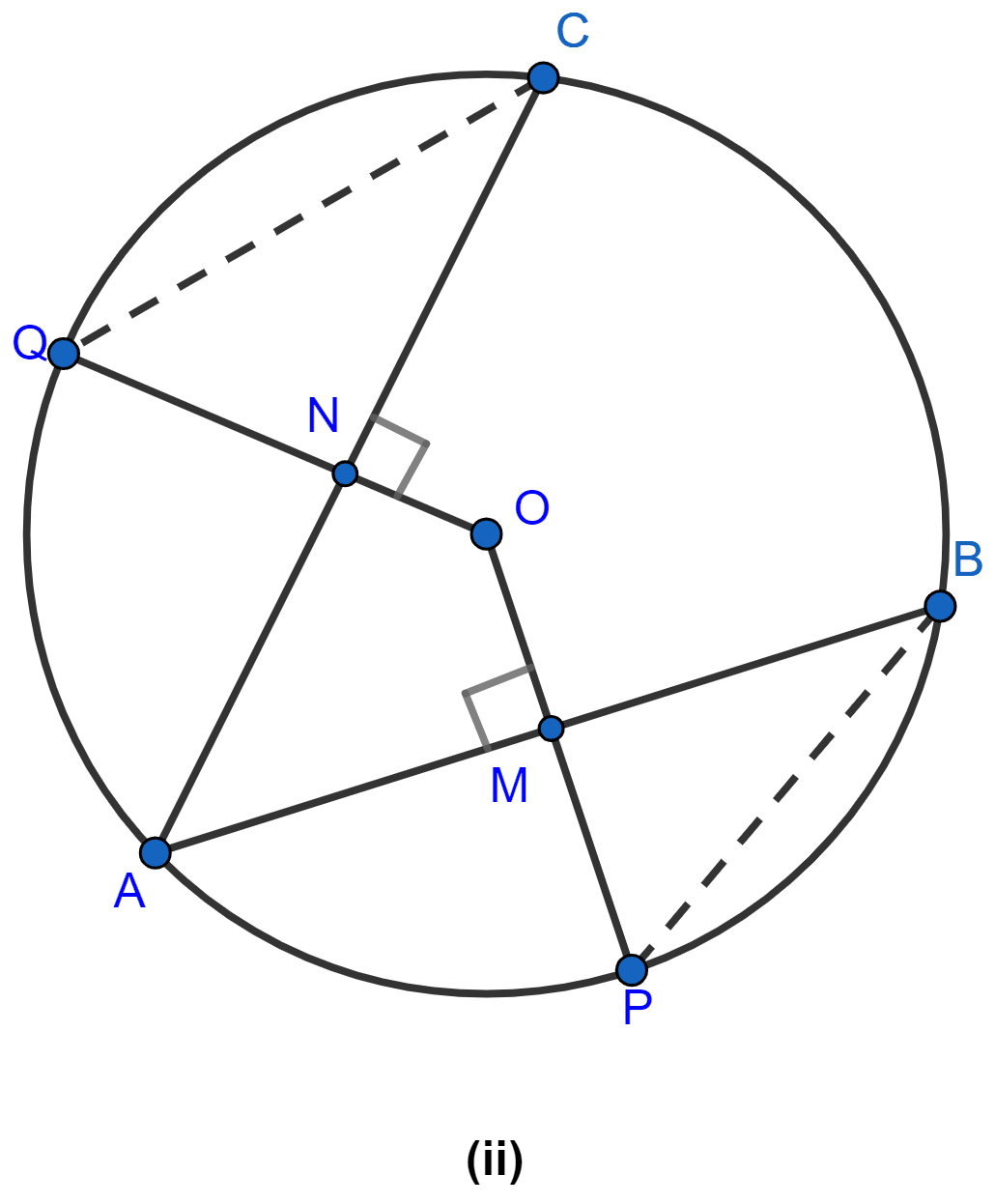
In the figure (ii) given below, O is the center of a circle. If AB and AC are chords of the circle such that AB = AC and OP ⊥ AB, OQ ⊥ AC, prove that PB = QC.
Circles
15 Likes
Answer
Let AB = AC = x
Given,
OM ⊥ AB and ON ⊥ AC
Since, the perpendicular to a chord from the centre of the circle bisects the chord,
∴ AM = MB =
and
AN = NC =
∴ MB = NC ……….(1)
Since, equal chords of a circle are equidistant from the centre,
∴ ON = OM = y (let).
Let radius of circle be r.
From figure,
OQ = OP = r
QN = OQ - ON = r - y
PM = OP - OM = r - y
∴ QN = PM ……….(2)
In △QNC and △PMB,
NC = MB [From (1)]
QN = PM [From (2)]
∠QNC = ∠PMB (Both equal to 90°)
△QNC ≅ △PMB by SAS axiom.
∴ PB = QC (By C.P.C.T.)
Hence, proved that PB = QC.
Answered By
10 Likes
Related Questions
In an equilateral triangle, prove that the centroid and the circumcentre of the triangle coincide.
In the figure (ii) given below, chords AB and CD of a circle with centre O intersect at E. If OE bisects ∠AED, prove that AB = CD.

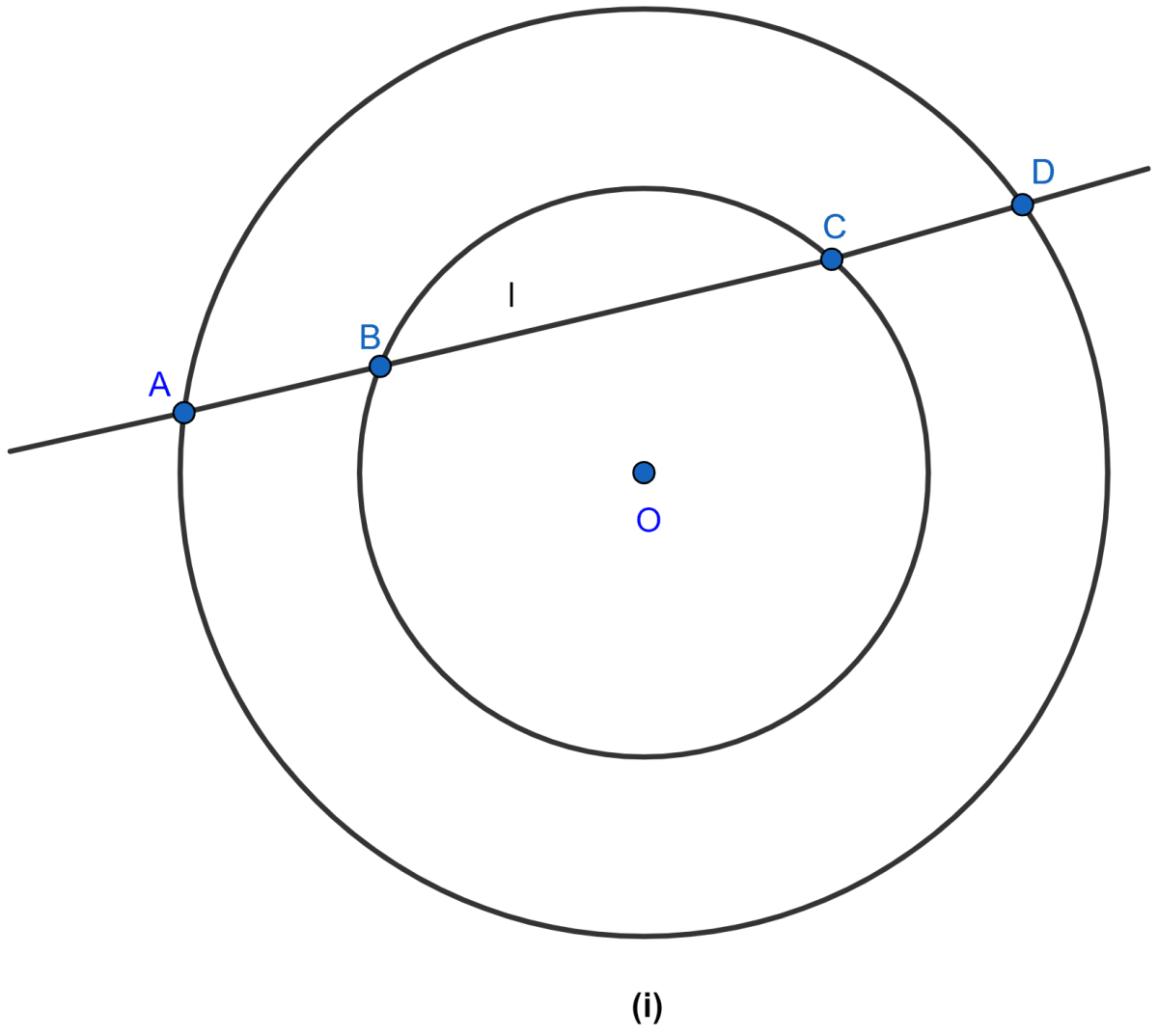
In the figure (i) given below, a line l intersects two concentric circles at the points A, B, C and D. Prove that AB = CD.
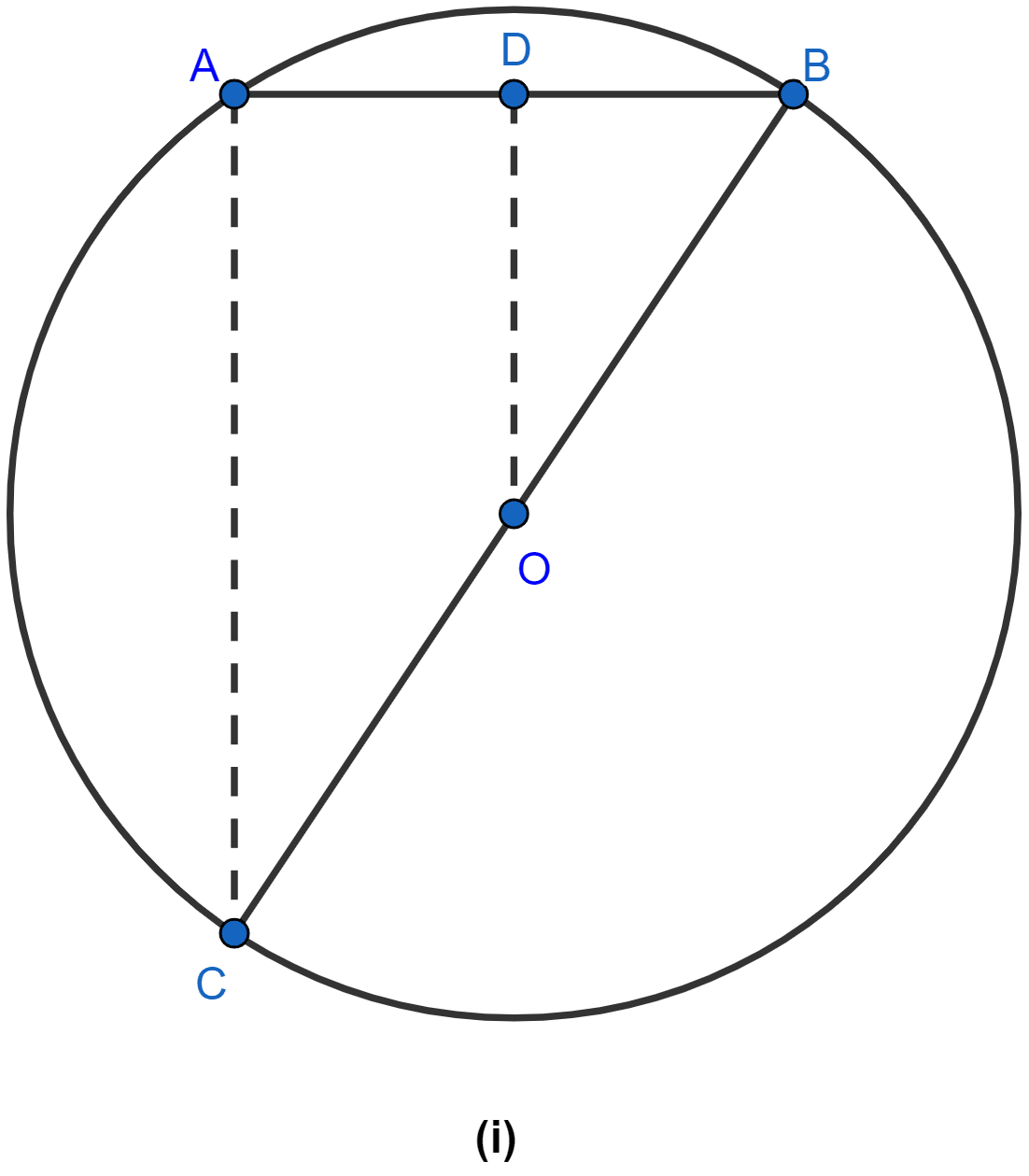
In the figure (i) given below, OD is perpendicular to the chord AB of a circle whose center is O. If BC is a diameter, show that CA = 2OD.