Mathematics
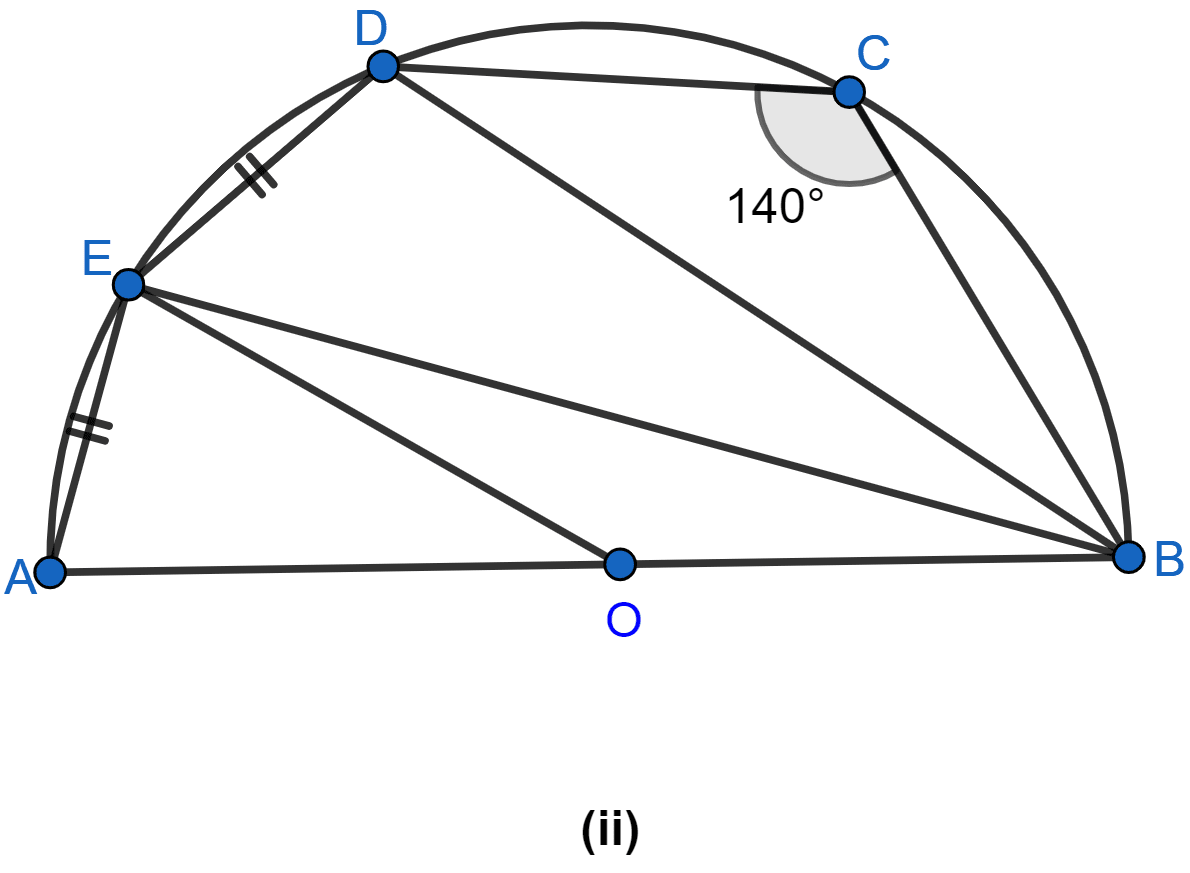
In the figure (ii) given below, AB is a diameter of the semicircle ABCDE with centre O. If AE = ED and ∠BCD = 140°, find ∠AED and ∠EBD. Also prove that OE is parallel to BD.
Circles
27 Likes
Answer
We know that in a cyclic quadrilateral the sum of opposite interior angles = 180°.
In cyclic quadrilateral BCDE,
⇒ ∠BCD + ∠BED = 180°
⇒ 140° + ∠BED = 180°
⇒ ∠BED = 180° - 140° = 40°.
∠AEB = 90°. (∵ angle in semicircle = 90°.)
From figure,
∠AED = ∠AEB + ∠BED = 90° + 40° = 130°.
In cyclic quadrilateral AEDB,
⇒ ∠AED + ∠DBA = 180°
⇒ 130° + ∠DBA = 180°
⇒ ∠DBA = 180° - 130° = 50°.
Given chord AE = ED
∴ ∠DBE = ∠EBA
From figure,
⇒ ∠DBA = ∠DBE + ∠EBA
⇒ 50 = ∠DBE + ∠DBE
⇒ 2∠DBE = 50°
⇒ ∠DBE = = 25°.
or, ∠EBD = 25°.
In △OEB, OE = OB (Radii of the same circle.)
∠OEB = ∠EBO = ∠DBE
But these are alternate angles.
∴ OE || BD.
Hence, the value of ∠AED = 130° and ∠EBD = 25°.
Answered By
15 Likes
Related Questions
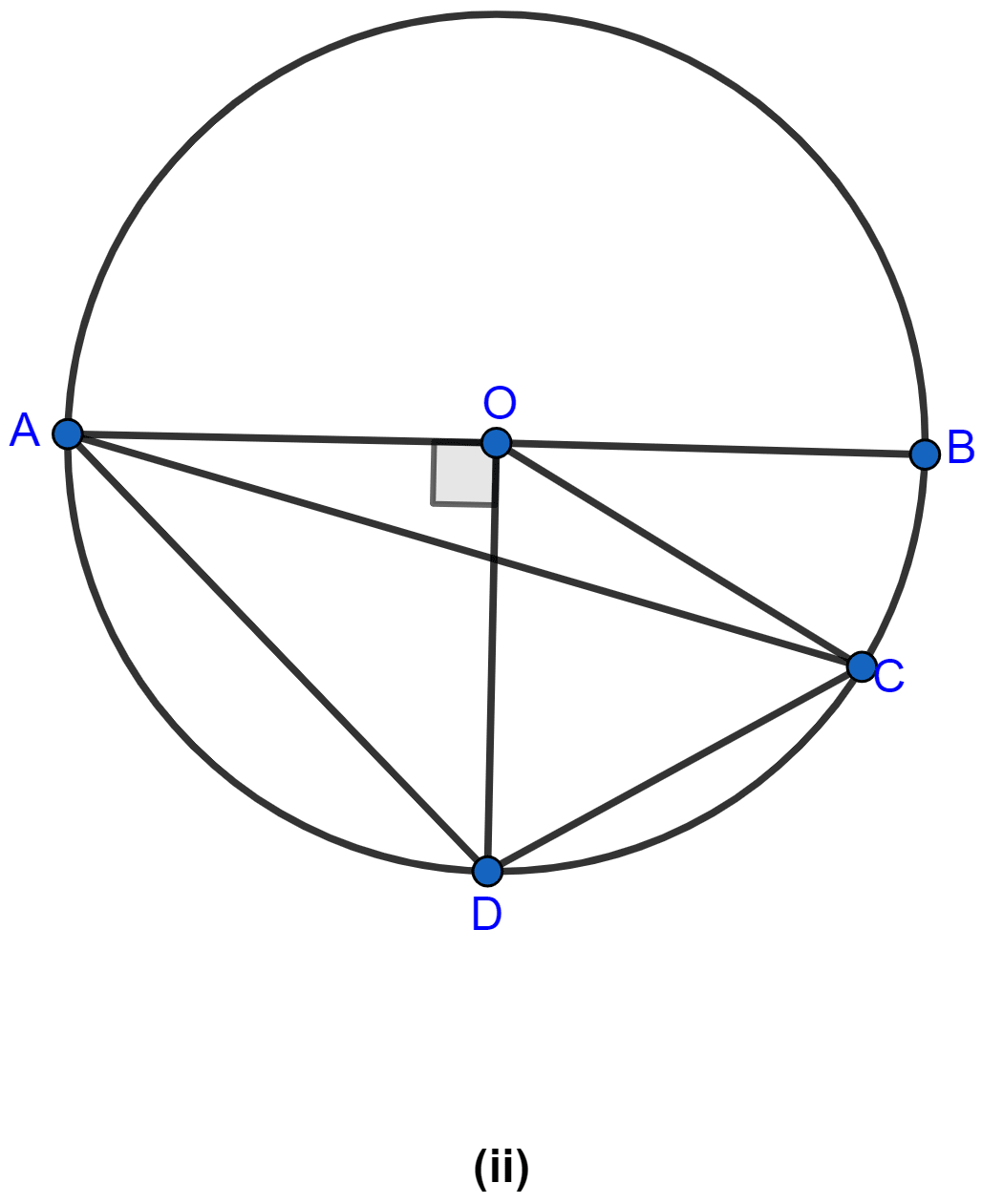
In the figure (ii) given below, AB is a diameter of a circle with centre O. OD is perpendicular to AB and C is a point on the arc DB. Find ∠BAD and ∠ACD.
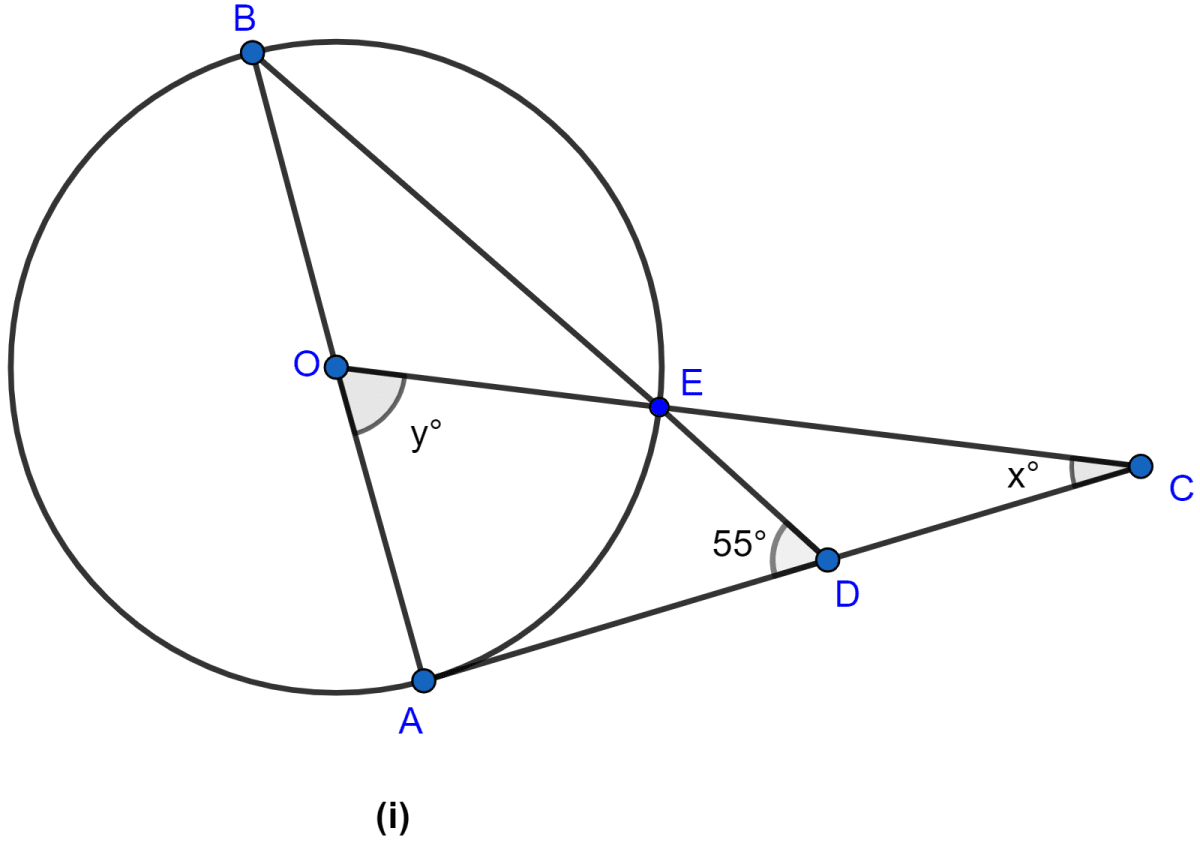
In the figure (i) given below, AC is a tangent to the circle with centre O. If ∠ADB = 55°, find x and y. Give reasons for your answers.
In the figure (i) given below, O is the centre of the circle. Prove that ∠AOC = 2(∠ACB + ∠BAC).
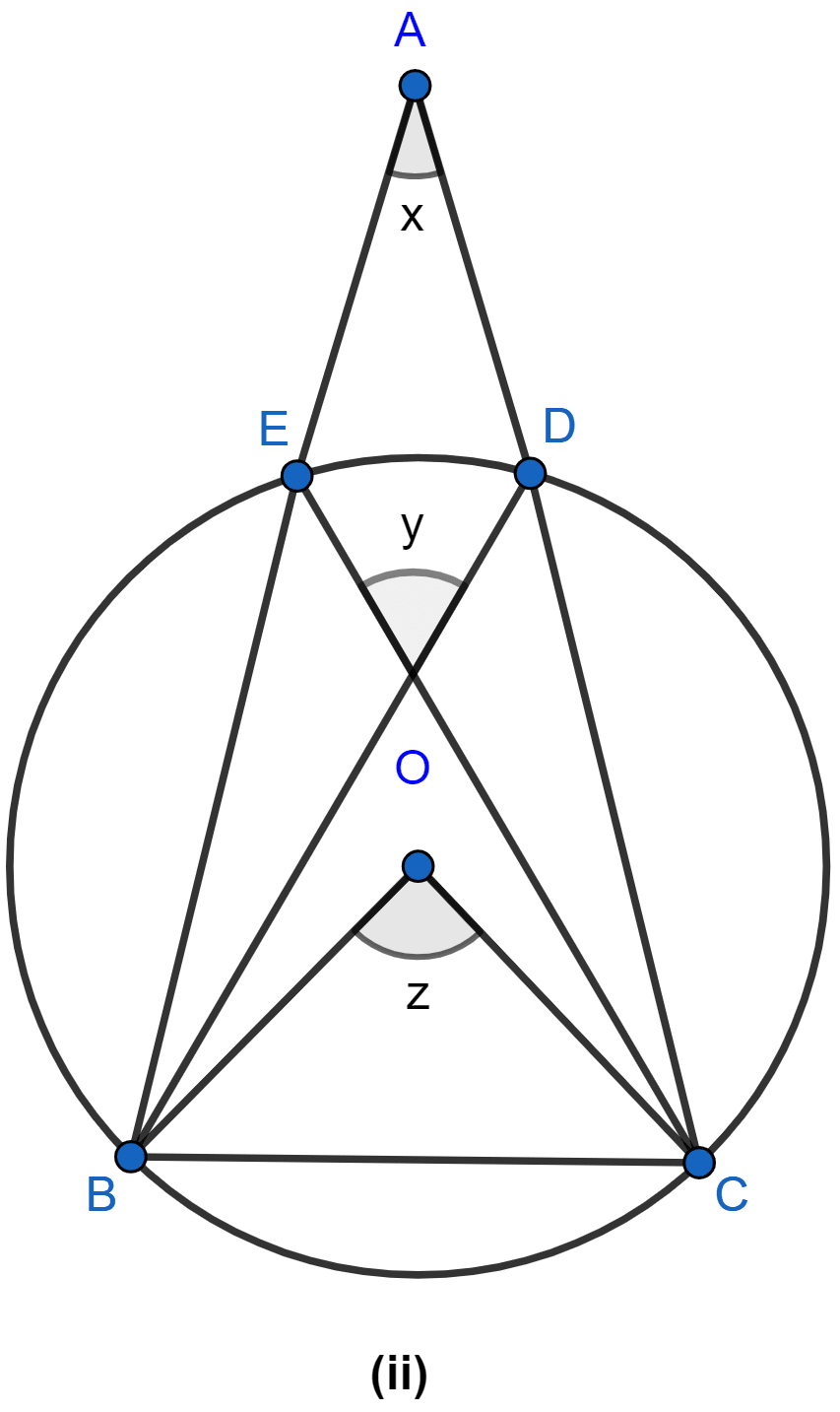
In the figure (ii) given below, O is the centre of the circle. Prove that x + y = z.