Mathematics
In the figure (ii) given below, A, B and C are three points on a circle. The tangent at C meets BA produced at T. Given that ∠ATC = 36° and ∠ACT = 48°, calculate the angle subtended by AB at the centre of the circle.
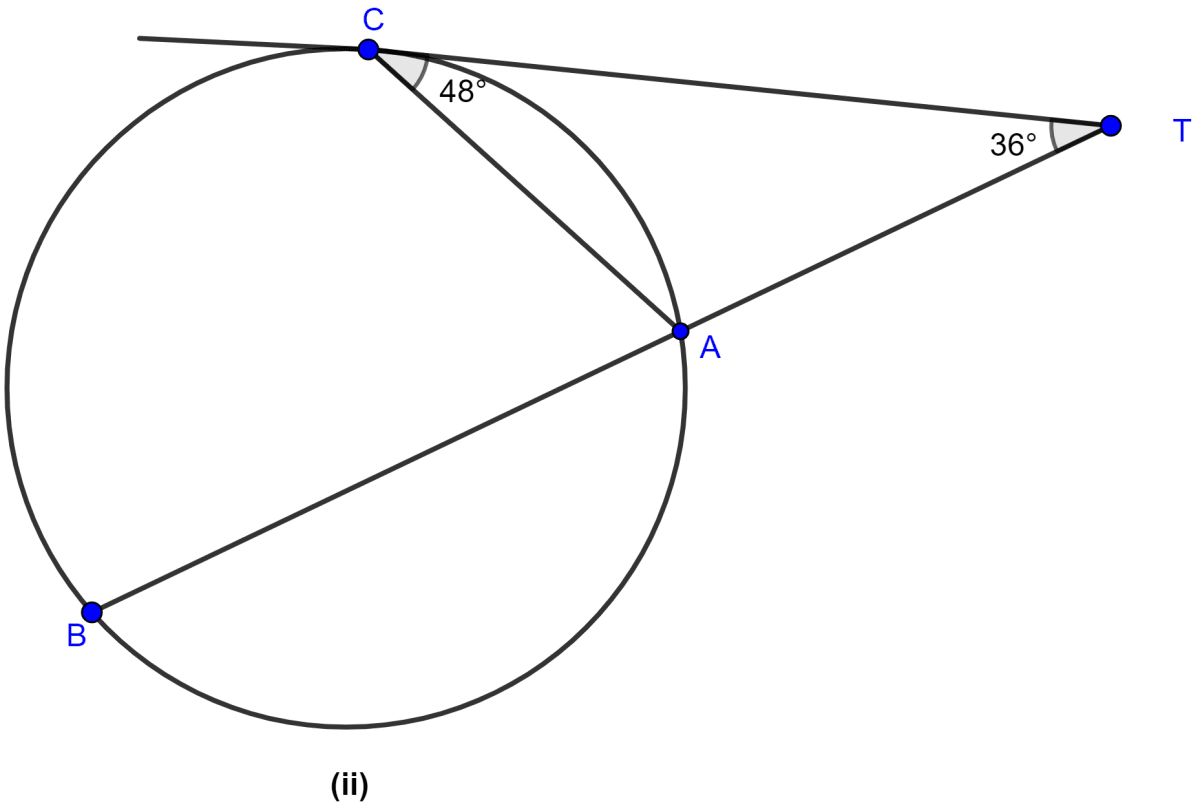
Circles
24 Likes
Answer
Join OA, OB and CB. In △ATC,
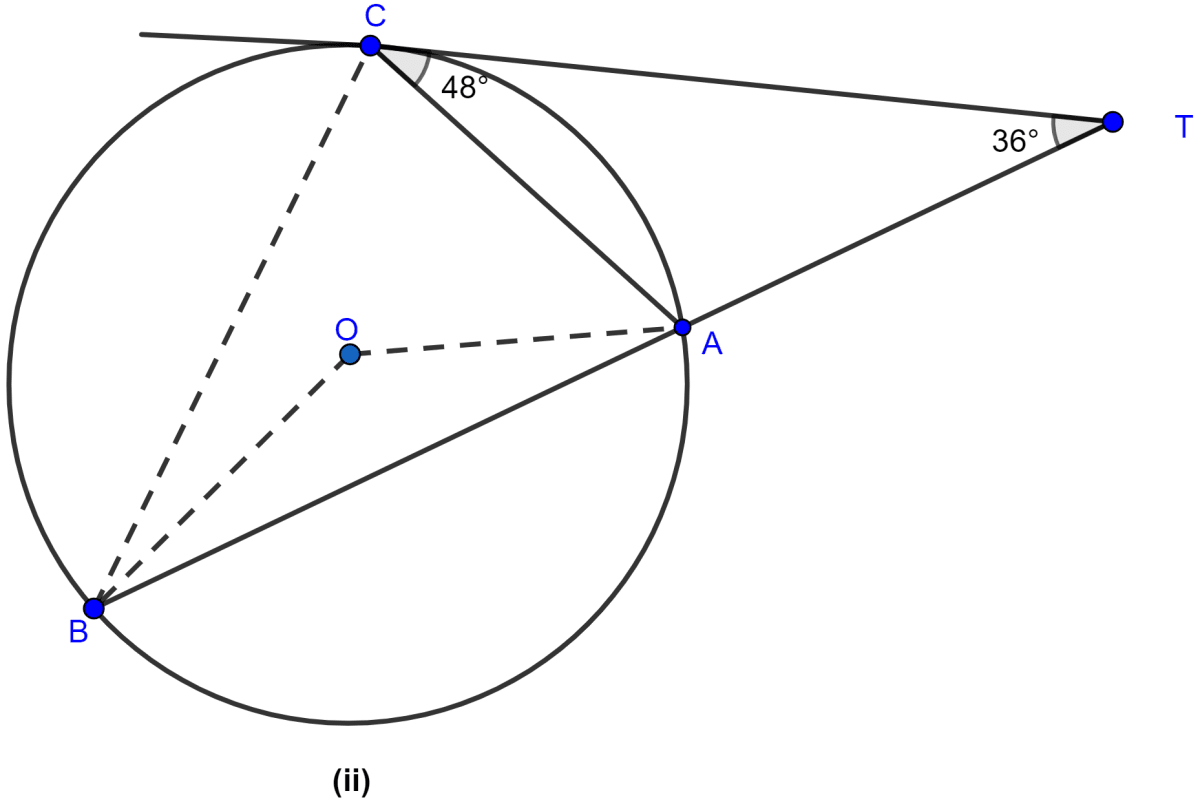
Ext. ∠CAB = ∠ATC + TCA (∵ external angle in a triangle is equal to the sum of opposite interior angles.)
Ext. ∠CAB = 36° + 48° = 84°.
From figure,
∠ABC = ∠TCA = 48° (∵ angles in alternate segment are equal.)
Since sum of angles in a triangle = 180°.
In △ABC,
⇒ ∠ABC + ∠BAC + ∠ACB = 180°
⇒ 48° + 84° + ∠ACB = 180°
⇒ 132° + ∠ACB = 180°
⇒ ∠ACB = 180° - 132°
⇒ ∠ACB = 48°.
Arc AB subtends ∠AOB at the centre and ∠ACB at the remaining part of the circle.
∴ ∠AOB = 2∠ACB (∵ angle subtended at centre is double the angle subtended at remaining part of the circle.)
∠AOB = 2 × 48° = 96°.
Hence, the angle subtended by AB at the center of the circle is 96°.
Answered By
12 Likes
Related Questions
Two chords AB, CD of a circle intersect externally at a point P. If PA = PC, prove that AB = CD.
In the figure (i) given below, AT is tangent to a circle at A. If ∠BAT = 45° and ∠BAC = 65°, find ∠ABC.

In the adjoining figure, △ABC is isosceles with AB = AC. Prove that the tangent at A to the circumcircle of △ABC is parallel to BC.

If the sides of a rectangle touch a circle, prove that the rectangle is a square.