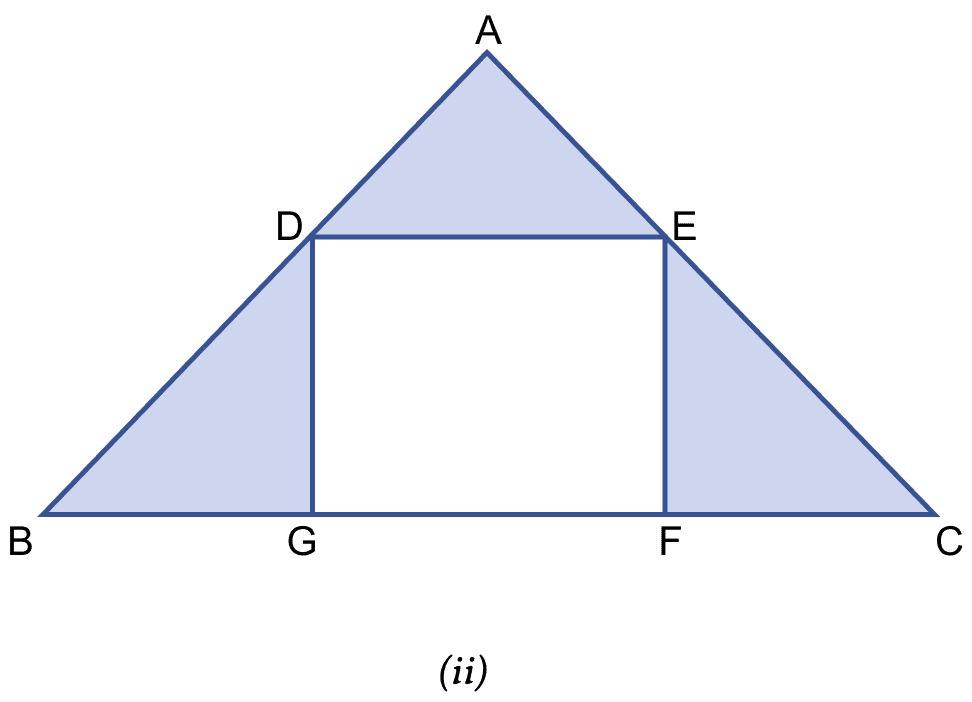
In the figure (ii) given, ABC is an isosceles right-angled triangle and DEFG is a rectangle. If AD = AE = 3 cm and DB = EC = 4 cm, find the area of the shaded region.

From figure,

In right angle triangle ADE,

Using pythagoras theorem,

⇒ DE² = AD² + AE²

⇒ DE² = 3² + 3²

⇒ DE² = 9 + 9

⇒ DE² = 18

⇒ DE = $\sqrt{18} = 3\sqrt{2}$ cm

Since, DEFG is a rectangle.

∴ GF = DE = $3\sqrt{2}$ cm.

In △DBG and △ECF,

DB = EC = 4 cm

DG = EF (Opposite sides of rectangle are equal)

∠DGB = ∠EFC = 90° (∵ DEFG is a rectangle)

Hence, by RHS axiom △DBG ≅ △ECF.

So, BG = FC (By C.P.C.T.)

Let BG = FC = x.

In right angle triangle ABC,

⇒ BC² = AB² + AC²

⇒ BC² = 7² + 7²

⇒ BC² = 49 + 49

⇒ BC² = 98

⇒ BC = $\sqrt{98} = 7\sqrt{2}$ cm

From figure,

BG + GF + FC = BC

⇒ BG + GF + FC = $7\sqrt{2}$

⇒ x + $3\sqrt{2}$ + x = $7\sqrt{2}$

⇒ 2x = $7\sqrt{2} - 3\sqrt{2}$

⇒ 2x = $4\sqrt{2}$

⇒ x = $2\sqrt{2}$ cm.

In right angle triangle DBG,

⇒ DB² = BG² + DG²

⇒ 4² = $(2\sqrt{2})$² + DG²

⇒ 16 = 8 + DG²

⇒ DG² = 16 - 8 = 8

⇒ DG = $\sqrt{8} = 2\sqrt{2}$ cm.

Area of right angle triangle DBG = $\dfrac{1}{2}$ x BG x DG

= $\dfrac{1}{2}$ x $2\sqrt{2}$ x $2\sqrt{2}$

= $\dfrac{1}{2}$ x 8 = 4 cm².

Since, △DBG ≅ △ECF.

∴ Areas of both triangle are equal.

Area of right angle triangle ADE = $\dfrac{1}{2}$ x AD x AE

= $\dfrac{1}{2}$ x 3 x 3

= $\dfrac{9}{2}$ = 4.5 cm².

Area of shaded region = Area of (△ADE + △DBG + △ECF)

= 4.5 + 4 + 4 = 12.5 cm².

Hence, area of shaded region = 12.5 cm².